-
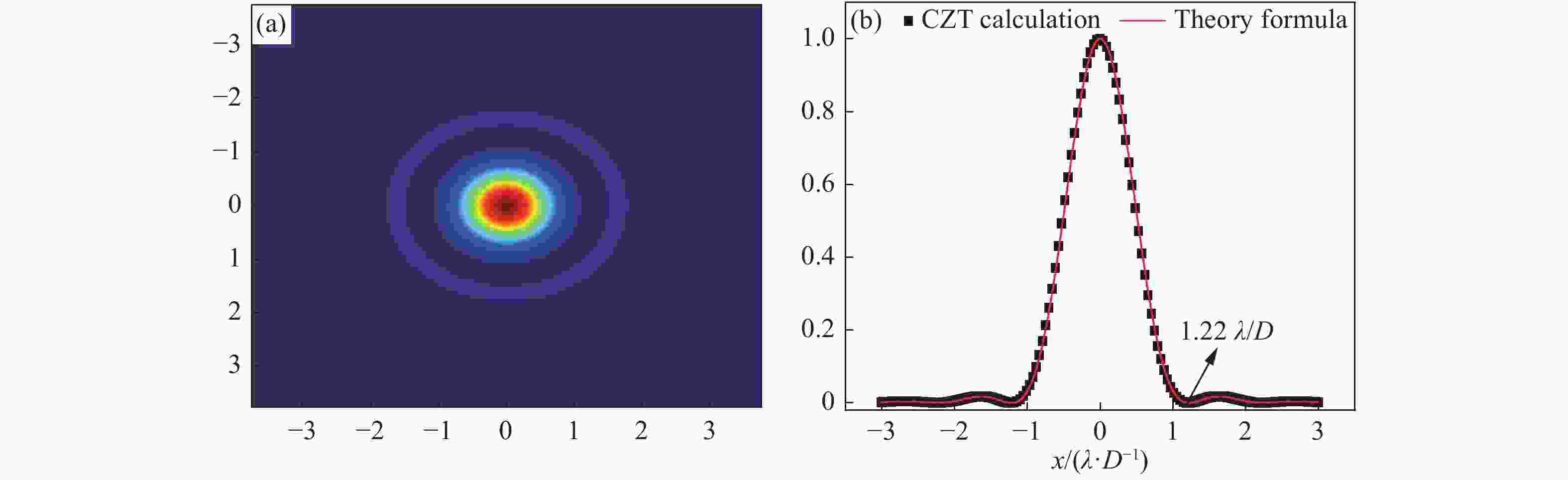
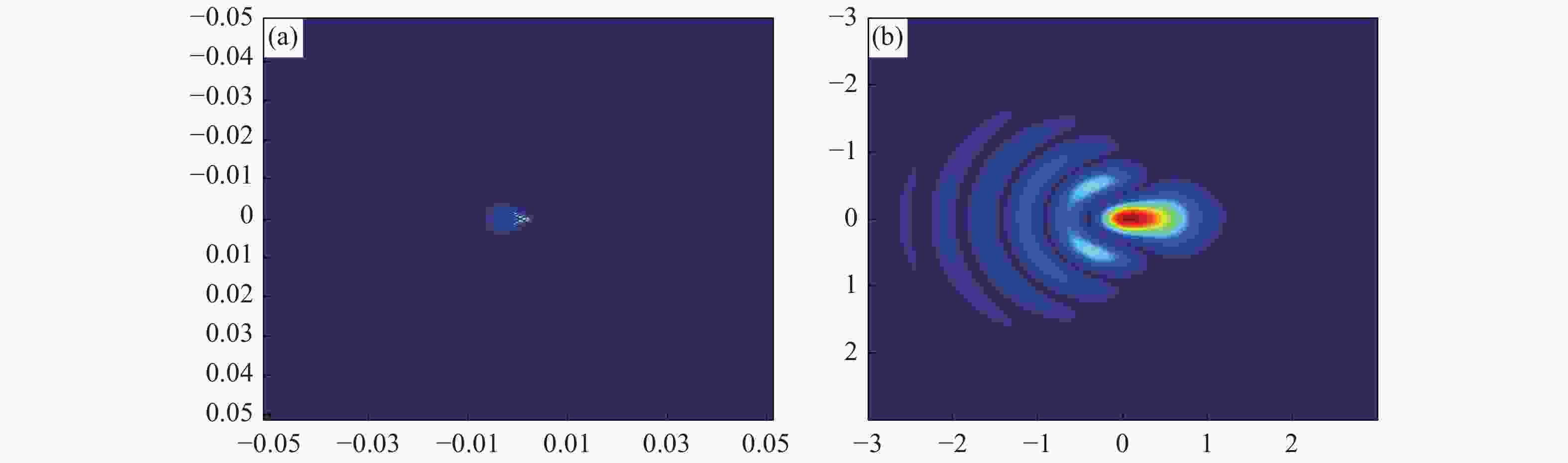
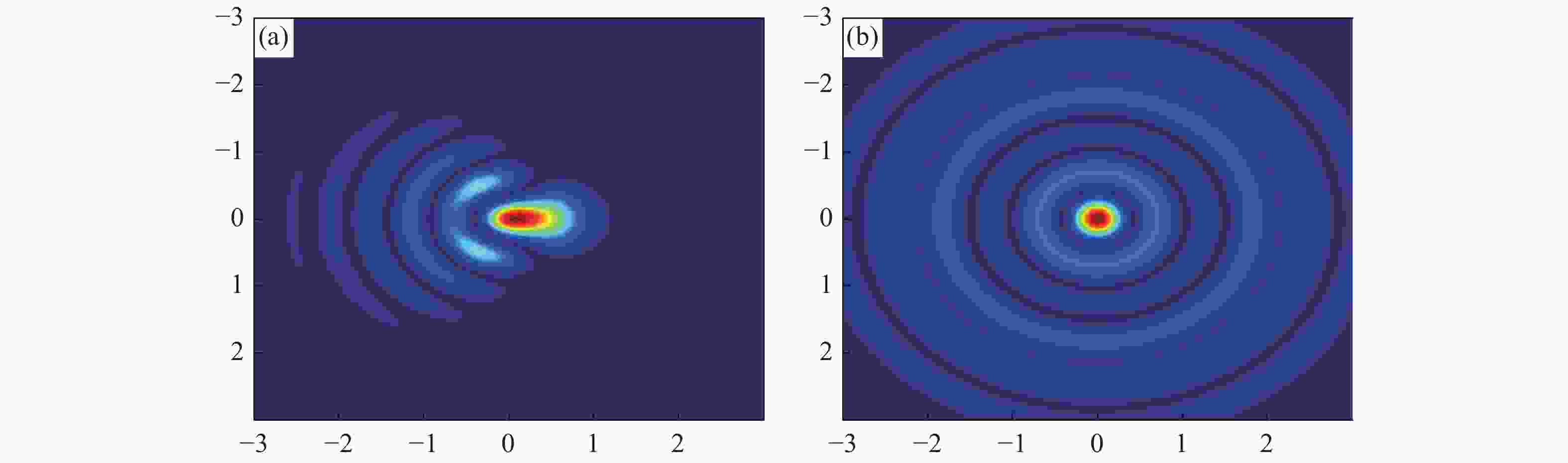
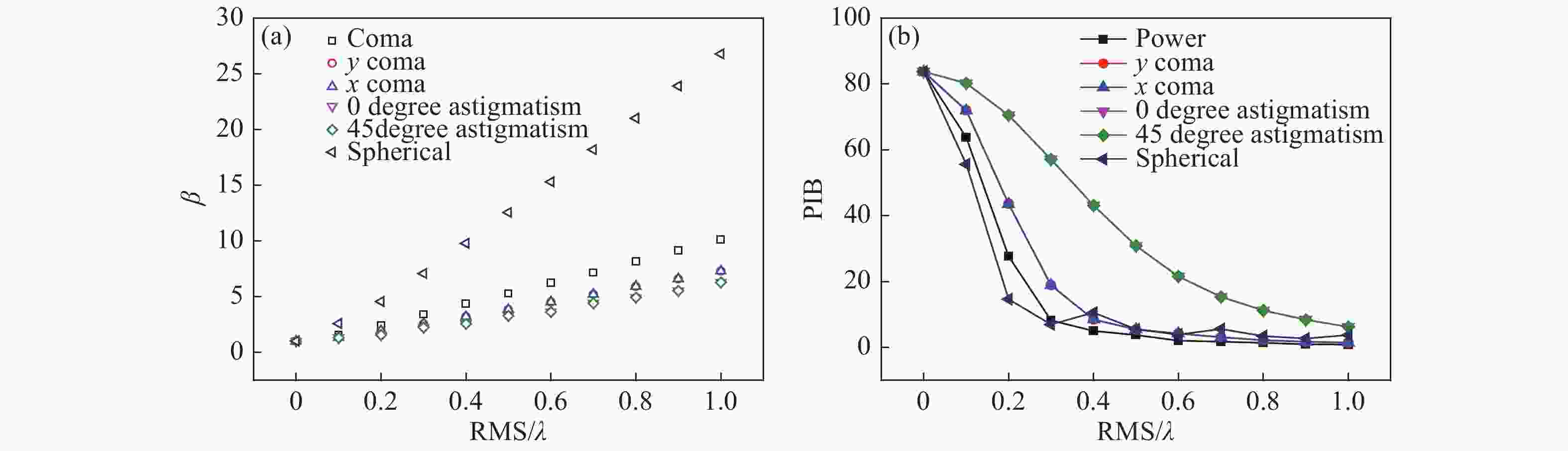
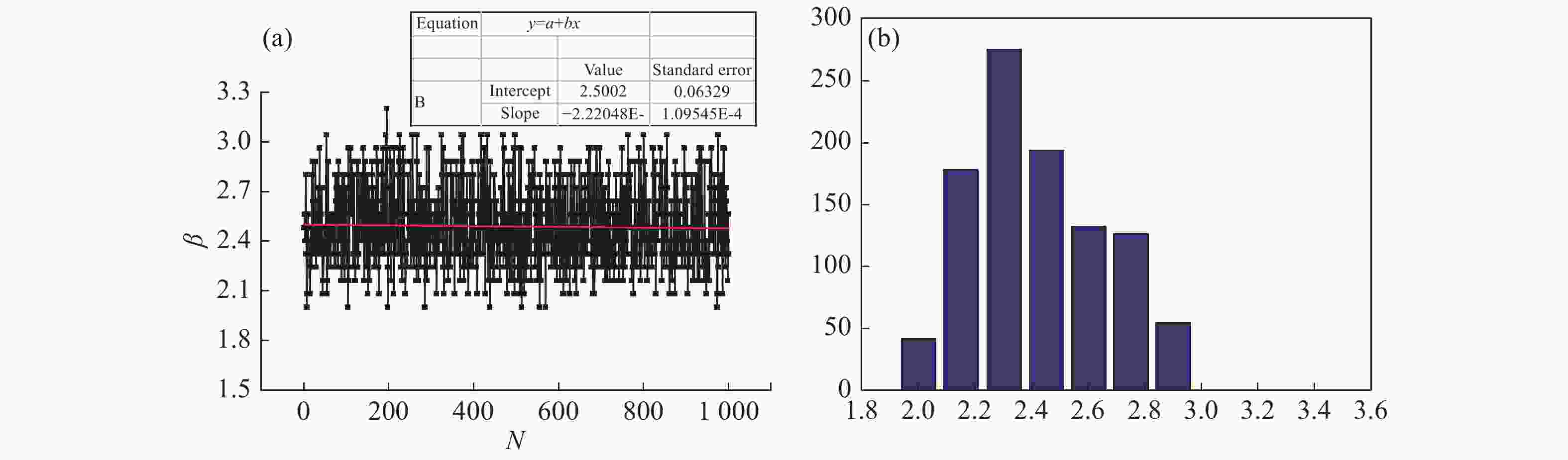
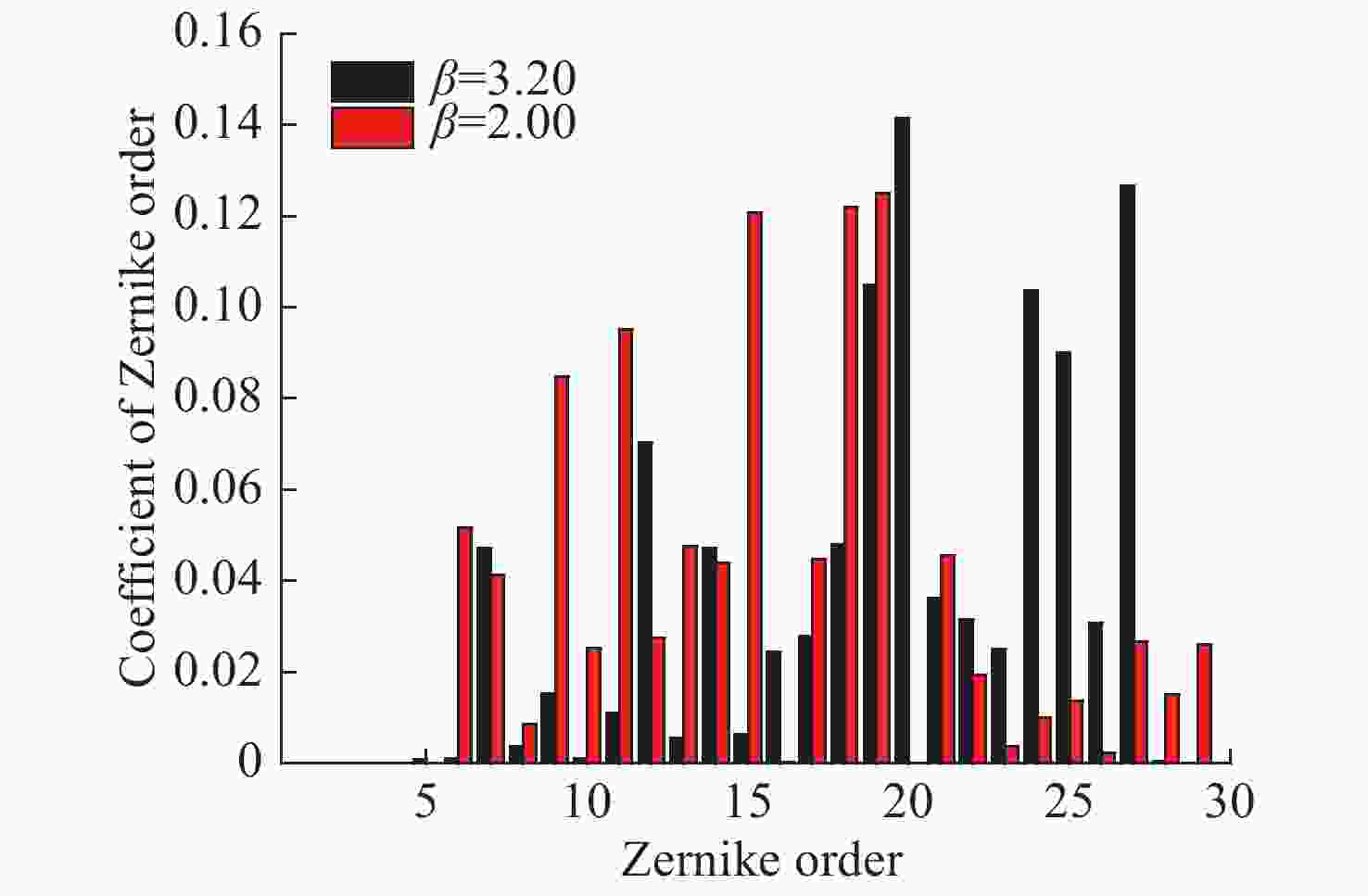
摘要: 本文提出了一种基于二维线性调频Z变换的衍射光场分布快速计算方法,该方法在不增加运算量的情况下可以显著提高光场分布的图像分辨率,进而能够得到更准确的光束质量β因子值。在算法正确性验证的基础上,本文数值模拟了不同光束波前畸变的均方根RMS值与光束质量β因子的对应关系。仿真结果表明,在像差分布RMS值相同的前提下,几种低阶像差类型中球差类型的像差对光束质量的影响最大。为了模拟不同光斑分布形态,随机Zernike像差组合方式的光束质量β因子的仿真计算结果表明:相同的RMS值情况下,高阶像差占比较高的像差组合方式对应的光束质量β因子较大。Abstract: An algorithm for fast calculation of the field distribution of diffraction based on two-dimension chirp Z transformation is proposed. The proposed algorithm does not increase computation and significantly improves the resolution of the diffraction distribution and obtains a more accurate beam quality β factor. After verifying the correctness of the proposed algorithm, the corresponding relationship between the RMS (Root-Mean-Square) of the beam′s wavefront aberration and β factor is simulated. The simulation results show that with the same RMS value, the effect of a spherical aberration on the β factor is the strongest among the lower order Zernike aberrations. In order to simulate the different distribution of beam spots, the β factors are calculated based on different random Zernike wavefront aberrations. The results indicate that a larger proportion of high-order Zernike aberrations in the cumulative aberrations induces a bigger β factor with an identical RMS value.
-
Key words:
- numerical simulation /
- wavefront aberration /
- beam quality /
- chirp Z transformation
-
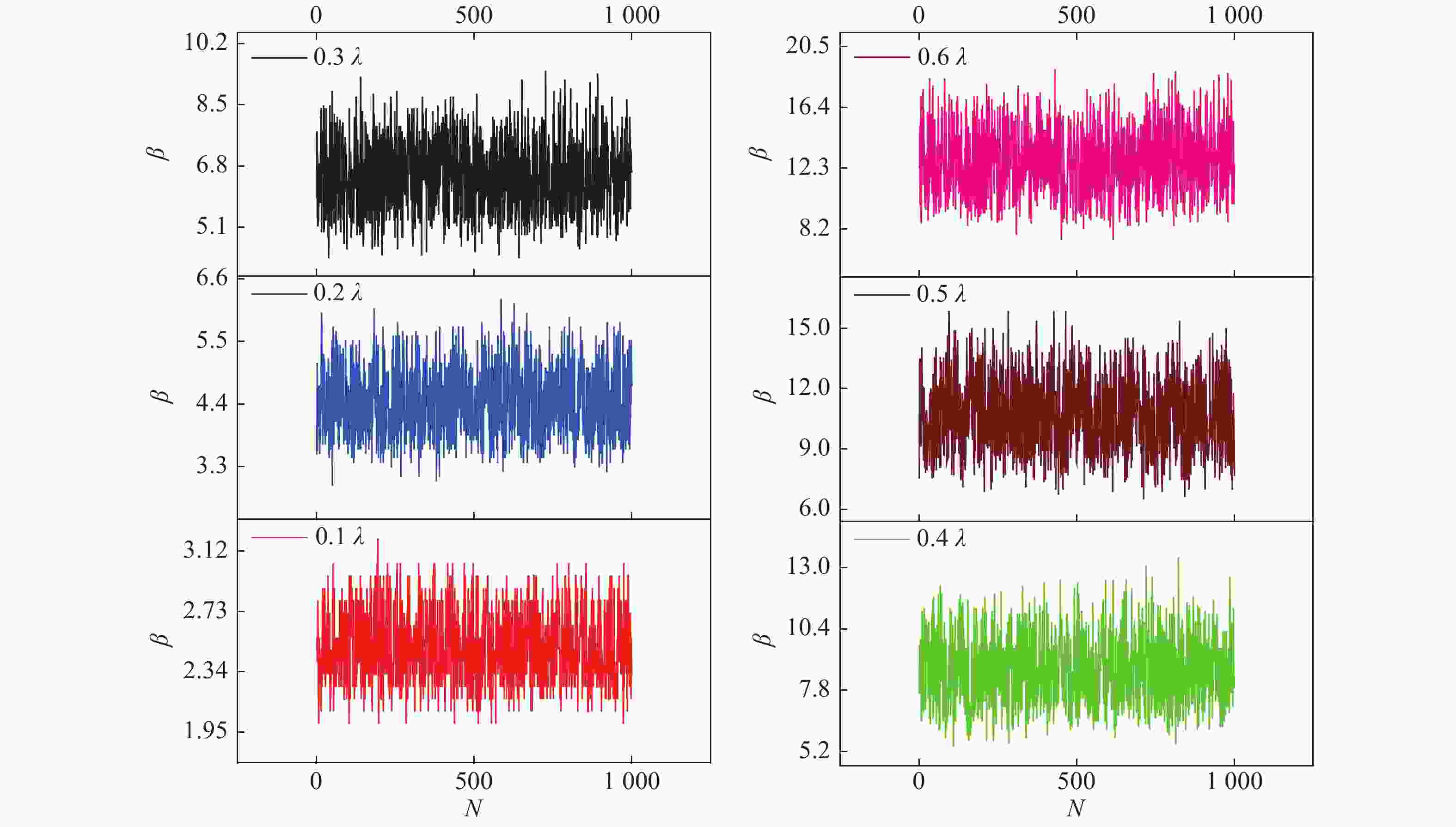
表 1 光束质量β因子统计结果
Table 1. Statistical results of the β factor
波像差RMS值 β因子统计均值 β因子标准偏差 0.1λ 2.50 0.06 0.2λ 4.42 0.04 0.3λ 6.56 0.07 0.4λ 8.66 0.10 0.5λ 10.81 0.13 0.6λ 12.64 0.16 0.7λ 14.87 0.18 0.8λ 17.11 0.21 0.9λ 18.67 0.22 1.0λ 20.90 0.24 -
吕百达, 康小平. 对金宝搏188软件怎么用 光束质量一些问题的认识[J]. 红外与金宝搏188软件怎么用 工程,2007,36(1):47-51. doi: 10.3969/j.issn.1007-2276.2007.01.012LÜ B D, KANG X P. Some aspects of laser beam quality[J]. Infrared and Laser Engineering, 2007, 36(1): 47-51. (in Chinese) doi: 10.3969/j.issn.1007-2276.2007.01.012 田英华, 叶一东, 向汝建, 等. 光束质量因子测量的不确定度分析[J]. 强金宝搏188软件怎么用 与粒子束,2008,20(7):1076-1078.TIAN Y H, YE Y D, XIANG R J, et al. Evaluation of uncertainty in beam quality measurement[J]. High Power Laser and Particle Beams, 2008, 20(7): 1076-1078. (in Chinese) 王云萍, 黄建余, 乔广林. 高能金宝搏188软件怎么用 光束质量的评价方法[J]. 光电子·金宝搏188软件怎么用 ,2001,12(10):1029-1033. doi: 10.3321/j.issn:1005-0086.2001.10.012WANG Y P, HUANG J Y, QIAO G L. A method for evaluating high energy laser beam quality[J]. Journal of Optoelectronics·Laser, 2001, 12(10): 1029-1033. (in Chinese) doi: 10.3321/j.issn:1005-0086.2001.10.012 闫宏宇, 高欣, 宋健, 等. 976 nm宽条形高功率半导体金宝搏188软件怎么用 器的光束质量M2评价[J]. 发光学报,2019,40(2):196-203. doi: 10.3788/fgxb20194002.0196YAN H Y, GAO X, SONG J, et al. Evaluation of beam quality M2 for 976 nm wide stripe high power semiconductor laser[J]. Chinese Journal of Luminescence, 2019, 40(2): 196-203. (in Chinese) doi: 10.3788/fgxb20194002.0196 陈诚, 郑加金, 韦玮, 等. 基于CCD测量金宝搏188软件怎么用 光束质量M2[J]. 发光学报,2017,38(5):642-647. doi: 10.3788/fgxb20173805.0642CHEN CH, ZHENG J J, WEI W, et al. Measurement of laser beam quality M2 based on CCD[J]. Chinese Journal of Luminescence, 2017, 38(5): 642-647. (in Chinese) doi: 10.3788/fgxb20173805.0642 许坤, 付林杰, 钟发成, 等. 高光束质量垂直腔面发射金宝搏188软件怎么用 器同相耦合阵列[J]. 发光学报,2018,39(6):844-849. doi: 10.3788/fgxb20183906.0844XU K, FU L J, ZHONG F CH, et al. High beam quality in-phase coupled vertical cavity surface emitting laser array[J]. Chinese Journal of Luminescence, 2018, 39(6): 844-849. (in Chinese) doi: 10.3788/fgxb20183906.0844 鲜浩, 姜文汉. 波像差与光束质量指标的关系[J]. 中国金宝搏188软件怎么用 ,1999,26(5):415-419. doi: 10.3321/j.issn:0258-7025.1999.05.007XIAN H, JIANG W H. The relation between wavefront aberration and the beam quality factor[J]. Chinese Journal of Lasers, 1999, 26(5): 415-419. (in Chinese) doi: 10.3321/j.issn:0258-7025.1999.05.007 李新阳, 鲜浩, 王春鸿, 等. 波像差与光束质量β因子的关系[J]. 中国金宝搏188软件怎么用 ,2005,32(6):798-802. doi: 10.3321/j.issn:0258-7025.2005.06.016LI X Y, XIAN H, WANG CH H, et al. Relationship between beam quality factor β and wavefront error[J]. Chinese Journal of Lasers, 2005, 32(6): 798-802. (in Chinese) doi: 10.3321/j.issn:0258-7025.2005.06.016 李新阳, 姜文汉, 王春红, 等. 湍流大气中哈特曼传感器的模式波前复原误差[J]. 强金宝搏188软件怎么用 与粒子束,2000,12(2):148-154.LI X Y, JIANG W H, WANG CH H, et al. Modal reconstruction error of the hartmann sensor on measuring the atmosphere disturbed wavefront[J]. High Power Laser and Particle Beams, 2000, 12(2): 148-154. (in Chinese) 李新阳, 姜文汉, 王春红, 等. 湍流大气中哈特曼传感器的模式波前复原误差II[J]. 强金宝搏188软件怎么用 与粒子束,2000,12(3):319-323.LI X Y, JIANG W H, WANG CH H, et al. Modal reconstruction error of the hartmann sensor on measuring the atmosphere disturbed wavefront II[J]. High Power Laser &Particle Beams, 2000, 12(3): 319-323. (in Chinese) 李新阳, 姜文汉. 哈特曼传感器对湍流畸变波前的泽尼克模式复原误差[J]. 强金宝搏188软件怎么用 与粒子束,2002,14(2):243-249.LI X Y, JIANG W H. Zernike modal wavefront reconstruction error of Hartmann sensor on measuring the atmosphere disturbed wavefront[J]. High Power Laser and Particle Beams, 2002, 14(2): 243-249. (in Chinese) 刘志强, 宋庆和, 刘超, 等. 基于虚拟光波场的菲涅耳衍射计算及应用研究[J]. 应用光学,2018,39(2):196-199.LIU ZH Q, SONG Q H, LIU CH, et al. Fresnel diffraction calculation with virtual light wave field and its application[J]. Journal of Applied Optics, 2018, 39(2): 196-199. (in Chinese) 胡琪, 王喆, 刘洪顺, 等. 基于单次傅里叶变换的分段衍射算法[J]. 中国光学,2018,11(4):568-575. doi: 10.3788/co.20181104.0568HU Q, WANG ZH, LIU H SH, et al. Step diffraction algorithm based on single fast fourier transform algorithm[J]. Chinese Optics, 2018, 11(4): 568-575. (in Chinese) doi: 10.3788/co.20181104.0568 向红丽, 范琦, 李云, 等. FFT计算菲涅尔衍射相位的跳变与矫正研究[J]. 计算机与现代化,2018(8):35-38, 50. doi: 10.3969/j.issn.1006-2475.2018.08.007XIANG H L, FAN Q, LI Y, et al. Jump and correction of Fresnel diffraction phase[J]. Computer and Modernization, 2018(8): 35-38, 50. (in Chinese) doi: 10.3969/j.issn.1006-2475.2018.08.007 钱克矛, 李川奇. 频谱校正的线性调频Z变换方法[J]. 振动工程学报,2000,13(4):628-632. doi: 10.3969/j.issn.1004-4523.2000.04.019QIAN K M, LI CH Q. New spectrum correction method based on chirp Z transform[J]. Journal of Vibration Engineering, 2000, 13(4): 628-632. (in Chinese) doi: 10.3969/j.issn.1004-4523.2000.04.019 徐建军, 胡光东, 李锦明. 线性调频Z变换在信号频谱分析中的应用[J]. 应用基础与工程科学学报,2009,17(6):966-972. doi: 10.3969/j.issn.1005-0930.2009.06.019XU J J, HU G D, LI J M. Chirp-Z transform and its applications in spectrum analysis[J]. Journal of Basic Science and Engineering, 2009, 17(6): 966-972. (in Chinese) doi: 10.3969/j.issn.1005-0930.2009.06.019 赖俊森, 杨爱英, 孙雨南. 线性调频Z变换在光采样光性能监测中的应用[J]. 北京理工大学学报,2011,31(7):833-837, 854.LAI J S, YANG A Y, SUN Y N. Application of Chirp-Z transform to the optical-sampling optical performance monitoring[J]. Transactions of Beijing Institute of Technology, 2011, 31(7): 833-837, 854. (in Chinese) -





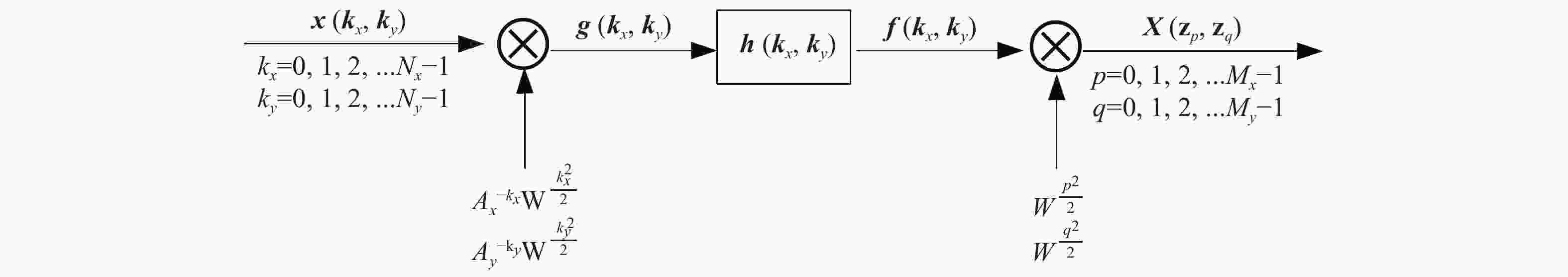
 下载:
下载: