Resolution, super-resolution and spatial bandwidth product expansion——some thoughts from the perspective of computational optical imaging
-
摘要:
传统光学成像实质上是场景强度信号在空间维度上的直接均匀采样记录与再现的过程。在此过程中，成像的分辨率与信息量不可避免地受到光学衍射极限、探测离散器采样、成像系统空间带宽积等若干物理条件制约。如何突破这些物理限制，获得分辨率更高，视场更宽广的图像信息，是该领域的永恒课题。本文概括性地介绍了分辨率、超分辨率与空间带宽积拓展的相关基础理论，核心机理及其在计算光学成像中的若干实例。通过将这些具体个案置入“计算光学成像”这个更高维度的体系框架去分析与探讨，揭示了它们大多数都可以被理解为一种可称作“空间带宽积调控”策略，即利用成像系统的可用自由度，在成像系统有限空间带宽积的限制下，以最佳方式进行编解码和传递信息的过程，或者形象地说——“戴着脚镣跳舞”。这实质上是一种在物理限制下，在“得”与“失”之间所作出的符合规律的权衡与选择。本文的结论有望为设计和探索面向各类复杂现实成像应用的新型成像机理与方法提供有益启示。
Abstract:Conventional optical imaging is essentially a process of recording and reproducing the intensity signal of a scene in the spatial dimension with direct uniform sampling. In this process, the resolution and information content of imaging are inevitably constrained by several physical limitations such as optical diffraction limit, detector sampling, and spatial bandwidth product of the imaging system. How to break these physical limitations and obtain higher resolution and broader image field of view has been an eternal topic in this field. In this paper, we introduce the basic theories and technologies associated with the resolution, super-resolution, and spatial bandwidth product expansion, as well as some examples in the field of computational optical imaging. By placing these specific cases into the higher dimensional framework of "computational optical imaging", this paper reveals that most of them can be understood as a "spatial bandwidth regulation" scheme, i.e., a process of exploiting the available degrees of freedom of the imaging system to optimally encode, decode, and transmit information within the constraints of the limited spatial bandwidth of the imaging system, or figuratively speaking - "dancing with shackles". This is essentially a legal trade-off and choice between "gain" and "loss" under physical constraints. The conclusions of this paper are expected to provide valuable insights into the design and exploration of new imaging mechanisms and methods for various complex practical imaging applications.
-
图 1 艾里斑的典型现象，由其中心的最亮光点和环绕的衍射环组成
Figure 1. The typical phenomenon of an Airy spot consists of the brightest spot at its center and the surrounding diffraction ring
图 2 “瑞利判据”的可视化表达。(a) 成像系统最小可分辨距离（光学角分辨率）与成像系统的孔径成反比。(b-d) 两个非相干的点目标在不同间距下所能拍摄到的艾里斑图像
Figure 2. Visual representation of the "Rayleigh criterion". (a) The minimum resolvable distance (optical angular resolution) of the imaging system is inversely proportional to the aperture of the imaging system. (b-d) Airy spot images of two non-coherent point targets at different spacings
图 3 阿贝成像原理显式将成像过程分为两步：分频与合成
Figure 3. The Abbe imaging principle explicitly divides the imaging process into two steps: frequency division and synthesis
图 4 艾里斑(a)与4个常用的分辨率度量准则（即Rayleigh (b)、 Sparrow (c)、 Abbe (d)和FWHM (e)）。灰色和蓝色的曲线代表试样中不同点的单个强度变化，其中垂直（y-）轴是强度，水平（x-）轴是各点之间的横向间隔。下图上方的曲线描述了所述的对强度分布的单独贡献，而下方的曲线展示了由各自上方曲线中的每个单独成分形成的叠加强度曲线
Figure 4. Airy spot (a) and 4 widely-utilized criterion (i.e., Rayleigh (b), Sparrow (c), Abbe (d), and FWHM (e)) for resolution computation. The gray and blue curves represent the individual intensity variations at different points in a specimen where the vertical (y-) axis is the intensity and the horizontal (x-) axis is the lateral separation between the points. The bottom plots describe the individual contributions to the intensity distribution while the top plots illustrate a super-imposed intensity profile formed by each of the individual components in the respective bottom plots
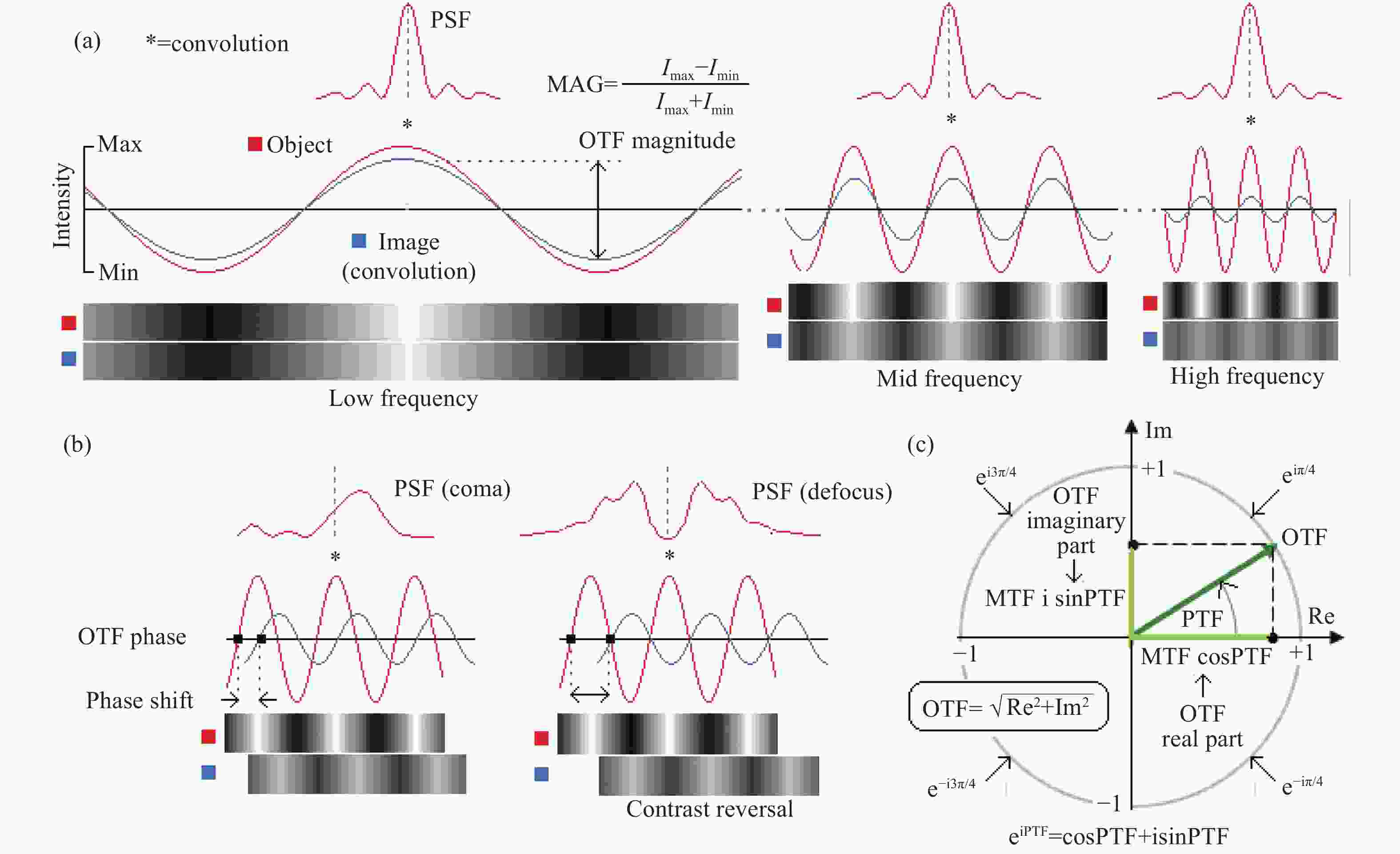
图 5 OTF 的幅值和相位成分。（a）表示 OTF 对强度调制的影响，即对比度的影响；（b）表示OTF对空间分布的影响。（c）OTF的大小完全取决于正弦波模式的最小强度（IMIN）与最大强度（IMAX）的相对大小。为了纳入可能的相移的影响，OTF是在复数坐标的单位圆内构建的，其实部和虚部反映了相移的大小，在这些坐标中，OTF 的实部和虚部的平方根给出，因此保持单位值，与相移无关
Figure 5. The magnitude and phase of the OTF. The former expresses the effect on intensity modulation, i.e., contrast (a), and the latter is the spatial distribution (b). OTF magnitude depends solely on the relative magnitude of the minimum intensity (IMIN) of the sinusoidal pattern vs. its maximum (IMAX). To incorporate the effect of a possible phase shift, OTF is constructed within a unit circle in complex coordinates, with its real and imaginary parts reflecting the magnitude of phase shift, but not the OTF magnitude itself, which is in these coordinates given by a square root of the sum of squared real and imaginary parts of OTF, therefore remaining unit value independent of the phase shift (c)
图 6 相干传递函数和非相干光学传递函数的计算模型和分布剖线
Figure 6. Computational model and distribution profile of coherent transfer function and optical transfer function under incoherence imaging condition
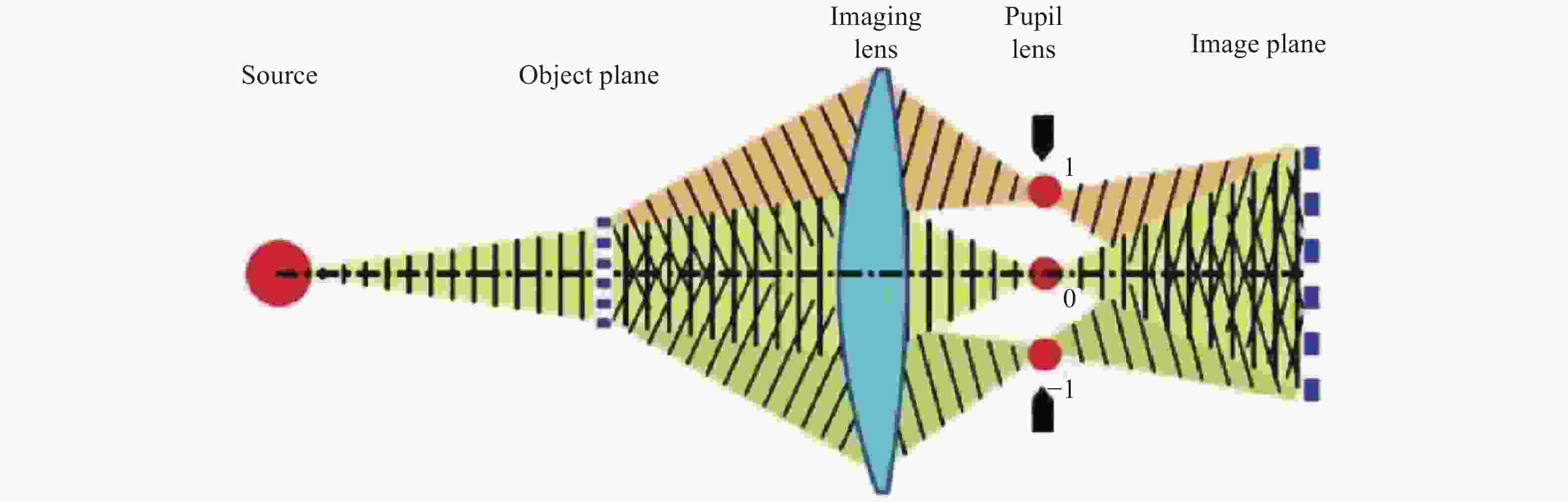
图 7 不同照明条件下的光学传递函数几何示意图。（a）相干与非相干成像情况（光源孔径无穷小或大于等于物镜孔径）；（b）部分相干成像情况（光源孔径小于物镜孔径）
Figure 7. Geometric schematic of the optical transfer function under different illumination conditions. (a) Coherent and incoherent imaging cases (source aperture is infinitely small, or is greater than or equal to the objective aperture); (b) partially coherent imaging case (source aperture is smaller than the objective aperture)
图 8 相干成像下两点源强度与它们相位差之间的关系。竖线表示两个点源的位置。其中ϕ是两个点源之间的相对相位[24]
Figure 8. The relationship between the intensity of two point sources under coherent imaging and their phase difference. The vertical lines indicate the positions of the two point sources, where ϕ is the relative phase between the two point sources[24]
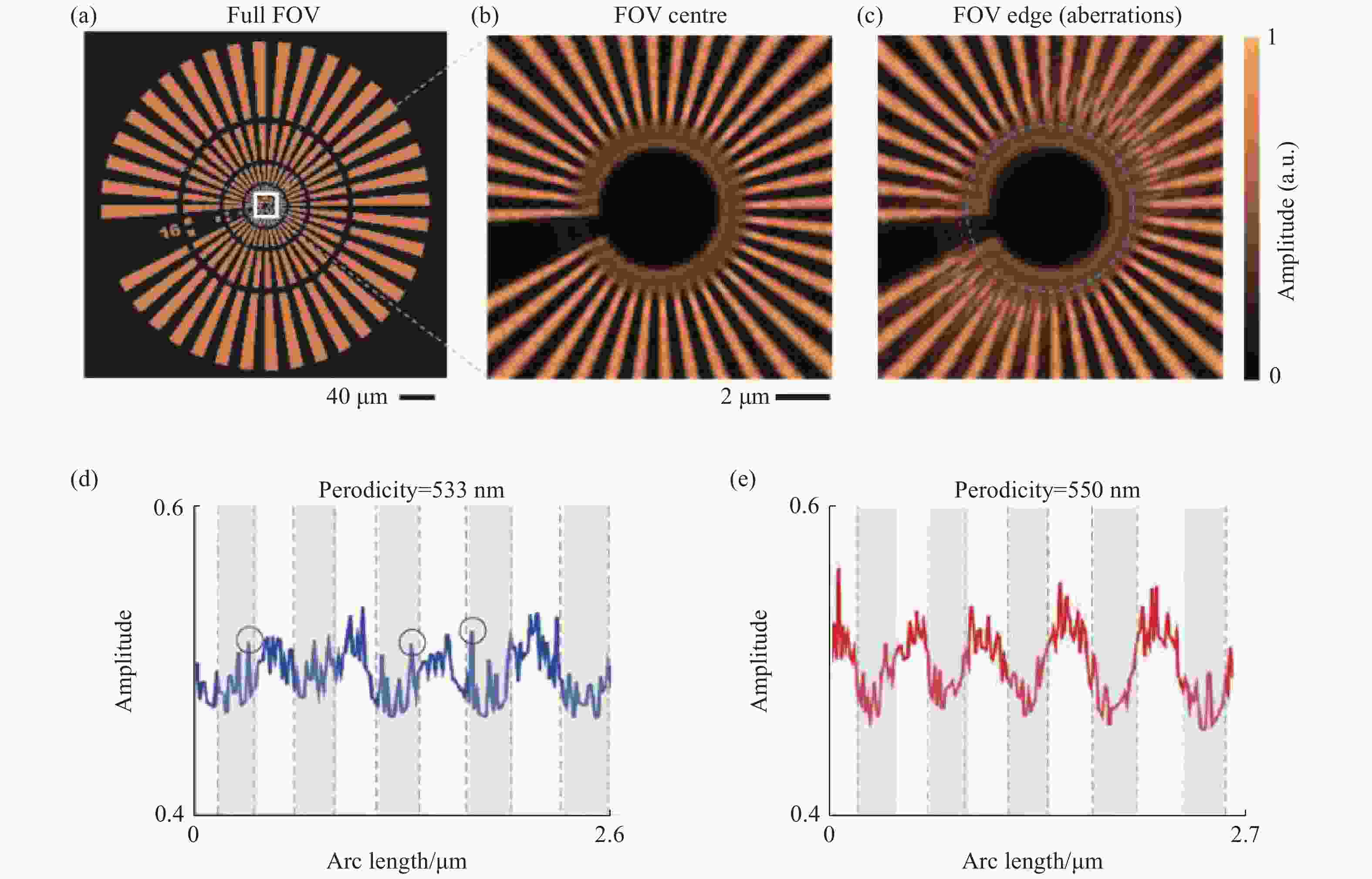
图 9 用西门子星的分辨率靶标衡量相干成像系统的分辨率（波长 400 nm，100× 0.8NA，像素大小1.3 μm，有泊松噪声）[51]。（a）理想目标图像； （b）视场中心成像效果；（c）视场边缘成像效果（存在像差）；（d）沿着（c）中圈出的一段图像振幅值，周期为533 nm。由于“暗”辐条内的噪声值（圈出的）超过了“亮”辐条内的值，因此不可能明确地声称分辨率为533 nm。（e）周期为550 nm的辐条被清晰分辨（所有辐条都通过了验证）
Figure 9. Simulated example of a resolution report with the Siemens star for a coherent imaging system (λ = 0.40 μm, 100× 0.8 numerical aperture objective, pixel size = 1.3 μm, with Poisson noise)[50]. (a) Ideal target image. (b) Imaging effect of region in while box of (a). (c) Re-imaged target center after moving it to the edge of the sensor, where aberrations further limit effective resolution. (d) Plot of amplitude values along a segment of the blue circle in (c) at 533 nm spoke periodicity. As noisy values within ‘dark’ spokes (circled) exceed values within ‘bright’ spokes, it is not possible to unambiguously claim a resolution of 533 nm. (e) Similar plot along the red circle in (c), showing that spokes at a periodicity of 550 nm are unambiguously resolved (verified for all spokes)
图 10 阿贝衍射极限—— 光学成像系统难以看清比波长一半小的物体的细节
Figure 10. Abbe diffraction limit—optical imaging systems have difficulty seeing details of objects smaller than half the wavelength
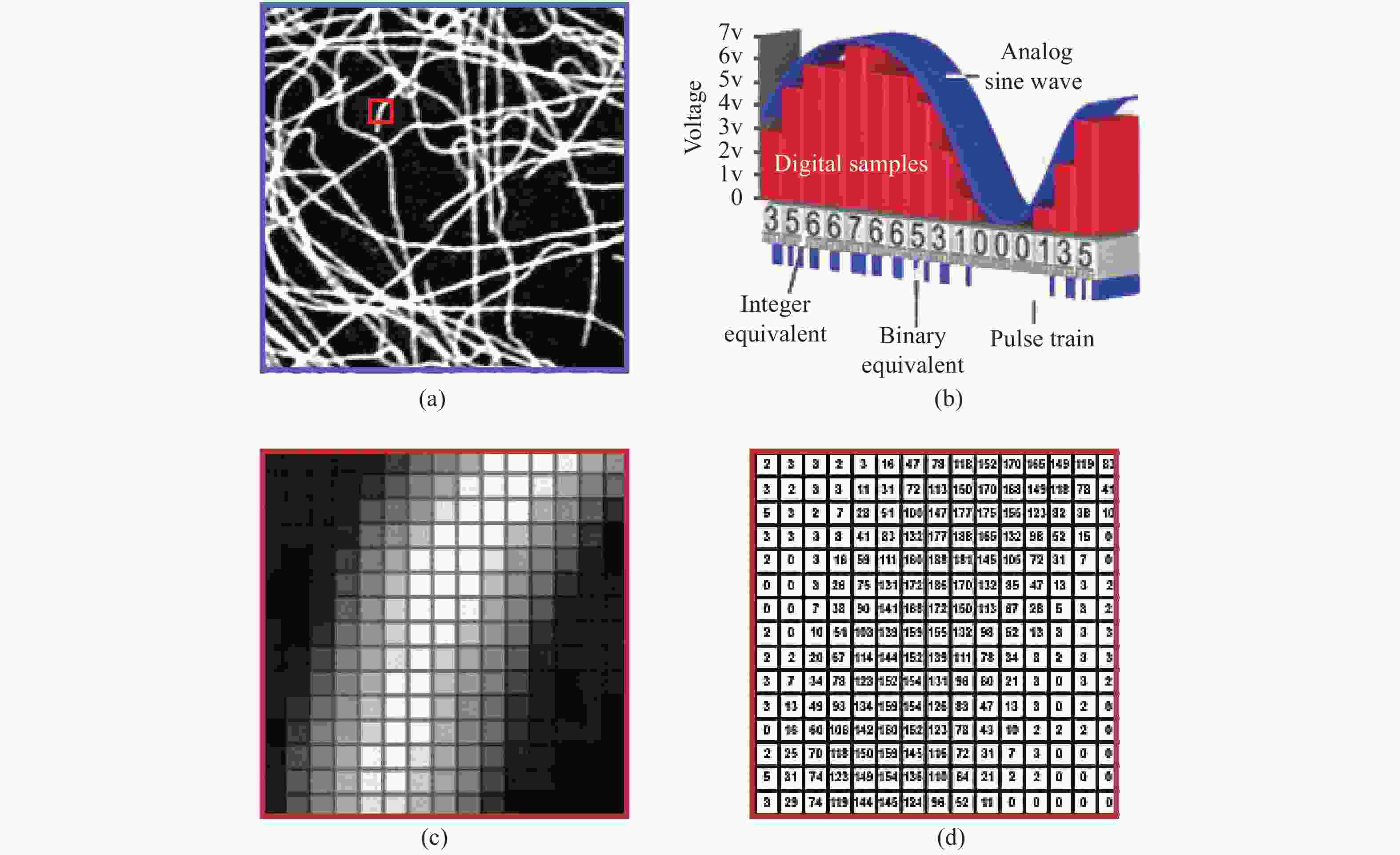
图 11 光学图像的离散化与数字化记录过程。（a）原始理想光学图像；（b）局部区域的离散采样图像；（c）图（a）红框区域的局部放大图；（d）对应区域的像素灰度数值
Figure 11. The process of discretization and digital recording of optical images. (a) Original ideal optical image; (b) local area discrete sampling image; (c) enlarged view of the area in the red box in (a); (d) pixel gray scale of the corresponding area
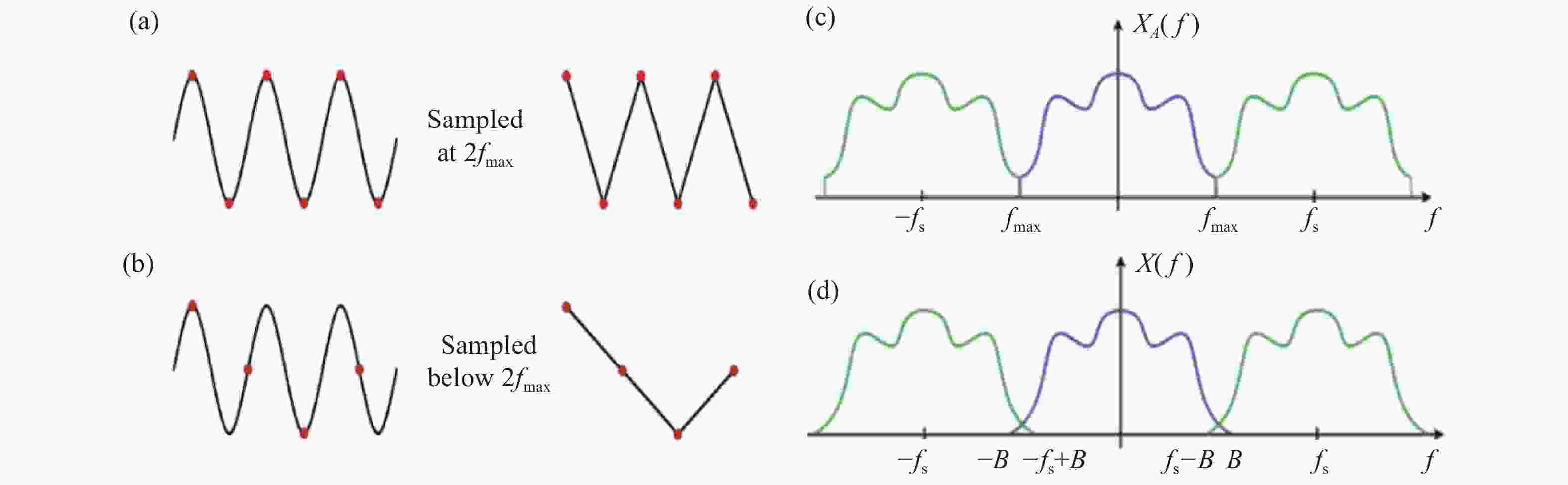
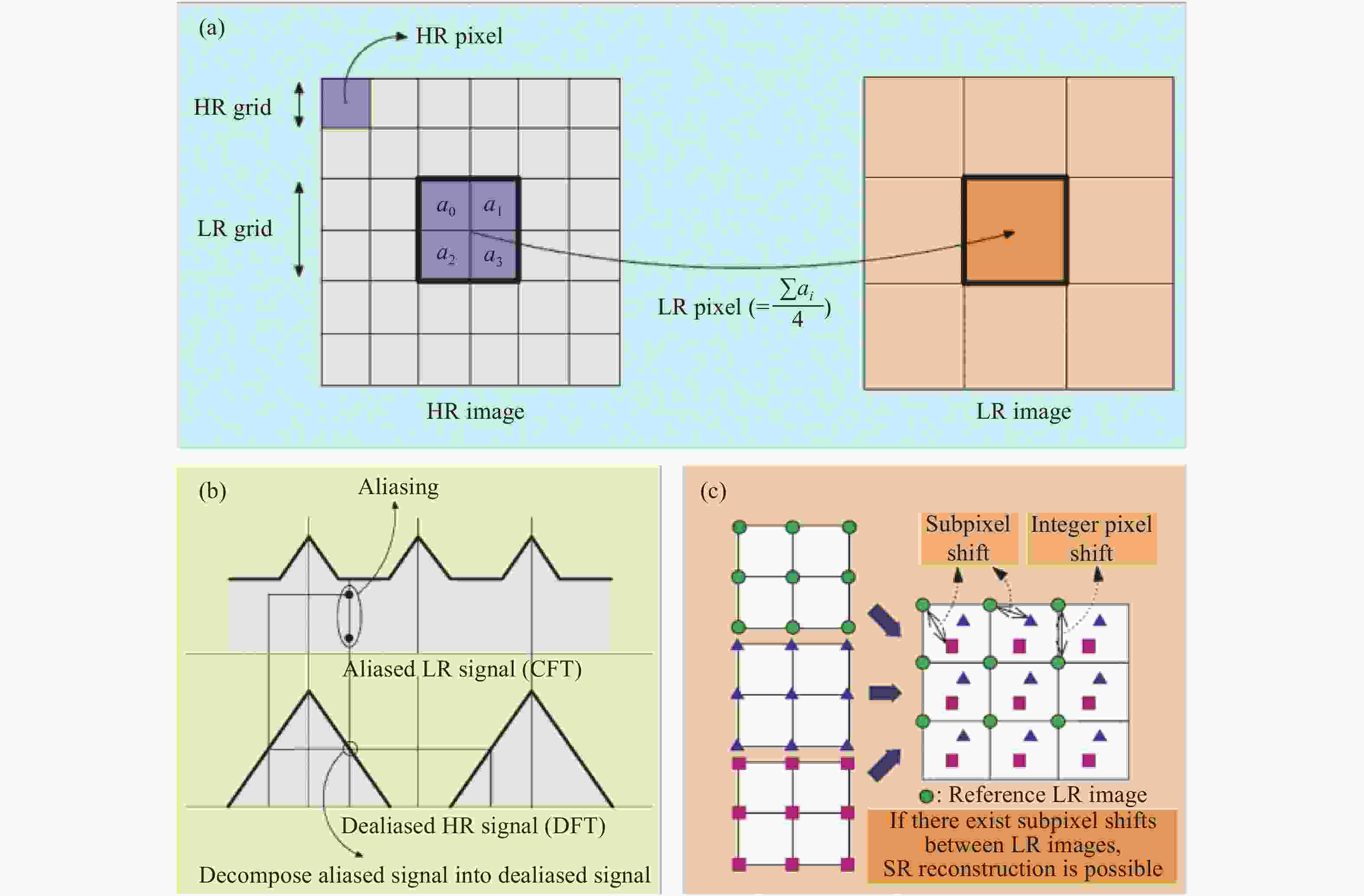
图 12 香农-奈奎斯特采样定理。（a）采样间距恰好满足Nyquist采样频率
$2{f_{\max }}$ 可以采集到信号正确的周期变化；（b）采样间距小于Nyquist采样频率$2{f_{\max }}$ 无法采集到正确的周期信号；（c）满足Nyquist采样频率时，原信号频谱沿频域产生复制但不产生混叠；（d）不满足Nyquist采样频率时信号频谱产生混叠Figure 12. Shannon-Nyquist sampling theorem. (a) The correct periodic variation of the signal can be captured when the sampling spacing exactly satisfies the Nyquist sampling frequency
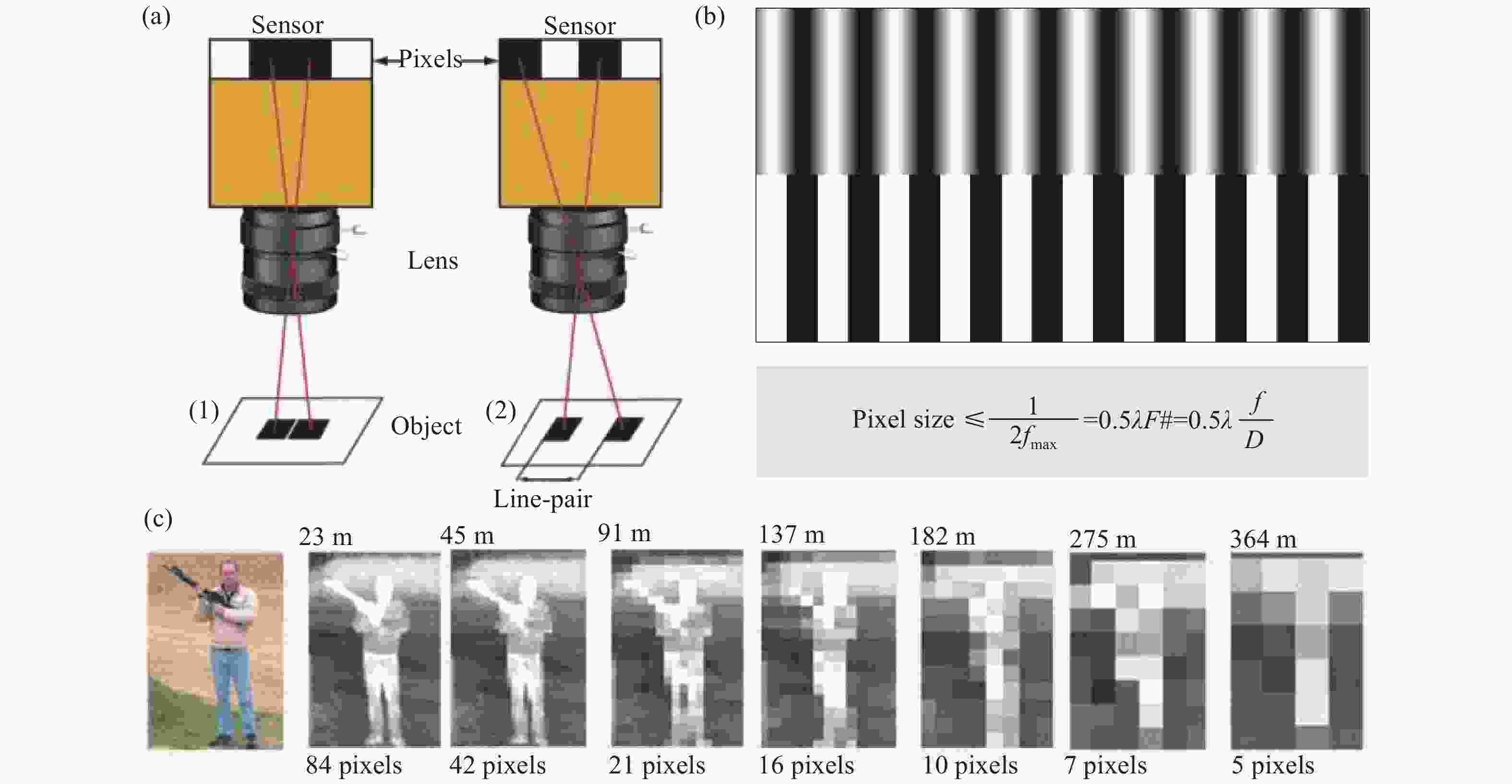
$2{f_{\max }}$ ; (b) the correct periodic signal cannot be captured when the sampling spacing is less than the Nyquist sampling frequency$2{f_{\max }}$ ; (c) when the Nyquist sampling frequency is satisfied, the original signal spectrum is replicated along the frequency domain but no aliasing occurs; (d) the signal spectrum is overlapped when the Nyquist sampling frequency is not satisfied
图 13 探测器像元大小所限制的奈奎斯特采样极限（马赛克效应）。（a）像素采样不足（像素尺寸过大）所导致的信息混叠现象；（b）恰好满足奈奎斯特采样极限时的情况；（c）一个典型的红外热像仪对于人体目标在不同距离下的成像效果（像元尺寸为38 μm，像素为320×240，50 mm焦距镜头）
Figure 13. Nyquist sampling limit (mosaic effect) limited by detector image element size. (a) Information aliasing caused by under-sampling of pixels (too large pixel size); (b) The case when the Nyquist sampling limit is exactly satisfied; (c) imaging results of a typical thermal imaging camera for a human target at different distances (image element size of 38 μm, pixels of 320 × 240, and a lens of 50 mm focal length)
图 14 大多数红外热像仪，如制冷中波热像仪和非制冷长波热像仪的像素尺寸较大。特别是当配备了在宽视场大口径的光学成像系统时，像元尺寸成为了限制其成像分辨率的根本因素（采样比Fλ/d小于2）[56]
Figure 14. The pixel size of most thermal imaging cameras, especially cooled mid-wave cameras and uncooled long-wave cameras, is large, especially when equipped with a large-aperture optical imaging system in a wide field of view, and the image element size becomes a fundamental factor limiting its imaging resolution (sampling ratio Fλ/d less than 2)[56]
图 15 常见信号变换在相空间的表征。（a）菲涅耳传播；（b）Chirp调制（透镜）；（c）傅立叶变换；（d）分数阶傅立叶变换；（e）光束放大器
Figure 15. The characterization of common signal transformations in phase space. (a) Fresnel propagation; (b) Chirp modulation (lens); (c) Fourier transform; (d) Fractional Fourier transform; (e) beam magnifier
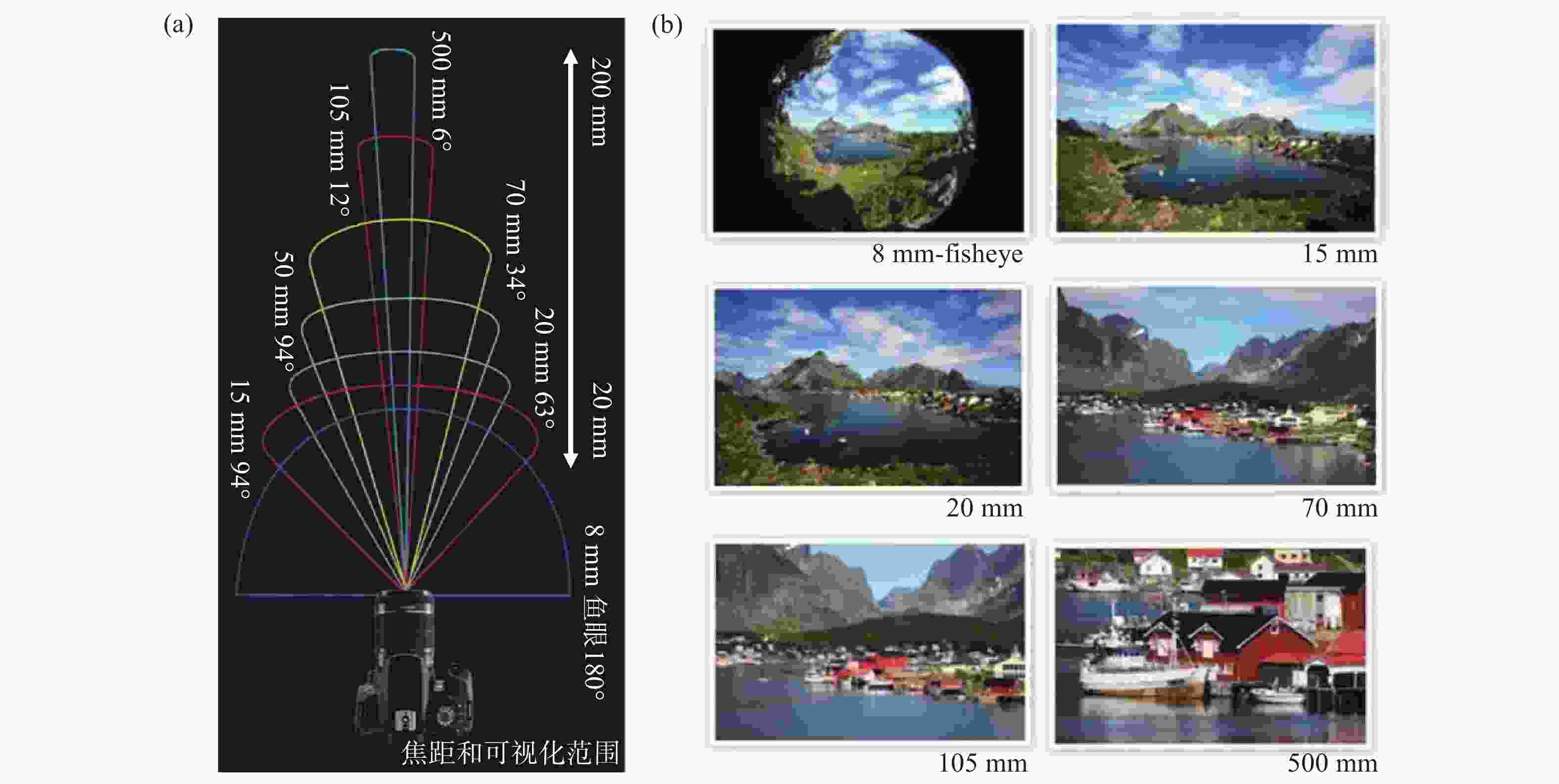
图 16 对于传统光学系统，视场与分辨率这两个参数互相矛盾，无法同时兼顾。（a）35 mm单反相机不同焦距下所对应的视场角；（b）35 mm单反相机不同焦距下所拍摄到的典型图像
Figure 16. For conventional optical systems, the two parameters of the field of view and resolution are contradictory and cannot be accommodated at the same time. (a) Field of view of 35 mm SLR cameras at different focal lengths; (b) typical images captured by 35 mm SLR cameras at different focal lengths
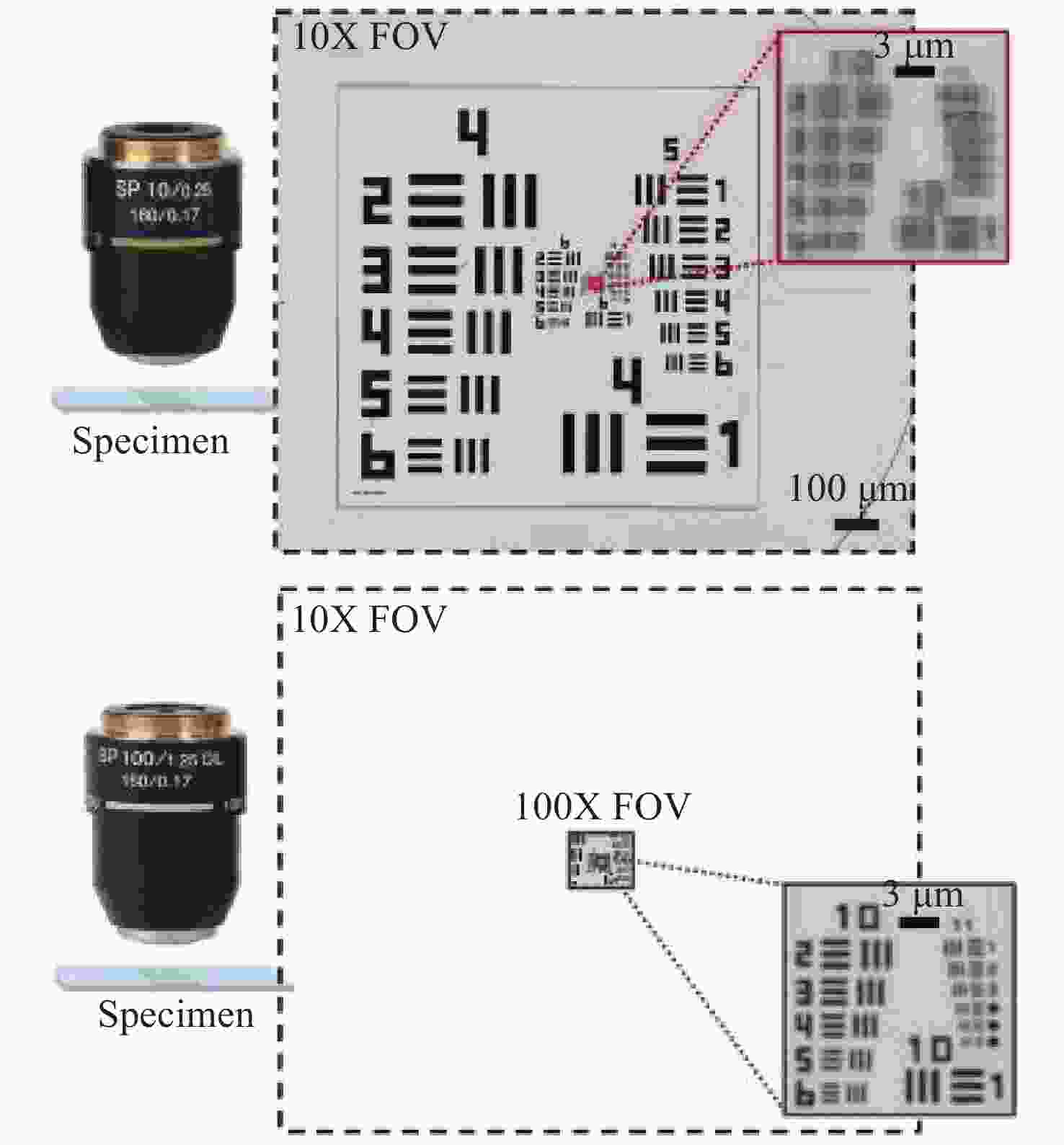
图 17 传统显微镜存在分辨率与视场大小难以同时兼顾的矛盾：低倍镜下视野大，但分辨率低；切换到高倍镜后分辨率虽得以提升，视场却相应的成更高比例的缩减
Figure 17. There is a tradeoff between the resolution and FOV in traditional microscopes: the FOV under low-magnification objective is large with the low resolution; for high-magnification objective, the resolution is improved while the FOV is reduced dramatically
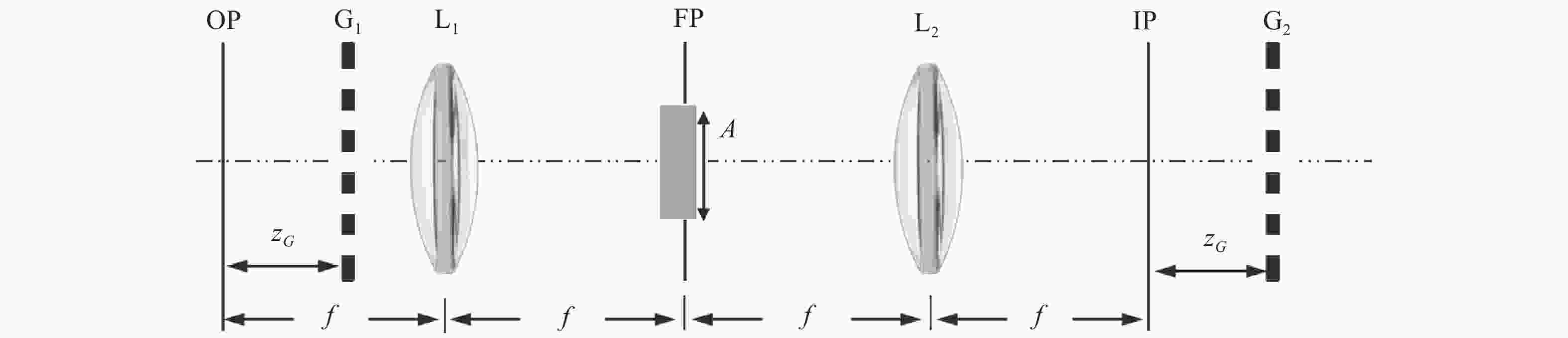
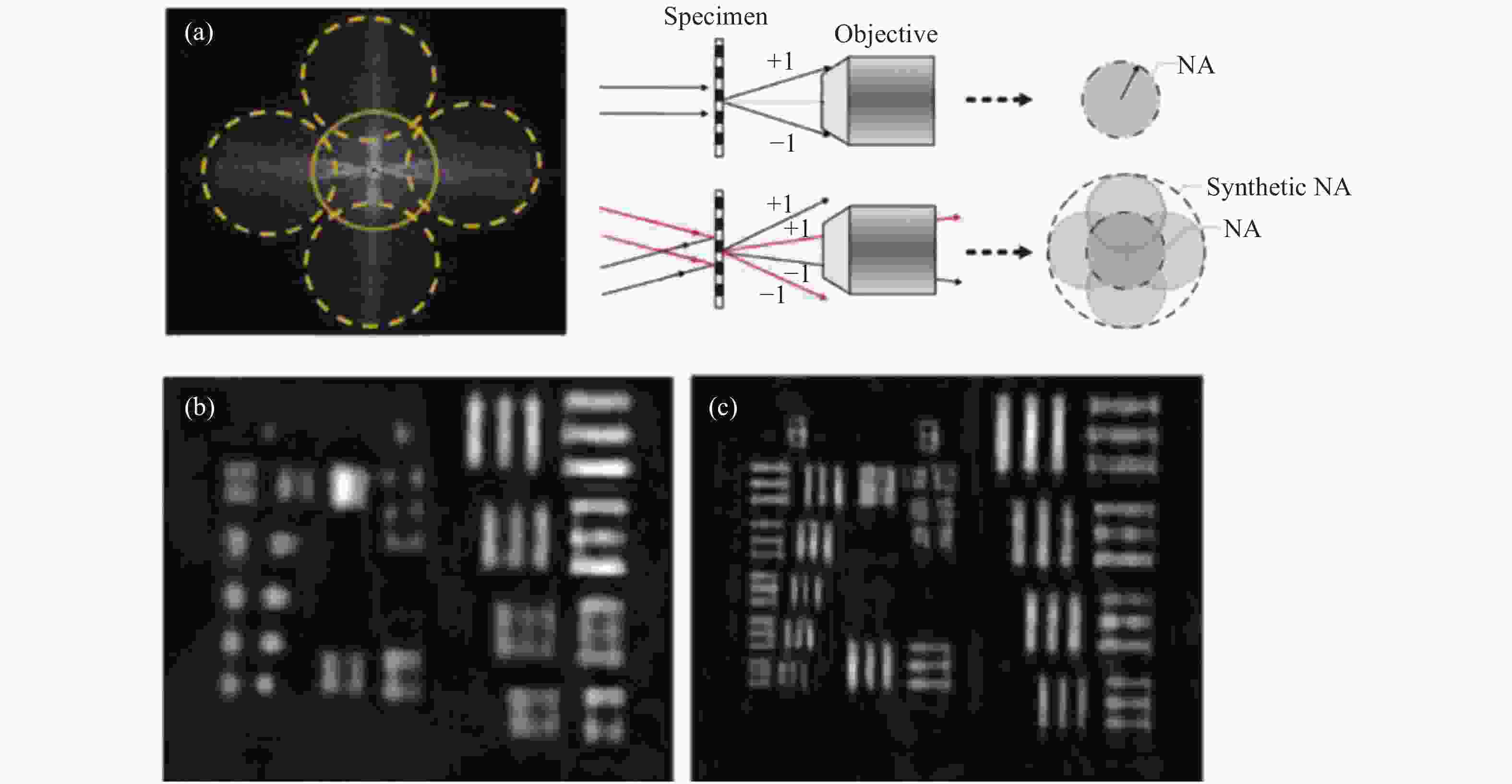
图 18 Lukosz型超分辨率系统。物体平面（OP）的信号被传播到第一个光栅（G1）。然后，编码信号通过由两个傅立叶透镜L1和L2组成的4f成像系统成像到位于第二个光栅（G2）的共轭平面。系统孔径大小为A，位于夫琅禾夫平面（FP）。解码后的信号在系统的图像平面（IP）中被观察到
Figure 18. Lukosz-type superresolution system. The signal in the object plane (OP) is propagated to the first grating (G1). The encoded signal is then imaged to the conjugate plane located at the second grating (G2) by the 4f imaging system consisting of two Fourier lenses, L1 and L2. The system aperture of size A resides in the Fraunhofer plane (FP). The decoded signal is observed in the image plane (IP) of the system
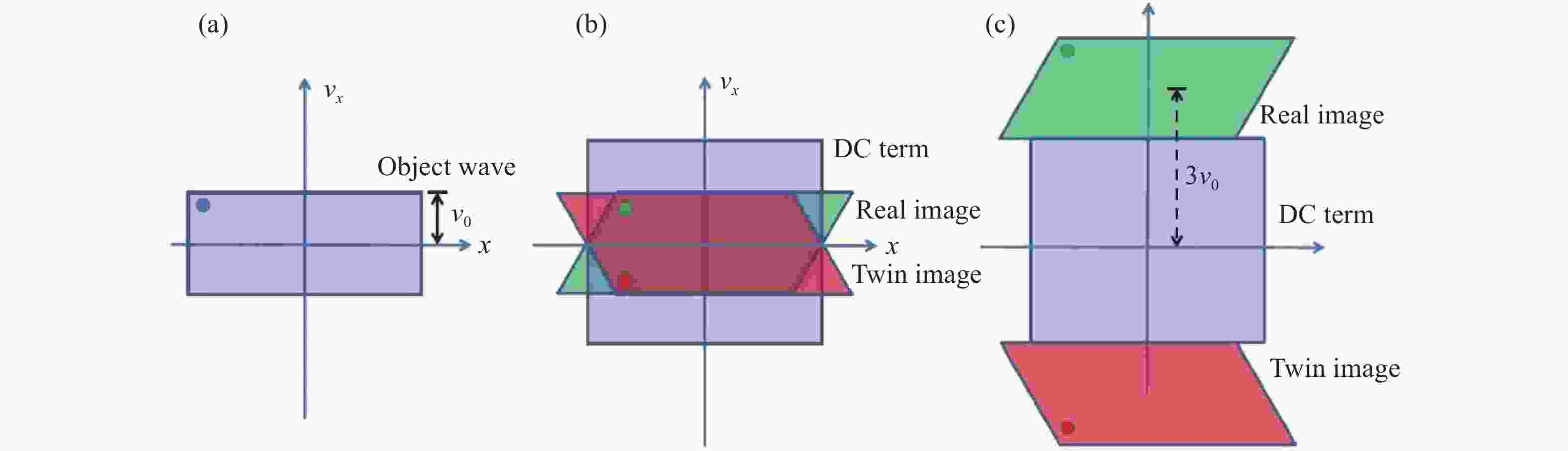
图 19 超分辨率系统的相空间图。（a）通过4f系统的信号，没有编码；（b）带宽超过4f系统通带2倍的信号；（c）第一个光栅（G1）之前；（d）G1之后；（e）通过4f系统后和第二个光栅（G2）之前的编码信号；（f）G2之后；（g）信号反传播到图像平面IP；（h）去除信号区域外的伪影之后
Figure 19. Phase-space diagram of the superresolution system. (a) Signal passing the 4f system without encoding; (b) signal with a bandwidth exceeding the pass band of the 4f system by a factor of two; (c) before the first grating (G1); (d) after G1; (e) encoded signal after passing the 4f system and before the second grating (G2); (f) after G2; (g) signal back-propagated to the image plane IP; and (h) after removing artifacts outside the signal area
图 20 最早的合成孔径“计算成像”技术——合成孔径雷达
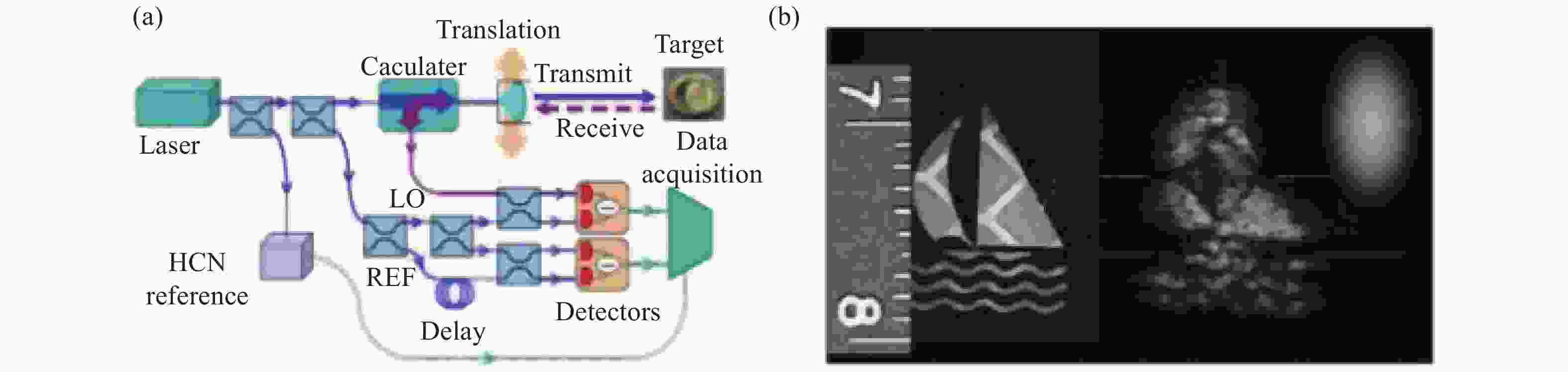
Figure 20. Synthetic Aperture Radar (SAR), the earliest computational imaging technique
图 21 合成孔径雷达技术[102]。（a）美国Aerospace公司研制的基于光纤的 合成孔径雷达成像原理图；（b）成像结果对比（右图为衍射受限成像结果，左图为合成孔径后的结果图）
Figure 21. Synthetic Aperture Ladar (SAR)[102]. (a) Principle diagram of laser synthetic aperture radar imaging based on optical fibers developed by Aerospace Corporation of the United States; (b) comparison of imaging results (right image is diffraction-limited imaging results, left image is synthetic aperture results)
图 24 非相干合成孔径技术原理[116]。（a）分时分孔径相位反演合成孔径过程；（b）基于分时分孔径相位反演合成孔径实现点扩散函数优化；（c）超分辨前后成像对比
Figure 24. Principle of incoherent synthetic aperture technology[116]. (a) Process for synthetic aperture super-resolution imaging based on time and aperture division synthetic aperture of phase reconstructive; (b) point spread function optimization based on time and aperture division synthetic aperture of phase reconstructive; (c) image comparison before and after super resolution
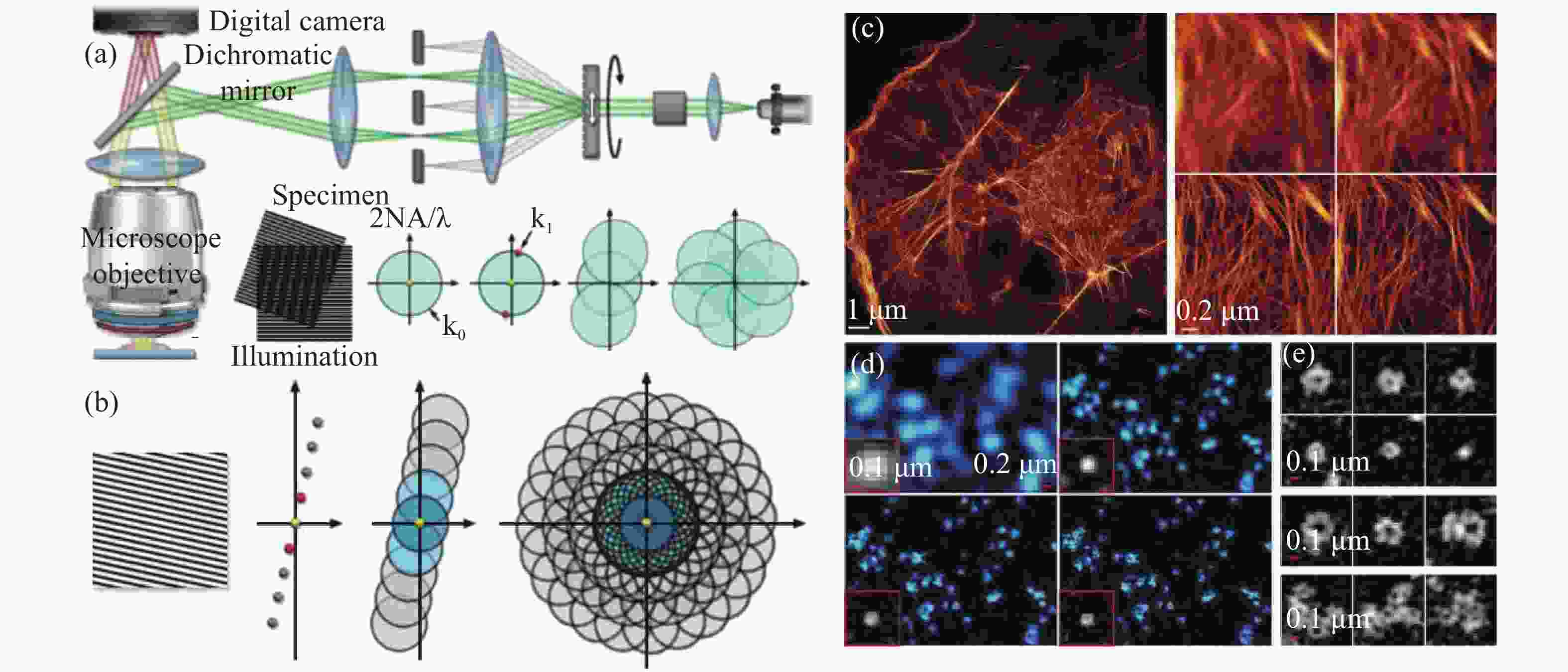
图 25 结构光照明显微术。（a）传统（线性）结构光照明显微术的光路及频谱调制过程；（b）饱和结构光照明显微技术的频谱调制过程；（c）COS-7细胞中f-肌动蛋白的饱和SIM超分辨图像及不同方法的对比结果（左上：宽场，右上：反卷积，左下：SIM，右下：SSIM）；（d）不同方法下COS-7细胞中质膜微囊的超分辨图像（左上：宽场，右上：反卷积，左下：SIM，右下：SSIM）；（e） 活体COS-7细胞中质膜微囊的SSIM超分辨结果[117,120-121]
Figure 25. Structured illumination microscopy. (a) Optical train and spectral modulation process of conventional (linear) structured illumination microscopy; (b) spectral modulation process of saturated structured illumination microscopy; (c) SIM super-resolution images of f-actin in COS-7 cells and the comparison results with different methods (upper left: wide field, upper right: deconvolution, lower left: SIM, lower right: SSIM); (d) super-resolution images of caveolae in COS-7 cells with different methods (upper left: wide field, upper right: deconvolution, lower left: SIM, lower right: SSIM); (e) SSIM super-resolution results of caveolae in living COS-7 cells[117,120-121]
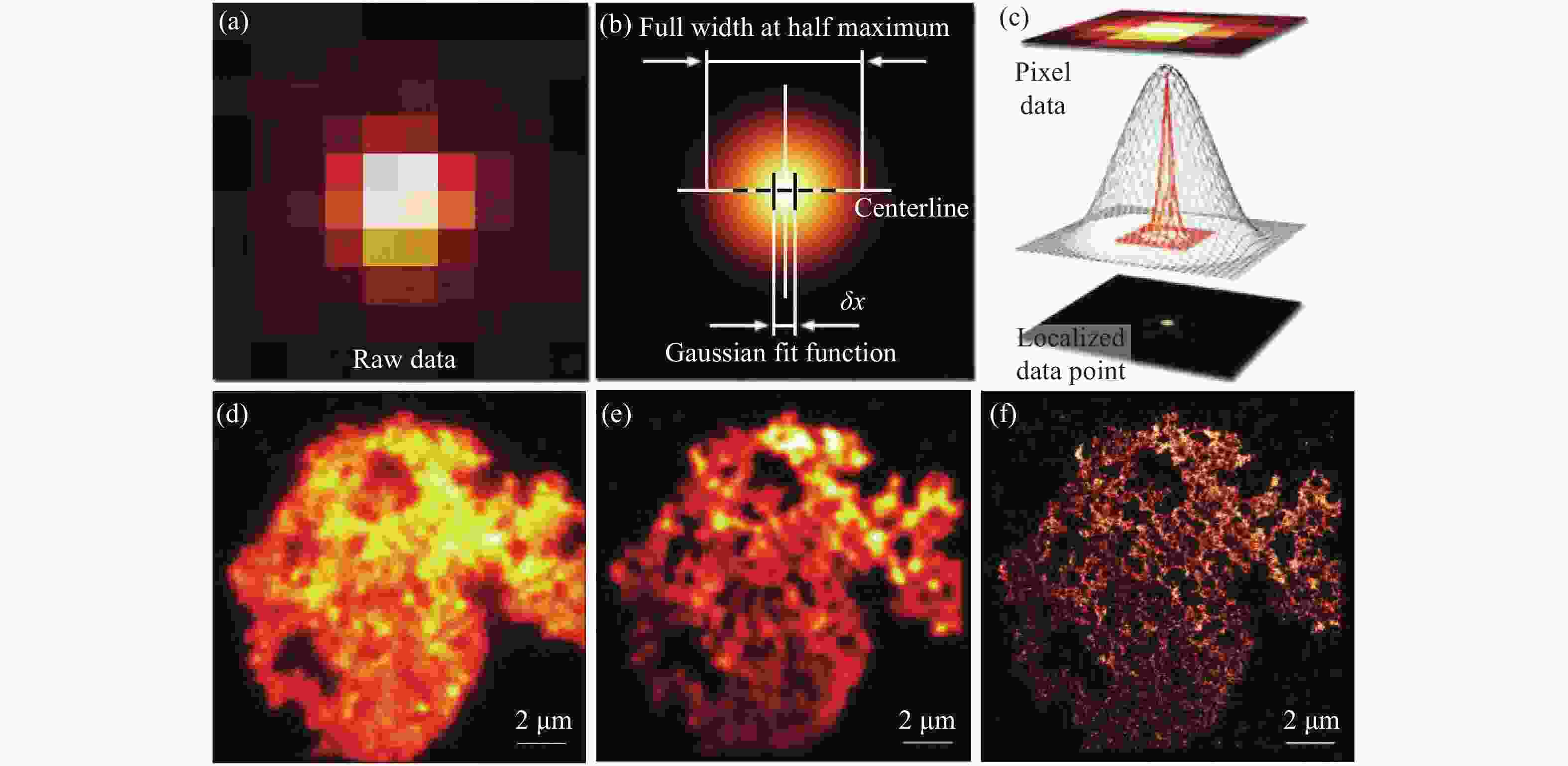
图 27 PALM的超分辨原理示意图与结果图。（a）探测的单个原始光子图像；（b）对（a）的高斯拟合；（c） 定位的（a）的中心；（d） 聚苯乙烯微球的宽场图像；（e）叠加原始PALM堆栈数据中单分子图像所获取的聚苯乙烯微球图像；（f）聚苯乙烯微球的PALM超分辨图像
Figure 27. Schematic diagram and result diagram of PALM super-resolution imaging. (a) Detected single raw photon image; (b) Gaussian fitting of (a); (c) localized center of (a); (d) wide-field image of plain polystyrene beads; (e) the plain polystyrene bead image obtained by superimposing the single molecule images in the entire PALM data stack; (f) PALM super-resolution image of plain polystyrene beads
图 29 通过线性光学变换进行带宽压缩。（a）带限函数的相空间图；（b）啁啾后的PSD；（c）经过分数傅立叶变换恢复带限函数后的PSD
Figure 29. Bandwidth compression via linear optical transformations. (a) Phase-space diagram of band-limited function; (b) PSD after chirping; (c) PSD after fractional Fourier transformation to recover band-limited function
图 30 菲涅尔全息图的广义采样。（a）空间紧凑的信号的相空间图；（b）采样域中的信号；（c）图（b）中去啁啾后的信号
Figure 30. Generalized sampling of Fresnel holograms. (a) Phase-space diagram of a signal compact in space; (b) signal in the domain of sampling; (c) signal in (b) after dechirping
图 31 可控亚像素移动所引起的像素级光强变化
Figure 31. Pixel level light intensity change caused by controllable sub-pixel movement
图 32 图像亚像素超分辨。（a）图像降采样正向模型；（b）采样频率不足产生频谱混叠效应；（c）亚像素位移超分辨重建示意图
Figure 32. Image subpixel super-resolution. (a) Image downsampling forward model; (b) spectral aliasing effect due to insufficient sampling frequency; (c) schematic diagram of subpixel shift super-resolution reconstruction
图 33 基于深度学习的单帧图像超分辨图像重构算法[184]。（a）基于图像特征提取的单帧图像超分辨率神经网络结构框图；（b） 单帧图像神经网络超分辨重构结果。虽然图像细节变清晰了，但大多与真实图像并完全不一致
Figure 33. Single-frame super-resolution image reconstruction algorithm based on deep learning[184]. (a) Block diagram of single-frame image super-resolution neural network structure based on image feature extraction; (b) results of single-frame image neural network super-resolution reconstruction. Although the image details become clearer, most of them do not match with the real image at all
图 34 多帧图像超分辨基本原理。通过进行不同的调控方式使得点扩散函数（采样矩阵）产生像素级的光强变化
Figure 34. The basic principle of multi-frame image super-resolution. The point spread function produces pixel-level light intensity variations (sampling matrix) by performing different modulation methods
图 35 微扫描装置。（a）光学折射法；（b）平板旋转法；（c）压电陶瓷体
Figure 35. Micro-scanning device. (a) Optical refraction method; (b) flat plate rotation method; (c) piezoelectric ceramics
图 36 基于孔径编码像素超分辨成像原理[116]。（a）成像系统光路结构示意图；（b）经过孔径编码调制后的点扩散函数与传统固定孔径成像对比；（c）不同编码下光学传递函数与点扩散函数分布；（d）由探测器空间采样不足所导致的频域混叠现象与孔径编码反演成像后的解调图像。
Figure 36. The principle of coded aperture pixel super-resolution imaging[116]. (a) Schematic diagram of optical path structure of imaging system; (b) the point spread function modulated by coded aperture is compared with the traditional fixed aperture imaging; (c) distribution of optical transfer function and point spread function under different patterns; (d) frequency domain aliasing caused by the insufficient spatial sampling of the detector and demodulated image after coded aperture constructive imaging
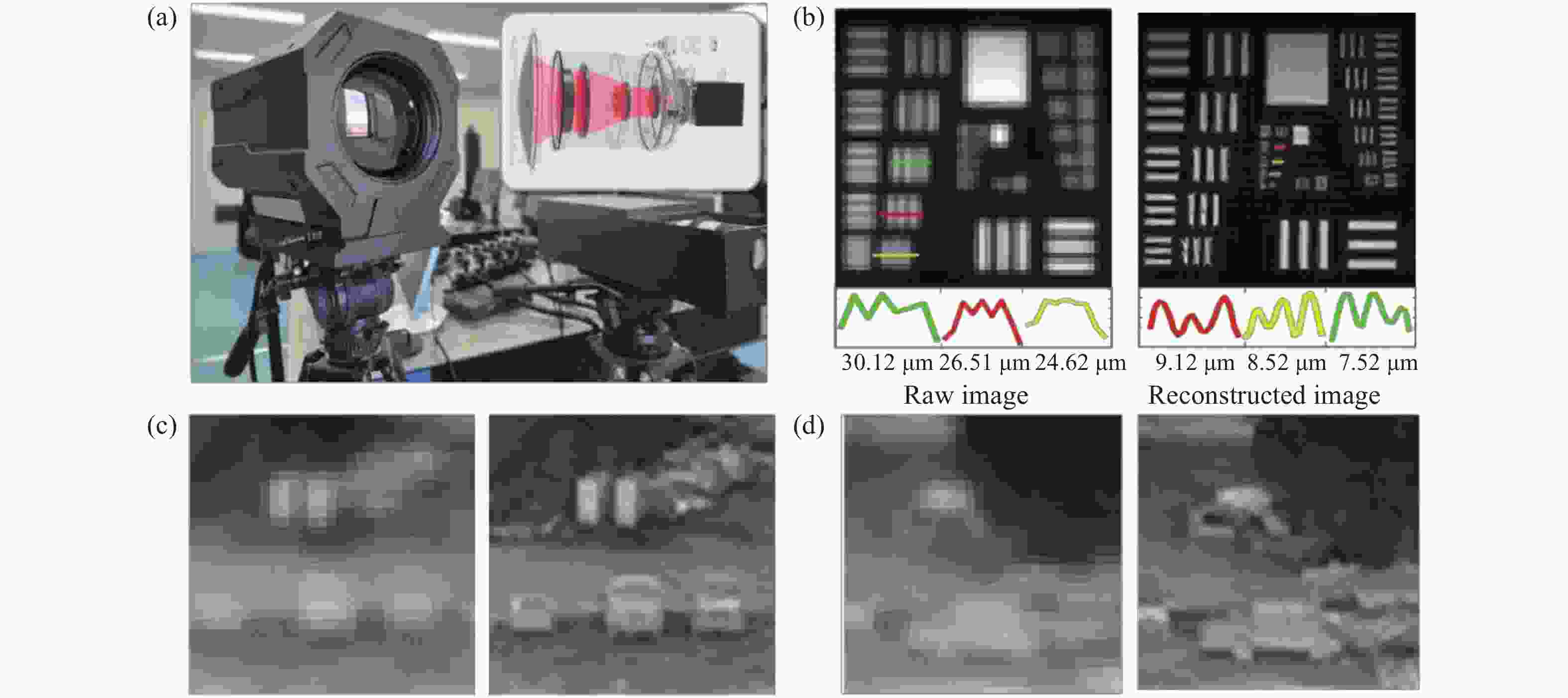
图 37 基于孔径编码的像素超分辨成像技术的典型实验结果[116]。（a）长波红外成像系统对标准分辨率靶标成像测试；（b）~（d） 采用像素超分辨算法对USAF靶标及远距离车辆前后成像效果对比
Figure 37. Typical experimental results of coded aperture-based pixel super-resolution imaging technique[116]. (a) Long-wave infrared imaging system for standard resolution target imaging test; (b)−(d) comparison of imaging resolution before and after applying pixel super-resolution algorithm on USAF target and vehicle
图 38 Gigapan全景拍摄系统及拍摄拼接所得的像素全景图
Figure 38. Gigapan panoramic imaging system and the gigapixel panorama image obtained by stitching
图 39 多片探测器进行集成与拼接。（a）由10片CCD4482芯片组成MOA-cam3；（b）由106片CCD 拼接而成的Gaia天文望远镜的焦平面阵列；（c）由21个模块组成的大型综合巡天望远镜LSST（Large Synoptic Survey Telescope）的焦平面阵列。每个模块由9个CCD 探测器组成；（d）由24块正六边形的镜片拼接组成的大天区面积多目标光谱天文望远镜的改正镜
Figure 39. Integration and stitching of multiple detectors. (a) MOA-cam3 is composed of 10 CCD4482 chips; (b) the Gaia Astronomical Telescope's focal plane array consists of 106 CCDs stitched together; (c) the focal plane array of the Large Synoptic Survey Telescope (LSST) is composed of 21 modules. Each module consists of 9 CCD detectors; (d) the correction mirror of large sky area multi-target spectral astronomical telescope is composed of 24 hexagonal lenses
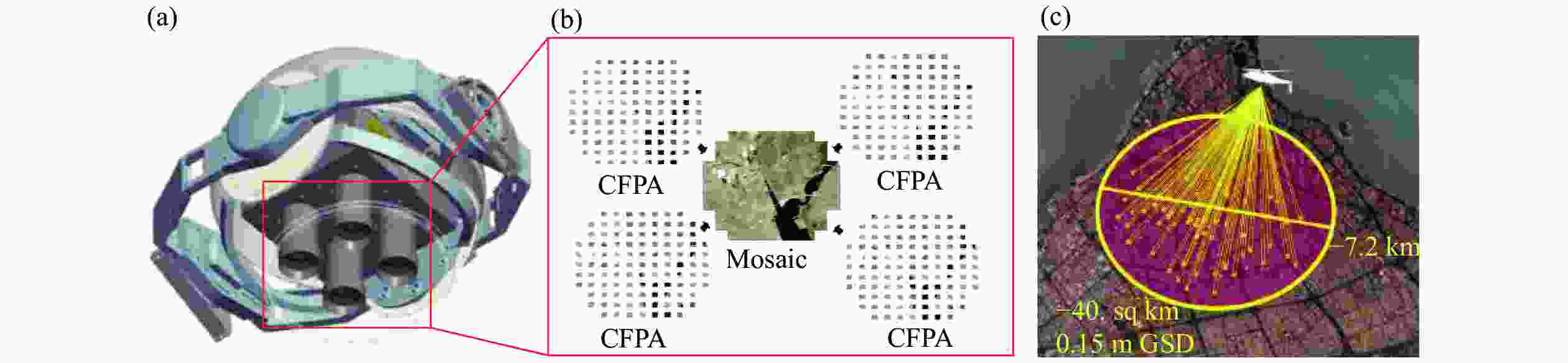
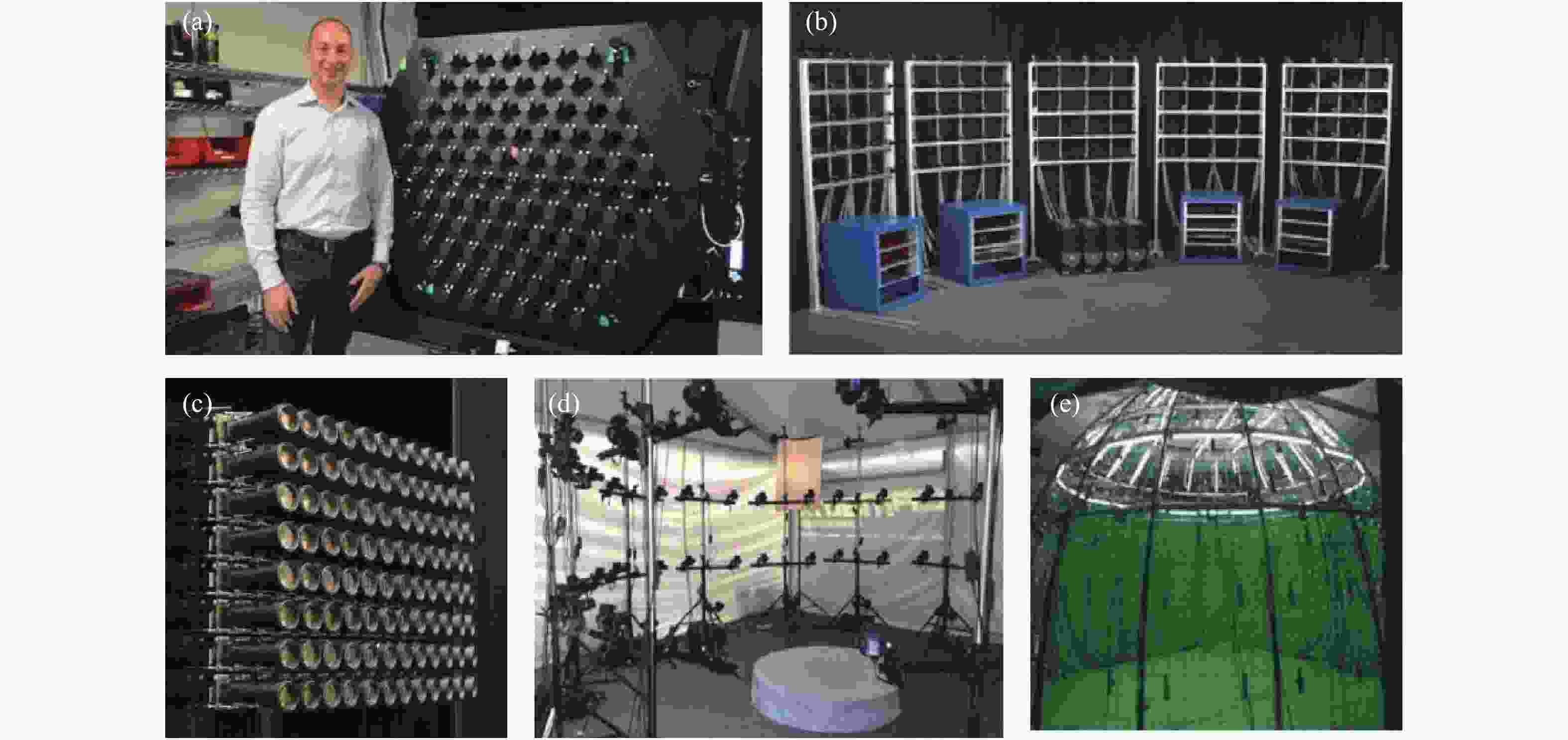
图 40 ARGUS-IS系统及其成像效果。（a）ARGUS-IS 系统外型；（b）系统采用了368个图像传感器和4个主镜头，其中92个传感器为一组，共用一个主镜头。通过巧妙设置传感器的安装位置，使得每组传感器获得的图像错位，互为补充，再通过图像拼接，能够得到较好的整体成像结果。（c）此成像系统在6000 m高空有效覆盖7.2 km×7.2 km的地面区域
Figure 40. ARGUS-IS system and its imaging results. (a) ARGUS-IS system appearance; (b) the system adopts 368 image sensors and four main lenses, of which 92 sensors are in one group and share one main lens. By skillfully setting the installation position of the sensors, the images obtained by each group of sensors are misaligned to complement each other, and then by image stitching, a better overall imaging result can be obtained. (c) This imaging system effectively covers a ground area of 7.2 km×7.2 km at 6000 m altitude
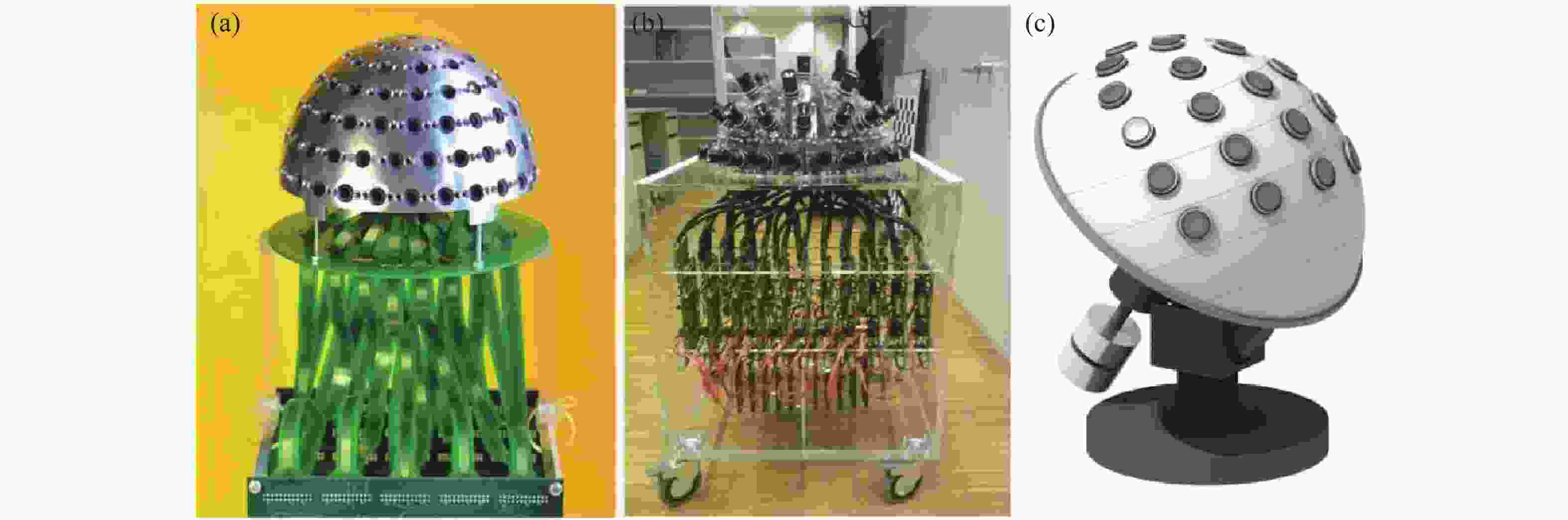
图 41 多相机拼接系统。（a）Lytro公司所研制的光场采集系统Immerge；（b）斯坦福半环型相机阵列系统；（c）斯坦福平面型相机阵列系统；（d）CAMatrix环型相机阵列系统；（e）清华大学鸟笼相机阵列系统
Figure 41. Multi-camera stitching system. (a) Immerge, a light field acquisition system developed by Lytro; (b) Stanford semi-ring camera array system; (c) Stanford planar camera array system; (d) CAMatrix ring camera array system; (e) Tsinghua University birdcage camera array system
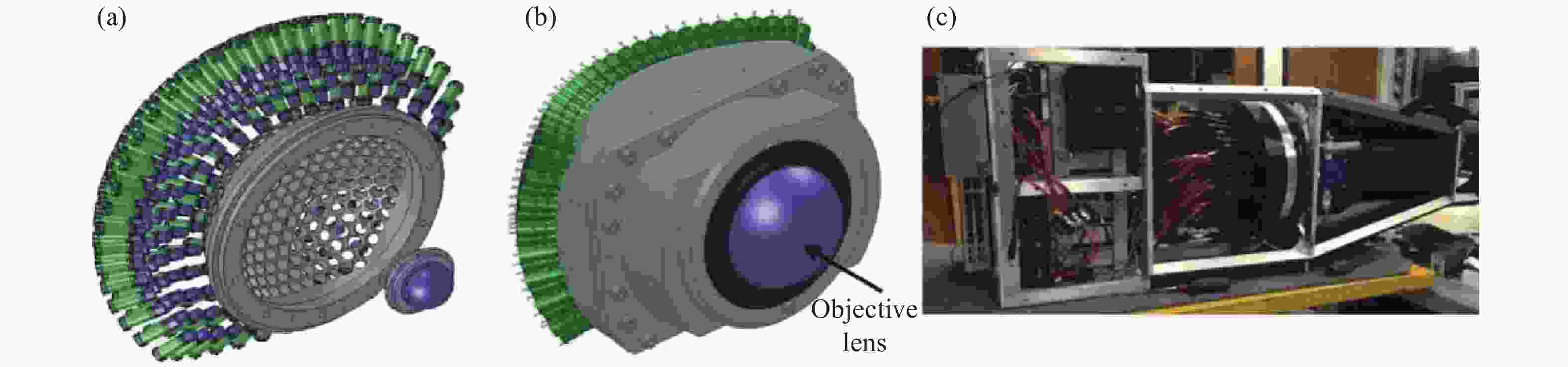
图 42 仿生复眼成像系统；（a）瑞士洛桑联邦理工学院（Swiss Federal Institute of Technology Lausanne，EPFL）的科研团队设计并研制了仿生复眼成像设备Panoptic；（b）大视场高分辨率的OMNI-R系统；（c）Nicholas Law研制的艾弗里地基望远系统Evryscope
Figure 42. Bionic compound eye imaging system; (a) Bionic compound eye imaging device Panoptic designed and developed by a research team at the Swiss Federal Institute of Technology Lausanne (EPFL); (b) large field of view high-resolution OMNI-R system; (c) Avery ground-based telescope Evryscope developed by Nicholas Law
图 43 多尺度成像系统。（a）AWARE-2结构图；（b）AWARE-10结构图；（c）AWARE-40结构图
Figure 43. Multiscale imaging system. (a) AWARE-2 architecture; (b) AWARE-10 architecture; (c) AWARE-40 architecture
图 44 不同记录结构的菲涅尔型全息图在维格纳空间的空间带宽积表示。（a）物体的原始SBP；（b）同轴全息的SBP；（c）离轴全息的SBP
Figure 44. SBP representation for different Fresnel-type holograms in Wigner space. (a) Original SBP of the object; (b) SBP for in-line geometry; and (c) SBP for off-axis geometry matching on the different elementary apertures
图 45 基于合成孔径的数字全息显微镜[220]。（a）合成孔径后的频谱图；（b）传统的单孔径低分辨率重建；（c）合成孔径后的高分辨率重建
Figure 45. Synthetic aperture-based digital holographic microscopy[220]. (a) Spectrum after synthetic aperture; (b) conventional single-aperture low-resolution reconstruction; (c) high-resolution reconstruction after synthetic aperture
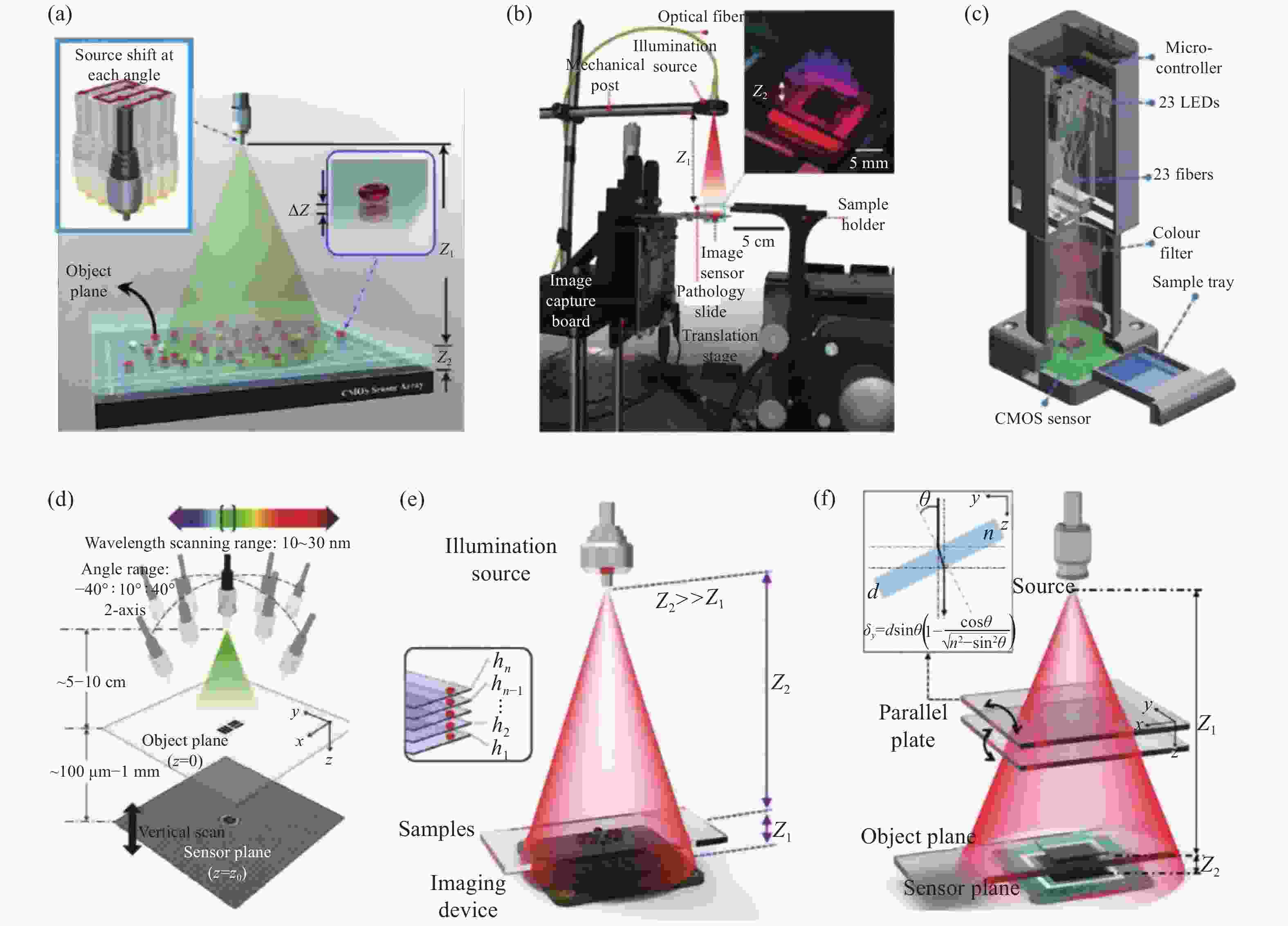
图 48 芯片上无透镜全息显微成像的“亚像素”超分辨技术。（a）照明源的亚像素移动[57]；（b）二维水平亚像素传感器移动[264]；（c）基于光纤阵列的光源扫描[268]；（d）照明波长扫描[266]；（e）多样品到传感器距离的轴向扫描[265]；（f）基于平行板的主动光源微扫描[267]
Figure 48. Schematic diagrams of the sub-pixel super-resolution based lensfree on-chip imaging setup. (a) Sub-pixel shifting of illumination source[57]; (b) 2D horizontal sub-pixel sensor motion[264]; (c) fiber-optic array based source scanning[268]; (d) illumination wavelength scanning[266]; (e) axial scanning with multiple sample-to-sensor distances[265]; (f) active source micro-scanning using parallel plates[267]
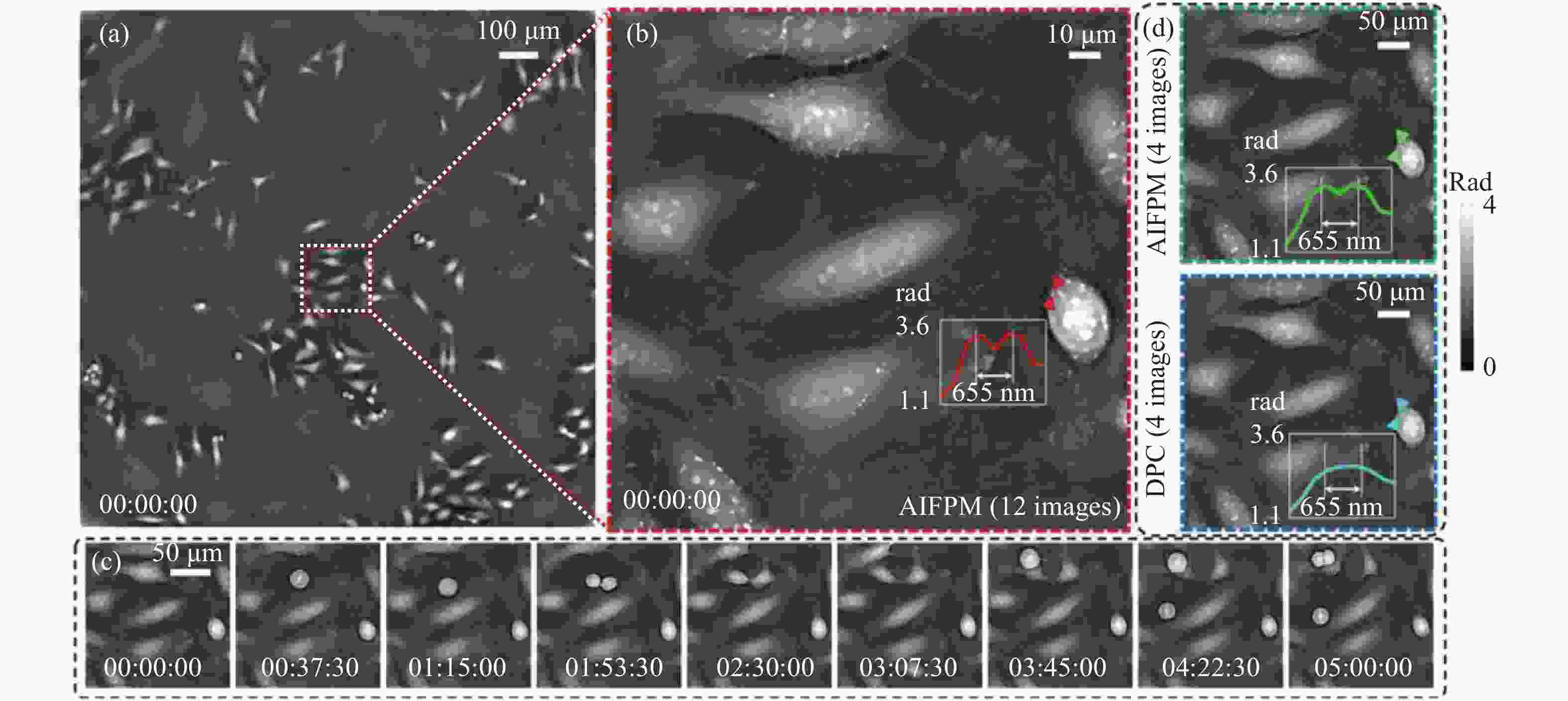
图 49 无透镜显微成像结果。（a）投影式成像系统胚胎干细胞的全场结果[255]；（b1−b9）图（a）中红框区域亚像素位移超分辨的时序结果；（c）基于多波长扫描的无透镜片上显微系统，Hela细胞的全场恢复结果[269]；（d）图（c）中Area1区域像素超分辨的时序结果
Figure 49. Lens-free microscopic imaging results. (a) Full-field results of embryonic stem cells from a projection-based imaging system[255]; (b1−b9) time-series results of subpixel shift super-resolution in the red-boxed region in (a); (c) full-field recovery results of Hela cells based on a lens-free on-chip microscopy system with multi-wavelength scanning[269]; (d) time-series results of pixel super-resolution of Area1 in (c)
图 50 通过有限区间频谱信息外推整个信号
Figure 50. Extrapolation of the entire signal through finite interval spectrum information
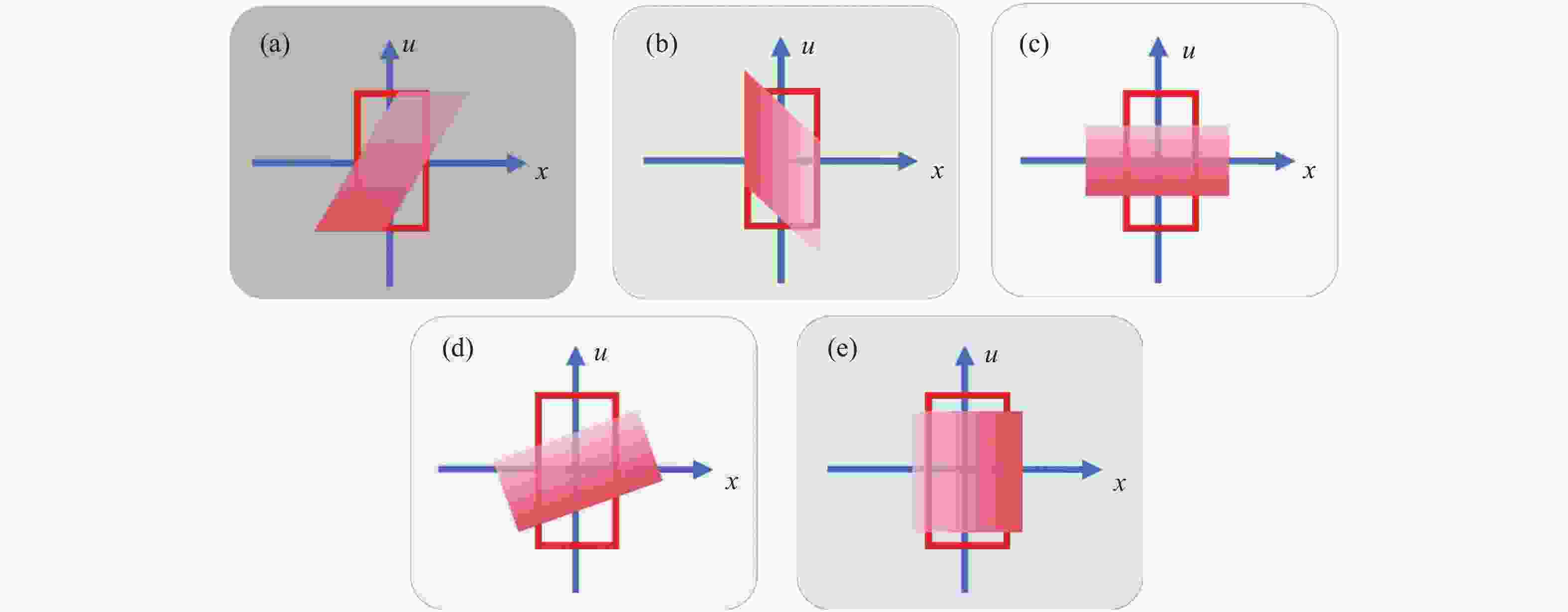
表 1 维格纳分布函数的性质
Table 1. Properties of WDF
Properties Representation Explanation Realness $ W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) \in \mathbb{R} $ W is always a real function Spatial marginal property $ I\left( {\boldsymbol{x}} \right) = \displaystyle\int {W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right){\rm{d}}{\boldsymbol{u}}} $ $ I\left(\mathit{x}\right) $ is the intensity Spatial frequency marginal property $ S\left( {\boldsymbol{u}} \right) = \displaystyle\int {W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right){\rm{d}}{\boldsymbol{x}}} $ $ S\left(\mathit{u}\right) $ is the power spectrum Convolution property $ \begin{gathered} U\left( {\boldsymbol{x}} \right) = {U_1}\left( {\boldsymbol{x}} \right){U_2}\left( {\boldsymbol{x}} \right){\text{ }}W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_1}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right)\mathop \otimes \limits_{\boldsymbol{u}} {W_2}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) \\ U\left( {\boldsymbol{x}} \right) = {U_1}\left( {\boldsymbol{x}} \right)\mathop \otimes \limits_{\boldsymbol{x}} {U_2}\left( {\boldsymbol{x}} \right){\text{ }}W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_1}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right)\mathop \otimes \limits_{\boldsymbol{x}} {W_2}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) \\ \end{gathered} $ $ \underset{\mathit{x}}{\otimes } $ is the convolution over x
$ \underset{\mathit{u}}{\otimes } $ is the convolution over uInstantaneous frequency $ \dfrac{{\displaystyle\int {{\boldsymbol{u}}W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right){\rm{d}}{\boldsymbol{u}}} }}{{\displaystyle\int {W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right){\rm{d}}{\boldsymbol{u}}} }} = \dfrac{1}{{2{\text{π}} }}\nabla \phi \left( {\boldsymbol{x}} \right) $ $ \varphi \left(\mathit{x}\right) $ is the phase component
$ \nabla \varphi \left(\mathit{x}\right) $ is the instantaneous frequency
下载: 导出CSV
表 2 维格纳分布函数的常见光学变换
Table 2. Common optical transformations of WDF
Optical transformations Representation Explanation Fresnel diffraction $ {W_{\textit{z}}}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_0}\left( {{\boldsymbol{x}} - \lambda {\textit{z}}{\boldsymbol{u}},{\boldsymbol{u}}} \right) $ λ is the wavelength
z is diffraction distanceChirp modulation (lens) $ W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_0}\left( {{\boldsymbol{x}},{\boldsymbol{u}} + \dfrac{{\boldsymbol{x}}}{{\lambda f}}} \right) $ λ is the wavelength
f is the focal length of lensFourier transform
(Fraunhofer diffraction)$ {W_{\hat U}}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_U}\left( { - {\boldsymbol{u}},{\boldsymbol{x}}} \right) $ $ \widehat{U} $ is the Fourier transform of signal Fractional Fourier transform $ {W_{{{\hat U}_\theta }}}\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_U}\left( {{\boldsymbol{x}}\cos \theta - {\boldsymbol{u}}\sin \theta ,{\boldsymbol{u}}\cos \theta + {\boldsymbol{x}}\sin \theta } \right) $ $ {\widehat{U}}_{\theta } $ is the Fractional Fourier transform, θ is the rotation angle Beam amplifier (compressor) $ W\left( {{\boldsymbol{x}},{\boldsymbol{u}}} \right) = {W_0}\left( {{\boldsymbol{x}},{{\boldsymbol{u}} \mathord{\left/ {\vphantom {{\boldsymbol{u}} M}} \right. } M}} \right) $ M is the magnification First order optical system $ \left[ \begin{gathered} {{\boldsymbol{x'}}} \\ {{\boldsymbol{u'}}} \\ \end{gathered} \right] = \left[ {\begin{array}{*{20}{c}} A&B \\ C&D \end{array}} \right]\left[ \begin{gathered} {\boldsymbol{x}} \\ {\boldsymbol{u}} \\ \end{gathered} \right] $ A, B, C, D corresponding to first order optical system
下载: 导出CSV
表 3 典型35 mm单反相机镜头的空间带宽积
Table 3. Spatial bandwidth product of typical 35 mm SLR lens
Focal length/mm Field of view
(Diagonal)/(°)Typical
F#Equivalent
NAResolution/μm
(Incident wavelength 550 nm)SBP
(Megapixel/MP)Angular
resolution/mrad8 180 3.5 0.14 2.396 3.35 0.29 20 94.5 1.8 0.27 1.242 12.4 0.06 50 46.8 1.2 0.41 0.818 28.7 0.016 85 28.6 1.4 0.35 0.959 20.9 0.011 100 24.4 2.8 0.17 1.973 4.9 0.018 200 12.3 4 0.12 2.795 2.4 0.013 400 6.2 5.6 0.08 4.193 1.1 0.009 1000 2.5 8 0.06 5.591 0.61 0.005
下载: 导出CSV
表 4 典型的显微物镜的空间带宽积
Table 4. Spatial bandwidth product of typical microscopic objectives
Objectives
(Magnification / Numerical
aperture/Field number)Resolution/nm
(Incident wavelength
532 nm)SBP
(Megapixel/MP)1.25×/0.04/26.5 8113 21.5 2×/0.08/26.5 4057 33.5 4×/0.16/26.5 2028 33.5 10×/0.3/26.5 1082 18.9 20×/0.5/26.5 649 13.1 40×/0.75/26.5 433 7.4 60×/0.9/26.5 361 4.7 100×/1.3/26.5 250 3.5
下载: 导出CSV
-
[1] COSSAIRT O S, MIAU D, NAYAR S K. Gigapixel Computational Imaging[C]//2011 IEEE International Conference on Computational Photography (ICCP). 2011: 1-8. https://doi.org/10.1109/ICCPHOT.2011.5753115. [2] BRADY D J, GEHM M E, STACK R A, et al. Multiscale gigapixel photography[J]. Nature, 2012, 486(7403): 386-389. doi: 10.1038/nature11150 [3] PARK J, BRADY D J, ZHENG G, et al. Review of bio-optical imaging systems with a high space-bandwidth product[J]. Advanced Photonics, 2021, 3(4): 044001. doi: 10.1117/1.AP.3.4.044001 [4] LIU Y, GADEPALLI K, NOROUZI M, et al.. Detecting Cancer Metastases on Gigapixel Pathology Images: arXiv: 1703.02442[R/OL]. arXiv, 2017[2022-05-21]. http://arxiv.org/abs/1703.02442. [5] ZUO, CH, CHEN Q. Exploiting optical degrees of freedom for information multiplexing in diffractive neural networks[J]. Light:Science &Applications, 2022, 11(8): 1639-1642. [6] CHEN G A. Fourier Ptychographic Imaging; A MATLAB tutorial[M]. ISBN: 978-1-6817-4273-1. IOP ebooks. Bristol, UK: IOP Publishing, 2016 [7] MAIT J N, EULISS G W, ATHALE R A. Computational imaging[J]. Advances in Optics and Photonics, 2018, 10(2): 409. doi: 10.1364/AOP.10.000409 [8] 左超, 陈钱. 计算光学成像: 何来, 何处, 何去, 何从?[J]. 红外与 工程,2022,51(2):20220110. doi: 10.3788/IRLA20220110ZUO CH, CHEN Q. Computational optical imaging: An overview[J]. Infrared and Laser Engineering, 2022, 51(2): 20220110. (in Chinese) doi: 10.3788/IRLA20220110 [9] SHANNON C E. A mathematical theory of communication[J]. The Bell System Technical Journal, 1948, 27(3): 379-423. doi: 10.1002/j.1538-7305.1948.tb01338.x [10] 韩申生, 胡晨昱. 信息光学成像研究回顾、现状与展望(特邀)[J]. 红外与 工程,2022,51(1):20220017. doi: 10.3788/IRLA20220017HAN SH SH, HU CH Y. Review, current status and prospect of researches on information optical imaging (Invited)[J]. Infrared and Laser Engineering, 2022, 51(1): 20220017. (in Chinese) doi: 10.3788/IRLA20220017 [11] HELL S W, WICHMANN J. Breaking the diffraction resolution limit by stimulated emission: stimulated-emission-depletion fluorescence microscopy[J]. Optics Letters, 1994, 19(11): 780-782. doi: 10.1364/OL.19.000780 [12] RUST M J, BATES M, ZHUANG X. Sub-diffraction-limit imaging by stochastic optical reconstruction microscopy (STORM)[J]. Nature Methods, 2006, 3(10): 793-796. doi: 10.1038/nmeth929 [13] BETZIG E, PATTERSON G H, SOUGRAT R, et al. Imaging Intracellular Fluorescent Proteins at Nanometer Resolution[J]. Science, 2006, 313(5793): 1642-1645. doi: 10.1126/science.1127344 [14] MOERNER W E, DAVID P. Methods of single-molecule fluorescence spectroscopy and microscopy[J]. Review of Scientific instruments, 2003, 74(8): 3597-3619. [15] LUKOSZ W. Optical Systems with Resolving Powers Exceeding the Classical Limit[J]. JOSA, 1966, 56(11): 1463-1471. doi: 10.1364/JOSA.56.001463 [16] LUKOSZ W. Optical Systems with Resolving Powers Exceeding the Classical Limit. II[J]. JOSA, 1967, 57(7): 932-941. doi: 10.1364/JOSA.57.000932 [17] PAPOULIS A. Generalized sampling expansion[J]. IEEE Transactions on Circuits and Systems, 1977, 24(11): 652-654. doi: 10.1109/TCS.1977.1084284 [18] BROWN J. Multi-channel sampling of low-pass signals[J]. IEEE Transactions on Circuits and Systems, 1981, 28(2): 101-106. doi: 10.1109/TCS.1981.1084954 [19] AIRY G B. On the Diffraction of an Object-glass with Circular Aperture[J]. Transactions of the Cambridge Philosophical Society, 1835, 5: 283. [20] RAYLEIGH. XV. On the theory of optical images, with special reference to the microscope[J]. The London,Edinburgh,and Dublin Philosophical Magazine and Journal of Science, 1896, 42(255): 167-195. doi: 10.1080/14786449608620902 [21] RAYLEIGH. XXXI. Investigations in optics, with special reference to the spectroscope[J]. The London,Edinburgh,and Dublin Philosophical Magazine and Journal of Science, 1879, 8(49): 261-274. doi: 10.1080/14786447908639684 [22] ABBE E. Beiträge zur Theorie des Mikroskops und der mikroskopischen Wahrnehmung[J]. Archiv für mikroskopische Anatomie,IX, 1873: 413-468. [23] SPARROW C M. On spectroscopic resolving power[J]. The Astrophysical Journal, 1916, 44: 76. doi: 10.1086/142271 [24] GOODMAN J W. Introduction to Fourier Optics[M]. Roberts and Company Publishers, 2005. [25] GOODMAN J W. Statistical optics[M]. Wiley classics library ed. New York: Wiley, 2000. [26] ZUO C, LI J, SUN J, et al. Transport of intensity equation: a tutorial[J]. Optics and Lasers in Engineering, 2020, 135: 106187. doi: 10.1016/j.optlaseng.2020.106187 [27] FAN Y, LI J, LU L, et al. Smart computational light microscopes (SCLMs) of smart computational imaging laboratory (SCILab)[J]. PhotoniX, 2021, 2(1): 19. doi: 10.1186/s43074-021-00040-2 [28] ZUO C, SUN J, LI J, et al. High-resolution transport-of-intensity quantitative phase microscopy with annular illumination[J]. Scientific Reports, 2017, 7(1): 7654. doi: 10.1038/s41598-017-06837-1 [29] SHEPPARD C J R. Partially coherent microscope imaging system in phase space: effect of defocus and phase reconstruction[J]. Journal of the Optical Society of America A, 2018, 35(11): 1846. doi: 10.1364/JOSAA.35.001846 [30] Phase retrieval from series of images obtained by defocus variation[J]. Optics Communications, 2001, 199(1-4): 65-75.https://doi.org/10.1016/S0030-4018(01)01556-5. [31] FIENUP J R. Phase retrieval algorithms: a comparison[J]. Applied Optics, 1982, 21(15): 2758-2769. doi: 10.1364/AO.21.002758 [32] TEAGUE M R. Deterministic phase retrieval: a Green’s function solution[J]. JOSA, 1983, 73(11): 1434-1441. doi: 10.1364/JOSA.73.001434 [33] TIAN L, WALLER L. Quantitative differential phase contrast imaging in an LED array microscope[J]. Optics Express, 2015, 23(9): 11394-11403. doi: 10.1364/OE.23.011394 [34] FAN Y, SUN J, CHEN Q, et al. Optimal illumination scheme for isotropic quantitative differential phase contrast microscopy[J]. Photonics Research, 2019, 7(8): 890-904. doi: 10.1364/PRJ.7.000890 [35] ZHENG G, HORSTMEYER R, YANG C. Wide-field, high-resolution Fourier ptychographic microscopy[J]. Nature Photonics, 2013, 7(9): 739-745. doi: 10.1038/nphoton.2013.187 [36] SUN J, ZUO C, ZHANG J, et al.. High-speed Fourier ptychographic microscopy based on programmable annular illuminations[J]. Scientific Reports, 2018, 8(1)[2018-09-11]. http://www.nature.com/articles/s41598-018-25797-8. [37] AKONDI V, CASTILLO S, VOHNSEN B. Digital pyramid wavefront sensor with tunable modulation[J]. Optics Express, 2013, 21(15): 18261. doi: 10.1364/OE.21.018261 [38] BURVALL A, DALY E, CHAMOT S R, et al. Linearity of the pyramid wavefront sensor[J]. Optics Express, 2006, 14(25): 11925. doi: 10.1364/OE.14.011925 [39] ZUO C, SUN J, FENG S, et al. Programmable aperture microscopy: A computational method for multi-modal phase contrast and light field imaging[J]. Optics and Lasers in Engineering, 2016, 80: 24-31. doi: 10.1016/j.optlaseng.2015.12.012 [40] IGLESIAS I. Pyramid phase microscopy[J]. Optics Letters, 2011, 36(18): 3636. doi: 10.1364/OL.36.003636 [41] HOPKINS H H. On the Diffraction Theory of Optical Images[J]. Proceedings of the Royal Society A:Mathematical,Physical and Engineering Sciences, 1953, 217(1130): 408-432. doi: 10.1098/rspa.1953.0071 [42] STREIBL N. Three-dimensional imaging by a microscope[J]. JOSA A, 1985, 2(2): 121-127. doi: 10.1364/JOSAA.2.000121 [43] SHEPPARD C J. Defocused transfer function for a partially coherent microscope and application to phase retrieval[J]. JOSA A, 2004, 21(5): 828-831. doi: 10.1364/JOSAA.21.000828 [44] MTF - Modulation transfer function[EB/OL]. [2022-05-22].https://www.telescope-optics.net/mtf.htm. [45] HORSTMEYER R, CHUNG J, OU X, et al. Diffraction tomography with Fourier ptychography[J]. Optica, 2016, 3(8): 827-835. doi: 10.1364/OPTICA.3.000827 [46] ASAKURA T. Resolution of two unequally bright points with partially coherent light[J]. Nouvelle Revue d’Optique, 1974, 5(3): 169-177. doi: 10.1088/0335-7368/5/3/304 [47] AERT S V, DYCK D V, DEKKER A J den. Resolution of coherent and incoherent imaging systems reconsidered - Classical criteria and a statistical alternative[J]. Optics Express, 2006, 14(9): 3830-3839. doi: 10.1364/OE.14.003830 [48] VILLIERS G de, PIKE E R. The Limits of Resolution[M/OL]. Boca Raton: CRC Press, 2016. https://doi.org/10.1201/9781315366708. [49] LATYCHEVSKAIA T. Lateral and axial resolution criteria in incoherent and coherent optics and holography, near- and far-field regimes[J]. Applied Optics, 2019, 58(13): 3597-3603. doi: 10.1364/AO.58.003597 [50] COTTE Y, TOY M F, DEPEURSINGE C. Beyond the lateral resolution limit by phase imaging[J]. Journal of biomedical optics, 2011, 16(10): 106007-106007. doi: 10.1117/1.3640812 [51] HORSTMEYER R, HEINTZMANN R, POPESCU G, et al. Standardizing the resolution claims for coherent microscopy[J]. Nature Photonics, 2016, 10(2): 68-71. doi: 10.1038/nphoton.2015.279 [52] SIGAL Y M, ZHOU R, ZHUANG X. Visualizing and discovering cellular structures with super-resolution microscopy[J]. Science, 2018, 361(6405): 880-887. doi: 10.1126/science.aau1044 [53] JERRI A J. The Shannon sampling theorem—Its various extensions and applications: A tutorial review[J]. Proceedings of the IEEE, 1977, 65(11): 1565-1596. doi: 10.1109/PROC.1977.10771 [54] HARDIE R. A Fast Image Super-Resolution Algorithm Using an Adaptive Wiener Filter[J]. IEEE Transactions on Image Processing, 2007, 16(12): 2953-2964. doi: 10.1109/TIP.2007.909416 [55] SJAARDEMA T A, SMITH C S, BIRCH G C. History and Evolution of the Johnson Criteria. SAND2015-6368[R/OL]. Sandia National Lab. (SNL-NM), Albuquerque, NM (United States), 2015[2022-05-22]. https://www.osti.gov/biblio/1222446. [56] ROBINSON J, KINCH M, MARQUIS M, et al.. Case for small pixels: system perspective and FPA challenge[C/OL]//Image Sensing Technologies: Materials, Devices, Systems, and Applications: 9100. SPIE, 2014: 73-81[2022-05-22]. https://www.spiedigitallibrary.org/conference-proceedings-of-spie/9100/91000I/Case-for-small-pixels-system-perspective-and-FPA-challenge/10.1117/12.2054452.full. [57] 张佳琳, 陈钱, 张翔宇, 等. 无透镜片上显微成像技术: 理论、发展与应用[J]. 红外与 工程,2019,48(6):0603009. doi: 10.3788/IRLA201948.0603009ZHANG J L, CHEN Q, ZHANG X Y, et al. Lens-free on-chip microscopy:theory, advances, and applications[J]. Infrared and Laser Engineering, 2019, 48(6): 0603009. (in Chinese) doi: 10.3788/IRLA201948.0603009 [58] BISHARA W, SU T W, COSKUN A F, et al. Lensfree on-chip microscopy over a wide field-of-view using pixel super-resolution[J]. Optics Express, 2010, 18(11): 11181. doi: 10.1364/OE.18.011181 [59] SUN J, CHEN Q, ZHANG Y, et al. Sampling criteria for Fourier ptychographic microscopy in object space and frequency space[J]. Optics Express, 2016, 24(14): 15765. doi: 10.1364/OE.24.015765 [60] FAN Y, SUN J, CHEN Q, et al. Wide-field anti-aliased quantitative differential phase contrast microscopy[J]. Optics Express, 2018, 26(19): 25129. doi: 10.1364/OE.26.025129 [61] SHANNON C E. Communication in the Presence of Noise[J]. Proceedings of the IRE, 1949, 37(1): 10-21. doi: 10.1109/JRPROC.1949.232969 [62] V. LAUVE M. Die Freiheitsgrade von Strahlenbündeln[J]. Annalen der Physik, 1914, 349(16): 1197-1212. doi: 10.1002/andp.19143491606 [63] GABOR D. IV Light and Information. This article is the substance of a Ritchie lecture, delivered by the author on March 2, 1951 at the University of Edinburgh. The contents of the lecture became known to a wider audience through the distribution of a limited number of mimeographed notes, which have since become widely quoted in the literature. The wish has been often expressed that a permanent record of the lecture should be made generally available. We are glad to be able to meet this wish[M/OL]//WOLF E. Progress in Optics: 1. Elsevier, 1961: 109-153[2022-05-22]. https://www.sciencedirect.com/science/article/pii/S0079663808706097. [64] GREIVENKAMP J E. Field Guide to Geometrical Optics[M/OL]. 1000 20th Street, Bellingham, WA 98227-0010 USA: SPIE, 2004[2022-05-22]. http://link.aip.org/link/doi/10.1117/3.547461. [65] FRANCIA G T di. Resolving Power and Information[J]. JOSA, 1955, 45(7): 497-501. doi: 10.1364/JOSA.45.000497 [66] LOHMANN A W, DORSCH R G, MENDLOVIC D, et al. Space–bandwidth product of optical signals and systems[J]. JOSA A, 1996, 13(3): 470-473. doi: 10.1364/JOSAA.13.000470 [67] SLEPIAN D, POLLAK H O. Prolate Spheroidal Wave Functions, Fourier Analysis and Uncertainty — I[J]. Bell System Technical Journal, 1961, 40(1): 43-63. doi: 10.1002/j.1538-7305.1961.tb03976.x [68] LANDAU H J, POLLAK H O. Prolate Spheroidal Wave Functions, Fourier Analysis and Uncertainty — II[J]. Bell System Technical Journal, 1961, 40(1): 65-84. doi: 10.1002/j.1538-7305.1961.tb03977.x [69] FRANCIA G T di. Degrees of Freedom of an Image[J]. JOSA, 1969, 59(7): 799-804. doi: 10.1364/JOSA.59.000799 [70] TESTORF M E, HENNELLY B M, OJEDA-CASTAÑEDA J. Phase-space optics: fundamentals and applications[M/OL]. New York: McGraw-Hill, 2010[2019-05-10]. http://accessengineeringlibrary.com/browse/phase-space-optics-fundamentals-and-applications. [71] WIGNER E. On the Quantum Correction For Thermodynamic Equilibrium[J]. Physical Review, 1932, 40(5): 749-759. doi: 10.1103/PhysRev.40.749 [72] DOLIN L. Beam description of weakly-inhomogeneous wave fields[J]. Izv Vyssh Uchebn Zaved Radiofiz, 1964, 7: 559-563. [73] WALTHER A. Radiometry and Coherence[J]. JOSA, 1968, 58(9): 1256-1259. doi: 10.1364/JOSA.58.001256 [74] WALTHER A. Radiometry and coherence[J]. JOSA, 1973, 63(12): 1622-1623. doi: 10.1364/JOSA.63.001622 [75] WALTHER A. Propagation of the generalized radiance through lenses[J]. JOSA, 1978, 68(11): 1606-1610. doi: 10.1364/JOSA.68.001606 [76] BASTIAANS M J. A Frequency-domain Treatment of Partial Coherence[J]. Optica Acta:International Journal of Optics, 1977, 24(3): 261-274. doi: 10.1080/713819536 [77] BASTIAANS M J. The Wigner distribution function applied to optical signals and systems[J]. Optics Communications, 1978, 25(1): 26-30. doi: 10.1016/0030-4018(78)90080-9 [78] BASTIAANS M J. The wigner distribution function and Hamilton’s characteristics of a geometric-optical system[J]. Optics Communications, 1979, 30(3): 321-326. doi: 10.1016/0030-4018(79)90361-4 [79] BASTIAANS M J. Transport Equations for the Wigner Distribution Function[J]. Optica Acta:International Journal of Optics, 1979, 26(10): 1265-1272. doi: 10.1080/713819904 [80] BASTIAANS M J. Wigner distribution function and its application to first-order optics[J]. JOSA, 1979, 69(12): 1710-1716. doi: 10.1364/JOSA.69.001710 [81] BASTIAANS M J. Transport Equations for the Wigner Distribution Function in an Inhomogeneous and Dispersive Medium[J]. Optica Acta:International Journal of Optics, 1979: [2021-02-18 doi: 10.1080/713819921 [82] BASTIAANS M J. The Wigner Distribution Function of Partially Coherent Light[J]. Optica Acta:International Journal of Optics, 1981: [2021-02-18 doi: 10.1080/713820722 [83] BASTIAANS M J. Application of the Wigner distribution function to partially coherent light[J]. Journal of the Optical Society of America A, 1986, 3(8): 1227. doi: 10.1364/JOSAA.3.001227 [84] ZHENGYUN ZHANG, LEVOY M. Wigner distributions and how they relate to the light field[C/OL]//2009 IEEE International Conference on Computational Photography (ICCP). 2009: 1-10. https://doi.org/10.1109/ICCPHOT.2009.5559007. [85] DRAGOMAN D. Phase-space interferences as the source of negative values of the Wigner distribution function[J]. JOSA A, 2000, 17(12): 2481-2485. doi: 10.1364/JOSAA.17.002481 [86] TESTORF M E, FIDDY M A. Superresolution Imaging—Revisited[M/OL]//Advances in Imaging and Electron Physics: 163. Elsevier, 2010: 165-218[2022-05-16]. https://linkinghub.elsevier.com/retrieve/pii/S1076567010630054. [87] TESTORF M. Phase-Space Optics and Modern Imaging Systems[J]. 2011: 67. [88] MENDLOVIC D, LOHMANN A W. Space–bandwidth product adaptation and its application to superresolution: fundamentals[J]. JOSA A, 1997, 14(3): 558-562. doi: 10.1364/JOSAA.14.000558 [89] MENDLOVIC D, LOHMANN A W, ZALEVSKY Z. Space–bandwidth product adaptation and its application to superresolution: examples[J]. JOSA A, 1997, 14(3): 563-567. doi: 10.1364/JOSAA.14.000563 [90] NEIFELD M A. Information, resolution, and space–bandwidth product[J]. Optics Letters, 1998, 23(18): 1477-1479. doi: 10.1364/OL.23.001477 [91] ZHENG G, OU X, HORSTMEYER R, et al. Fourier Ptychographic Microscopy: A Gigapixel Superscope for Biomedicine[J]. Optics and Photonics News, 2014, 25(4): 26-33. doi: 10.1364/OPN.25.4.000026 [92] SUN J, ZHANG J, CHEN Q, et al. Fourier ptychographic microscopy theory advances and applications[J]. Acta Optica Sinica, 2016, 36(10): 1011005. doi: 10.3788/AOS201636.1011005 [93] TIAN L, LIU Z, YEH L H, et al. Computational illumination for high-speed in vitro Fourier ptychographic microscopy[J]. Optica, 2015, 2(10): 904. doi: 10.1364/OPTICA.2.000904 [94] SUN J, CHEN Q, ZHANG J, et al. Single-shot quantitative phase microscopy based on color-multiplexed Fourier ptychography[J]. Optics Letters, 2018, 43(14): 3365. doi: 10.1364/OL.43.003365 [95] ZALEVSKY Z, MENDLOVIC D, LOHMANN A W. IV Optical systems with improved resolving power[M/OL]//WOLF E. Progress in Optics: 40. Elsevier, 2000: 271-341[2022-11-20]. https://www.sciencedirect.com/science/article/pii/S0079663800800323. [96] BACHL A, LUKOSZ W. Experiments on superresolution imaging of a reduced object field[J]. JOSA, 1967, 57(2): 163-169. doi: 10.1364/JOSA.57.000163 [97] 陶纯堪, 陶纯匡. 光学信息论[M]. 科学出版社, 1999[2022-05-22].TAO C K, TAO C K. Optical Information Theory[M]. Science Press, 1999[2022-05-22]. [98] ZALESVKY Z. Super Resolved Imaging in Wigner-Based Phase Space[M]//Phase-Space Optics: Fundamentals and Applications. McGraw-Hill New York, 2009: 193-216. [99] ZALEVSKY Z, MENDLOVIC D, LOHMANN A W. Understanding superresolution in Wigner space[J]. JOSA A, 2000, 17(12): 2422-2430. doi: 10.1364/JOSAA.17.002422 [100] BROWN W M. Synthetic Aperture Radar[J]. IEEE Transactions on Aerospace and Electronic Systems, 1967, AES-3(2): 217-229. doi: 10.1109/TAES.1967.5408745 [101] LUCKE R L, RICKARD L J, BASHKANSKY M, et al.. Synthetic aperture ladar (SAL): fundamental theory, design equations for a satellite system, and laboratory demonstration[R]. NAVAL RESEARCH LAB WASHINGTON DC, 2002. [102] BASHKANSKY M, LUCKE R L, FUNK E, et al. Two-dimensional synthetic aperture imaging in the optical domain[J]. Optics Letters, 2002, 27(22): 1983. doi: 10.1364/OL.27.001983 [103] BECK S M, BUCK J R, BUELL W F, et al. Synthetic-aperture imaging laser radar: laboratory demonstration and signal processing[J]. Applied Optics, 2005, 44(35): 7621. doi: 10.1364/AO.44.007621 [104] GARCÍA J, MICÓ V, GARCÍA-MARTÍNEZ P, et al.. Synthetic Aperture Superresolution by Structured Light Projection[C/OL]//AIP Conference Proceedings: 860. Toledo (Spain): AIP, 2006: 136-145[2019-06-05]. http://aip.scitation.org/doi/abs/10.1063/1.2361214. [105] GARCÍA J, ZALEVSKY Z, FIXLER D. Synthetic aperture superresolution by speckle pattern projection[J]. Optics Express, 2005, 13(16): 6073. doi: 10.1364/OPEX.13.006073 [106] RICHARD B. Principles of synthetic aperture radar[J]. Surveys in Geophysics, 2000, 21(2): 147-157. [107] KOCH B. Status and future of laser scanning, synthetic aperture radar and hyperspectral remote sensing data for forest biomass assessment[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2010, 65(6): 581-590. doi: 10.1016/j.isprsjprs.2010.09.001 [108] HORSTMEYER R, CHEN R Y, OU X, et al. Solving ptychography with a convex relaxation[J]. New Journal of Physics, 2015, 17(5): 053044. doi: 10.1088/1367-2630/17/5/053044 [109] YEH L H, DONG J, ZHONG J, et al. Experimental robustness of Fourier ptychography phase retrieval algorithms[J]. Optics Express, 2015, 23(26): 33214. doi: 10.1364/OE.23.033214 [110] ZUO C, SUN J, CHEN Q. Adaptive step-size strategy for noise-robust Fourier ptychographic microscopy[J]. Optics Express, 2016, 24(18): 20724. doi: 10.1364/OE.24.020724 [111] HOLLOWAY J, ASIF M S, SHARMA M K, et al.. Toward Long Distance, Sub-diffraction Imaging Using Coherent Camera Arrays[J]. arXiv: 1510.08470 [physics], 2015[2019-12-18]. http://arxiv.org/abs/1510.08470. [112] HOLLOWAY J, WU Y, SHARMA M K, et al. SAVI: Synthetic apertures for long-range, subdiffraction-limited visible imaging using Fourier ptychography[J]. Science Advances, 2017, 3(4): e1602564. doi: 10.1126/sciadv.1602564 [113] KENDRICK R L, DUNCAN A, OGDEN C, et al.. Segmented Planar Imaging Detector for EO Reconnaissance[C]//Imaging and Applied Optics. Arlington, Virginia: OSA, 2013: CM4C. 1[2021-03-10]. [114] KENDRICK R L, DUNCAN A, OGDEN C, et al.. Flat-panel space-based space surveillance sensor[C]//Advanced maui optical and space surveillance technologies conference. 2013: E45. [115] KATZ B, ROSEN J. Super-resolution in incoherent optical imaging using synthetic aperture with Fresnel elements[J]. Optics Express, 2010, 18(2): 962-972. doi: 10.1364/OE.18.000962 [116] 陈钱. 先进夜视成像技术发展探讨[J]. 红外与 工程,2022,51(2):20220128.CHEN Q. Discussions on the development of advanced night vision imaging technology[J]. Infrared and Laser Engineering, 2022, 51(2): 20220128. (in Chinese) [117] GUSTAFSSON M G. Surpassing the lateral resolution limit by a factor of two using structured illumination microscopy[J]. Journal of microscopy, 2000, 198(2): 82-87. doi: 10.1046/j.1365-2818.2000.00710.x [118] EGGELING C, WILLIG K I, SAHL S J, et al. Lens-based fluorescence nanoscopy[J]. Quarterly reviews of biophysics, 2015, 48(2): 178-243. doi: 10.1017/S0033583514000146 [119] KNER P, CHHUN B B, GRIFFIS E R, et al. Super-resolution video microscopy of live cells by structured illumination[J]. Nature methods, 2009, 6(5): 339. doi: 10.1038/nmeth.1324 [120] GUSTAFSSON M G. Nonlinear structured-illumination microscopy: wide-field fluorescence imaging with theoretically unlimited resolution[J]. Proceedings of the National Academy of Sciences, 2005, 102(37): 13081-13086. doi: 10.1073/pnas.0406877102 [121] LI D, SHAO L, CHEN B C, et al. Extended-resolution structured illumination imaging of endocytic and cytoskeletal dynamics[J]. Science, 2015, 349(6251): aab3500-aab3500. doi: 10.1126/science.aab3500 [122] MICÓ V, ZHENG J, GARCIA J, et al. Resolution enhancement in quantitative phase microscopy[J]. Advances in Optics and Photonics, 2019, 11(1): 135. doi: 10.1364/AOP.11.000135 [123] GAO P, PEDRINI G, OSTEN W. Structured illumination for resolution enhancement and autofocusing in digital holographic microscopy[J]. Optics Letters, 2013, 38(8): 1328. doi: 10.1364/OL.38.001328 [124] WICKER K, HEINTZMANN R. Resolving a misconception about structured illumination[J]. Nature Photonics, 2014, 8(5): 342-344. doi: 10.1038/nphoton.2014.88 [125] KLAR T A, JAKOBS S, DYBA M, et al. Fluorescence microscopy with diffraction resolution barrier broken by stimulated emission[J]. Proceedings of the National Academy of Sciences, 2000, 97(15): 8206-8210. doi: 10.1073/pnas.97.15.8206 [126] HELL S W. Far-Field Optical Nanoscopy[J]. Science, 2007, 316(5828): 1153-1158. doi: 10.1126/science.1137395 [127] WILLIG K I, RIZZOLI S O, WESTPHAL V, et al. STED microscopy reveals that synaptotagmin remains clustered after synaptic vesicle exocytosis[J]. Nature, 2006, 440(7086): 935. doi: 10.1038/nature04592 [128] HELL S W, SAHL S J, MARK B M et al. The 2015 super-resolution microscopy roadmap[J]. Journal of Physics D:Applied Physics, 2015, 48(44): 443001. [129] HESS S T, GIRIRAJAN T P, MASON M D. Ultra-high resolution imaging by fluorescence photoactivation localization microscopy[J]. Biophysical journal, 2006, 91(11): 4258-4272. doi: 10.1529/biophysj.106.091116 [130] DI FRANCIA G T. Super-gain antennas and optical resolving power[J]. Il Nuovo Cimento ( , 1943, -1954),1952,9(3): 426-438. [131] MARTÍNEZ-CORRAL M, ANDRÉS P, OJEDA-CASTAÑEDA J, et al. Tunable axial superresolution by annular binary filters. Application to confocal microscopy[J]. Optics Communications, 1995, 119(5-6): 491-498. doi: 10.1016/0030-4018(95)00380-Q [132] SALES T R, MORRIS G M. Diffractive superresolution elements[J]. JOSA A, 1997, 14(7): 1637-1646. doi: 10.1364/JOSAA.14.001637 [133] SHEPPARD C, CALVERT G, WHEATLAND M. Focal distribution for superresolving toraldo filters[J]. JOSA A, 1998, 15(4): 849-856. doi: 10.1364/JOSAA.15.000849 [134] ZHANG Y. Design of three-dimensional superresolving binary amplitude filters by using the analytical method[J]. Optics Communications, 2007, 274(1): 37-42. doi: 10.1016/j.optcom.2007.01.062 [135] AHARONOV Y, ANANDAN J, POPESCU S, et al. Superpositions of time evolutions of a quantum system and a quantum time-translation machine[J]. Physical review letters, 1990, 64(25): 2965. doi: 10.1103/PhysRevLett.64.2965 [136] BERRY M V. Evanescent and real waves in quantum billiards and Gaussian beams[J]. Journal of Physics A:Mathematical and General, 1994, 27(11): L391. doi: 10.1088/0305-4470/27/11/008 [137] BERRY M. Faster than fourier[J]. Quantum Coherence and Reality, 1994: 55-65. [138] BERRY M, DENNIS M. Natural superoscillations in monochromatic waves in D dimensions[J]. Journal of Physics A:Mathematical and Theoretical, 2008, 42(2): 022003. [139] DENNIS M R, HAMILTON A C, COURTIAL J. Superoscillation in speckle patterns[J]. Optics letters, 2008, 33(24): 2976-2978. doi: 10.1364/OL.33.002976 [140] HUANG K, YE H, TENG J, et al. Optimization-free superoscillatory lens using phase and amplitude masks: Optimization-free Super-oscillatory Lens[J]. Laser &Photonics Reviews, 2014, 8(1): 152-157. doi: 10.1002/lpor.201300123 [141] ROGERS E T F, QURAISHE S, ROGERS K S, et al. Far-field unlabeled super-resolution imaging with superoscillatory illumination[J]. APL Photonics, 2020, 5(6): 066107. doi: 10.1063/1.5144918 [142] FERREIRA P J S, KEMPF A. Superoscillations: faster than the Nyquist rate[J]. IEEE transactions on signal processing, 2006, 54(10): 3732-3740. doi: 10.1109/TSP.2006.877642 [143] ZALEVSKY Z, GARCÍA-MARTÍNEZ P, GARCÍA J. Superresolution using gray level coding[J]. Optics express, 2006, 14(12): 5178-5182. doi: 10.1364/OE.14.005178 [144] STERN A, JAVIDI B. Sampling in the light of Wigner distribution[J]. JOSA A, 2004, 21(3): 360-366. doi: 10.1364/JOSAA.21.000360 [145] STERN A, JAVIDI B. Sampling in the light of Wigner distribution: errata[J]. JOSA A, 2004, 21(10): 2038-2038. doi: 10.1364/JOSAA.21.002038 [146] PAPOULIS A. Pulse compression, fiber communications, and diffraction: a unified approach[J]. JOSA A, 1994, 11(1): 3-13. doi: 10.1364/JOSAA.11.000003 [147] STERN A. Sampling of linear canonical transformed signals[J]. Signal Processing, 2006, 86(7): 1421-1425. doi: 10.1016/j.sigpro.2005.07.031 [148] UNSER M, ZERUBIA J. A generalized sampling theory without band-limiting constraints[J]. IEEE Transactions on Circuits and Systems II:Analog and Digital Signal Processing, 1998, 45(8): 959-969. doi: 10.1109/82.718806 [149] ROMBERG J. Imaging via compressive sampling[J]. IEEE Signal Processing Magazine, 2008, 25(2): 14-20. doi: 10.1109/MSP.2007.914729 [150] CANDÈS E J, ROMBERG J, TAO T. Robust uncertainty principles: Exact signal reconstruction from highly incomplete frequency information[J]. IEEE Transactions on information theory, 2006, 52(2): 489-509. doi: 10.1109/TIT.2005.862083 [151] BRADY D J, CHOI K, MARKS D L, et al. Compressive holography[J]. Optics express, 2009, 17(15): 13040-13049. doi: 10.1364/OE.17.013040 [152] TAKHAR D, LASKA J N, WAKIN M B, et al.. A new compressive imaging camera architecture using optical-domain compression[C]//Computational Imaging IV: 6065. SPIE, 2006: 43-52. [153] GLASNER D, BAGON S, IRANI M. Super-resolution from a single image[C/OL]//2009 IEEE 12th International Conference on Computer Vision. Kyoto: IEEE, 2009: 349-356[2019-06-05]. http://ieeexplore.ieee.org/document/5459271/. [154] HUANG J B, SINGH A, AHUJA N. Single image super-resolution from transformed self-exemplars[C/OL]//2015 IEEE Conference on Computer Vision and Pattern Recognition (CVPR). Boston, MA, USA: IEEE, 2015: 5197-5206[2019-06-05]. http://ieeexplore.ieee.org/document/7299156/. [155] KWANG IN KIM, YOUNGHEE KWON. Single-Image Super-Resolution Using Sparse Regression and Natural Image Prior[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2010, 32(6): 1127-1133. doi: 10.1109/TPAMI.2010.25 [156] WANG D, FU T, BI G, et al. Long-Distance Sub-Diffraction High-Resolution Imaging Using Sparse Sampling[J]. Sensors, 2020, 20(11): 3116. doi: 10.3390/s20113116 [157] XIANG M, PAN A, ZHAO Y Y, et al.. Coherent synthetic aperture imaging for visible remote sensing via reflective Fourier ptychography[J]. Optics Letters, 2021, 46(1): 29-32. [158] BIONDI F. Recovery of partially corrupted SAR images by super-resolution based on spectrum extrapolation[J]. IEEE Geoscience and Remote Sensing Letters, 2016, 14(2): 139-143. [159] BHATTACHARJEE S, SUNDARESHAN M K. Mathematical extrapolation of image spectrum for constraint-set design and set-theoretic superresolution[J]. JOSA A, 2003, 20(8): 1516-1527. doi: 10.1364/JOSAA.20.001516 [160] ELAD M, DATSENKO D. Example-based regularization deployed to super-resolution reconstruction of a single image[J]. The Computer Journal, 2009, 52(1): 15-30. [161] BEVILACQUA M, ROUMY A, GUILLEMOT C, et al. Single-image super-resolution via linear mapping of interpolated self-examples[J]. IEEE Transactions on image processing, 2014, 23(12): 5334-5347. doi: 10.1109/TIP.2014.2364116 [162] DONG C, LOY C C, HE K, et al. Image super-resolution using deep convolutional networks[J]. IEEE transactions on pattern analysis and machine intelligence, 2015, 38(2): 295-307. [163] ZOU Y, ZHANG L, LIU C, et al. Super-resolution reconstruction of infrared images based on a convolutional neural network with skip connections[J]. Optics and Lasers in Engineering, 2021, 146: 106717. doi: 10.1016/j.optlaseng.2021.106717 [164] B. Wang, Y. Zou, L. Zhang, Y. Hu, H. Yan, C. Zuo, et al. Low-light-level image super-resolution reconstruction based on a multi-scale features extraction network[J]. Photonics, 2021, 8(8): 321. doi: 10.3390/PHOTONICS8080321 [165] WANG B, ZOU Y, ZHANG L, et al. Multimodal super-resolution reconstruction of infrared and visible images via deep learning[J]. Optics and Lasers in Engineering, 2022, 156: 107078. doi: 10.1016/j.optlaseng.2022.107078 [166] DONG C, LOY C C, HE K, et al. Image Super-Resolution Using Deep Convolutional Networks[J]. IEEE Transactions on Pattern Analysis and Machine Intelligence, 2016, 38(2): 295-307. doi: 10.1109/TPAMI.2015.2439281 [167] DONG C, LOY C C, HE K, et al.. Learning a Deep Convolutional Network for Image Super-Resolution[C/OL]//FLEET D, PAJDLA T, SCHIELE B, et al. Computer Vision – ECCV 2014. Cham: Springer International Publishing, 2014: 184-199.https://doi.org/10.1007/978-3-319-10593-2_13. [168] CHAKRABARTI A. A Neural Approach to Blind Motion Deblurring[C/OL]//LEIBE B, MATAS J, SEBE N, et al. Computer Vision – ECCV 2016. Cham: Springer International Publishing, 2016: 221-235. https://doi.org/10.1007/978-3-319-46487-9_14. [169] HE K, ZHANG X, REN S, et al.. Deep Residual Learning for Image Recognition[C/OL]//Proceedings of the IEEE Conference on Computer Vision and Pattern Recognition. 2016: 770-778[2022-11-20]. https://openaccess.thecvf.com/content_cvpr_2016/html/He_Deep_Residual_Learning_CVPR_2016_paper.html. [170] SHOCHER A, COHEN N, IRANI M. “Zero-Shot” Super-Resolution using Deep Internal Learning[J]. arXiv: 1712.06087 [cs, eess], 2017[2021-12-17]. http://arxiv.org/abs/1712.06087. [171] ZHANG K, VAN GOOL L, TIMOFTE R. Deep Unfolding Network for Image Super-Resolution[C/OL]//2020 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR). Seattle, WA, USA: IEEE, 2020: 3214-3223[2021-12-17]. https://ieeexplore.ieee.org/document/9157092/. [172] LUO Z, HUANG Y, LI S, et al.. End-to-end Alternating Optimization for Blind Super Resolution[J]. arXiv: 2105.06878 [cs], 2021[2021-10-22]. http://arxiv.org/abs/2105.06878. [173] Lim B, Son S, Kim H, et al.. Enhanced Deep Residual Networks for Single Image Super-Resolution: IEEE, 10.1109/CVPRW.2017.151[P]. 2017. [174] HE K, ZHANG X, REN S, et al.. Deep residual learning for image recognition[C]//Proceedings of the IEEE conference on computer vision and pattern recognition. 2016: 770-778. [175] RONNEBERGER O, FISCHER P, BROX T. U-net: Convolutional networks for biomedical image segmentation[C]//International Conference on Medical image computing and computer-assisted intervention. Springer, 2015: 234-241. [176] GANDELSMAN Y, SHOCHER A, IRANI M. “Double-DIP”: Unsupervised Image Decomposition via Coupled Deep-Image-Priors[C/OL]//2019 IEEE/CVF Conference on Computer Vision and Pattern Recognition (CVPR). Long Beach, CA, USA: IEEE, 2019: 11018-11027[2021-12-24]. https://ieeexplore.ieee.org/document/8954420/. [177] KIM S Y, SIM H, KIM M. KOALAnet: Blind Super-Resolution using Kernel-Oriented Adaptive Local Adjustment[C]. 10.48550/arXiv.2012.081032020. [178] ZHANG K, ZUO W, GU S, et al.. Learning Deep CNN Denoiser Prior for Image Restoration[C/OL]//2017 IEEE Conference on Computer Vision and Pattern Recognition (CVPR). Honolulu, HI: IEEE, 2017: 2808-2817[2022-04-27]. http://ieeexplore.ieee.org/document/8099783/. [179] TAO G, JI X, WANG W, et al.. Spectrum-to-Kernel Translation for Accurate Blind Image Super-Resolution[A]. Advances in Neural Information Processing Systems[C]. Curran Associates, Inc., 2021, 34: 22643-22654. [180] ZHANG L J, GU K, VAN GOOL S, L., et al.. Flow based kernel prior with application to blind super-resolution[C]. IEEE Conference on Computer Vision and Pattern Recognition (CVPR) . 2021: 10601–10610. [181] LI X, SUO J, ZHANG W, et al.. Universal and Flexible Optical Aberration Correction Using Deep-Prior Based Deconvolution[C/OL]//2021 IEEE/CVF International Conference on Computer Vision (ICCV). Montreal, QC, Canada: IEEE, 2021: 2593-2601[2022-04-26]. https://ieeexplore.ieee.org/document/9710104/. [182] CAI J, ZENG H, YONG H, et al.. Toward Real-World Single Image Super-Resolution: A New Benchmark and a New Model[C/OL]//2019 IEEE/CVF International Conference on Computer Vision (ICCV). Seoul, Korea (South): IEEE, 2019: 3086-3095[2021-10-22]. https://ieeexplore.ieee.org/document/9009805/. [183] WANG X, XIE L, DONG C, et al.. Real-ESRGAN: Training Real-World Blind Super-Resolution with Pure Synthetic Data[C/OL]//2021 IEEE/CVF International Conference on Computer Vision Workshops (ICCVW). Montreal, BC, Canada: IEEE, 2021: 1905-1914[2022-04-25]. https://ieeexplore.ieee.org/document/9607421/. [184] NAZERI K, THASARATHAN H, EBRAHIMI M. Edge-Informed Single Image Super-Resolution[J]. arXiv: 1909.05305 [cs, eess], 2019[2021-02-24]. http://arxiv.org/abs/1909.05305. [185] UNSER M, ALDROUBI A. A general sampling theory for nonideal acquisition devices[J]. IEEE Transactions on Signal Processing, 1994, 42(11): 2915-2925. doi: 10.1109/78.330352 [186] VANDEWALLE P, SÜSSTRUNK S, VETTERLI M. A Frequency Domain Approach to Registration of Aliased Images with Application to Super-resolution[J]. EURASIP Journal on Advances in Signal Processing, 2006, 2006(1)[2019-06-05]. https://asp-eurasipjournals.springeropen.com/articles/10.1155/ASP/2006/71459. [187] NGUYEN N, MILANFAR P. A wavelet-based interpolation-restoration method for superresolution (wavelet superresolution)[J]. Circuits Systems and Signal Processing, 2000, 19(4): 321-338. doi: 10.1007/BF01200891 [188] IRANI M, PELEG S. Improving resolution by image registration[J]. CVGIP:Graphical Models and Image Processing, 1991, 53(3): 231-239. doi: 10.1016/1049-9652(91)90045-L [189] CHEN J, LI Y, CAO L. Research on region selection super resolution restoration algorithm based on infrared micro-scanning optical imaging model[J]. Scientific Reports, 2021, 11(1): 1-8. doi: 10.1038/s41598-020-79139-8 [190] ZHANG X, HUANG W, XU M, et al. Super-resolution imaging for infrared micro-scanning optical system[J]. Optics express, 2019, 27(5): 7719-7737. doi: 10.1364/OE.27.007719 [191] DAI S sheng, LIU J song, XIANG H yan, et al. Super-resolution reconstruction of images based on uncontrollable microscanning and genetic algorithm[J]. Optoelectronics Letters, 2014, 10(4): 313-316. doi: 10.1007/s11801-014-4067-x [192] HUSZKA G, GIJS M A. Turning a normal microscope into a super-resolution instrument using a scanning microlens array[J]. Scientific reports, 2018, 8(1): 1-8. [193] GUNTURK B K, ALTUNBASAK Y, MERSEREAU R M. Super-resolution reconstruction of compressed video using transform-domain statistics[J]. IEEE Transactions on Image Processing, 2004, 13(1): 33-43. doi: 10.1109/TIP.2003.819221 [194] CABANSKI W A, BREITER R, MAUK K H, et al.. Miniaturized high-performance starring thermal imaging system[C]//Infrared Detectors and Focal Plane Arrays VI: International Society for Optics and Photonics, 2000: 208-219. [195] WANG B, ZUO C, SUN J, et al.. A computational super-resolution technique based on coded aperture imaging[C/OL]//PETRUCCELLI J C, TIAN L, PREZA C. Computational Imaging V. Online Only, United States: SPIE, 2020: 25[2020-10-13].https://www.spiedigitallibrary.org/conference-proceedings-of-spie/11396/2560579/A-computational-super-resolution-technique-based-on-coded-aperture-imaging/10.1117/12.2560579.full. [196] PAN GIGA. High-Resolution images panoramic photography GigaPixel images[EB/OL]. [2021-03-08]. http://gigapan.com/. [197] SAKO T, SEKIGUCHI T, SASAKI M, et al. MOA-cam3: a wide-field mosaic CCD camera for a gravitational microlensing survey in New Zealand[J]. Experimental Astronomy, 2008, 22(1): 51-66. [198] Gaia (spacecraft)[EB/OL]//Wikipedia. (2021-02-22)[2021-03-08]. https://en.wikipedia.org/w/index.php ? title = Gaia (spacecraft) & oldid = 1008206925. [199] VERA C. Rubin Observatory[EB/OL]//Wikipedia. (2021-03-05)[2021-03-08]. https://en.wikipedia.org/w/index.php ? title = Vera C. Rubin Observatory&oldid = 1010481905. [200] ZHAO G, ZHAO Y H, CHU Y Q, et al. LAMOST spectral survey—An overview[J]. Research in Astronomy and Astrophysics, 2012, 12(7): 723. doi: 10.1088/1674-4527/12/7/002 [201] ARGUS-IS[EB/OL]//Wikipedia. (2020-07-15)[2021-03-08]. https://en.wikipedia.org/w/index.php ? title = ARGUS-IS & oldid = 967762056. [202] WILBURN B, JOSHI N, VAISH V, et al. High-speed videography using a dense camera array[C/OL]//Proceedings of the 2004 IEEE Computer Society Conference on Computer Vision and Pattern Recognition, 2004. CVPR 2004.: 2. 2004: II-II. https://doi.org/10.1109/CVPR.2004.1315176. [203] WILBURN B, JOSHI N, VAISH V, et al. High Performance Imaging Using Large Camera Arrays[M]//ACM SIGGRAPH 2005 Papers. 2005: 765-776. [204] A 360 degree camera that sees in 3D (w/Video)[EB/OL]. [2021-03-08]. https://phys.org/news/2010-12-degree-camera-3d-video.html. [205] COGAL O, AKIN A, SEYID K, et al. A new omni-directional multi-camera system for high resolution surveillance[C]//Mobile Multimedia/Image Processing, Security, and Applications 2014: 9120. International Society for Optics and Photonics, 2014: 91200N. [206] LAW N M, FORS O, RATZLOFF J, et al. The Evryscope: design and performance of the first full-sky gigapixel-scale telescope[C]//Ground-based and Airborne Telescopes VI: 9906. International Society for Optics and Photonics, 2016: 99061M. [207] LAW N M, FORS O, RATZLOFF J, et al. Evryscope science: exploring the potential of all-sky gigapixel-scale telescopes[J]. Publications of the Astronomical Society of the Pacific, 2015, 127(949): 234. doi: 10.1086/680521 [208] BRADY D J, HAGEN N. Multiscale lens design[J]. Optics express, 2009, 17(13): 10659-10674. doi: 10.1364/OE.17.010659 [209] BRADY D J. Focus in multiscale imaging systems[C]. Computational Optical Sensing and Imaging, 2012: CM2B-1. [210] TREMBLAY E J, MARKS D L, BRADY D J, et al. Design and scaling of monocentric multiscale imagers[J]. Applied Optics, 2012, 51(20): 4691-4702. doi: 10.1364/AO.51.004691 [211] MARKS D L, BRADY D J. Close-up imaging using microcamera arrays for focal plane synthesis[J]. Optical Engineering, 2011, 50(3): 033205. doi: 10.1117/1.3554389 [212] MARKS D L, TREMBLAY E J, FORD J E, et al. Microcamera aperture scale in monocentric gigapixel cameras[J]. Applied Optics, 2011, 50(30): 5824-5833. doi: 10.1364/AO.50.005824 [213] MARKS D L, BRADY D J. Gigagon: a Monocentric Lens Design Imaging 40 Gigapixels[C/OL]//Imaging Systems (2010), paper ITuC2. Optica Publishing Group, 2010: ITuC2[2022-11-20]. https://opg.optica.org/abstract.cfm?uri=IS-2010-ITuC2. [214] SON H S, MARKS D L, HAHN J, et al. Design of a spherical focal surface using close-packed relay optics[J]. Optics Express, 2011, 19(17): 16132-16138. doi: 10.1364/OE.19.016132 [215] SON H S, MARKS D L, TREMBLAY E, et al.. A Multiscale, Wide Field, Gigapixel Camera[C/OL]//Imaging and Applied Optics (2011), paper JTuE2. Optica Publishing Group, 2011: JTuE2[2022-11-20]. https://opg.optica.org/abstract.cfm?uri=COSI-2011-JTuE2. [216] MARKS D L, LLULL P R, PHILLIPS Z, et al. Characterization of the AWARE 10 two-gigapixel wide-field-of-view visible imager[J]. Applied optics, 2014, 53(13): C54-C63. doi: 10.1364/AO.53.000C54 [217] LLULL P, BANGE L, PHILLIPS Z, et al. Characterization of the AWARE 40 wide-field-of-view visible imager[J]. Optica, 2015, 2(12): 1086-1089. doi: 10.1364/OPTICA.2.001086 [218] 小科普. 超振荡以及在光学中的应用, 即突破衍射极限[EB/OL]//知乎专栏. [2022-05-22]. https://zhuanlan.zhihu.com/p/88964582.Popular Science. Superoscillation and its application in optics: beyond the diffraction limit[EB/OL]. Zhihu. [2022-05-22]. https://zhuanlan.zhihu.com/p/88964582. [219] GOODMAN J W. Holography Viewed from the Perspective of the Light Field Camera[M/OL]//OSTEN W. Fringe 2013. Berlin, Heidelberg: Springer Berlin Heidelberg, 2014: 3-15[2022-05-16]. http://link.springer.com/10.1007/978-3-642-36359-7_1. [220] GAO P, YUAN C. Resolution enhancement of digital holographic microscopy via synthetic aperture: a review[J]. Light:Advanced Manufacturing, 2022, 3(1): 105-120. doi: 10.37188/lam.2022.006 [221] MICO V, ZALEVSKY Z, GARCÍA-MARTÍNEZ P, et al. Synthetic aperture superresolution with multiple off-axis holograms[J]. JOSA A, 2006, 23(12): 3162-3170. doi: 10.1364/JOSAA.23.003162 [222] MICO V, ZALEVSKY Z, GARCÍA-MARTÍNEZ P, et al. Superresolved imaging in digital holography by superposition of tilted wavefronts[J]. Applied Optics, 2006, 45(5): 822-828. doi: 10.1364/AO.45.000822 [223] GRANERO L, MICÓ V, ZALEVSKY Z, et al. Superresolution imaging method using phase-shifting digital lensless Fourier holography[J]. Optics Express, 2009, 17(17): 15008-15022. doi: 10.1364/OE.17.015008 [224] MICÓ V, FERREIRA C, GARCÍA J. Surpassing digital holography limits by lensless object scanning holography[J]. Optics Express, 2012, 20(9): 9382-9395. doi: 10.1364/OE.20.009382 [225] MICO V, ZALEVSKY Z, GARCÍA J. Common-path phase-shifting digital holographic microscopy: A way to quantitative phase imaging and superresolution[J]. Optics Communications, 2008, 281(17): 4273-4281. doi: 10.1016/j.optcom.2008.04.079 [226] MICÓ V, GARCÍA J. Common-path phase-shifting lensless holographic microscopy[J]. Optics Letters, 2010, 35(23): 3919-3921. doi: 10.1364/OL.35.003919 [227] MICÓ V, ZALEVSKY Z, GARCIA J. Superresolved common-path phase-shifting digital inline holographic microscopy using a spatial light modulator[J]. Optics Letters, 2012, 37(23): 4988-4990. doi: 10.1364/OL.37.004988 [228] CHOI W, FANG-YEN C, BADIZADEGAN K, et al. Tomographic phase microscopy[J]. Nature Methods, 2007, 4(9): 717-719. doi: 10.1038/nmeth1078 [229] Mirsky S K, Shaked N T. First experimental realization of six-pack holography and its application to dynamic synthetic aperture superresolution[J]. Optics Express, 2019, 27(19): 26708. [230] MICÓ V, ZALEVSKY Z. Superresolved digital in-line holographic microscopy for high-resolution lensless biological imaging[J]. Journal of Biomedical Optics, 2010, 15(4): 046027. doi: 10.1117/1.3481142 [231] SANZ M, PICAZO-BUENO J A, GARCÍA J, et al. Improved quantitative phase imaging in lensless microscopy by single-shot multi-wavelength illumination using a fast convergence algorithm[J]. Optics Express, 2015, 23(16): 21352-21365. doi: 10.1364/OE.23.021352 [232] PICAZO-BUENO J Á, ZALEVSKY Z, GARCÍA J, et al. Superresolved spatially multiplexed interferometric microscopy[J]. Optics Letters, 2017, 42(5): 927-930. doi: 10.1364/OL.42.000927 [233] MICO V, FERREIRA C, ZALEVSKY Z, et al. Spatially-multiplexed interferometric microscopy (SMIM): converting a standard microscope into a holographic one[J]. Optics Express, 2014, 22(12): 14929-14943. doi: 10.1364/OE.22.014929 [234] CHOWDHURY S, ELDRIDGE W J, WAX A, et al. Structured illumination multimodal 3D-resolved quantitative phase and fluorescence sub-diffraction microscopy[J]. Biomedical Optics Express, 2017, 8(5): 2496. doi: 10.1364/BOE.8.002496 [235] COTTE Y, TOY F, JOURDAIN P, et al. Marker-free phase nanoscopy[J]. Nature Photonics, 2013, 7(2): 113-117. doi: 10.1038/nphoton.2012.329 [236] GABAI H, SHAKED N T. Dual-channel low-coherence interferometry and its application to quantitative phase imaging of fingerprints[J]. Optics Express, 2012, 20(24): 26906. doi: 10.1364/OE.20.026906 [237] GIRSHOVITZ P, SHAKED N T. Doubling the field of view in off-axis low-coherence interferometric imaging[J]. Light:Science &Applications, 2014, 3(3): e151. doi: 10.1038/lsa.2014.32 [238] FRENKLACH I, GIRSHOVITZ P, SHAKED N T. Off-axis interferometric phase microscopy with tripled imaging area[J]. Optics Letters, 2014, 39(6): 1525. doi: 10.1364/OL.39.001525 [239] OU X, ZHENG G, YANG C. Embedded pupil function recovery for Fourier ptychographic microscopy[J]. Optics Express, 2014, 22(5): 4960. doi: 10.1364/OE.22.004960 [240] SUN J, CHEN Q, ZHANG Y, et al. Efficient positional misalignment correction method for Fourier ptychographic microscopy[J]. Biomedical Optics Express, 2016, 7(4): 1336. doi: 10.1364/BOE.7.001336 [241] DONG S, SHIRADKAR R, NANDA P, et al. Spectral multiplexing and coherent-state decomposition in Fourier ptychographic imaging[J]. Biomedical Optics Express, 2014, 5(6): 1757. doi: 10.1364/BOE.5.001757 [242] TIAN L, LI X, RAMCHANDRAN K, et al. Multiplexed coded illumination for Fourier Ptychography with an LED array microscope[J]. Biomedical Optics Express, 2014, 5(7): 2376-2389. doi: 10.1364/BOE.5.002376 [243] LI P, BATEY D J, EDO T B, et al. Separation of three-dimensional scattering effects in tilt-series Fourier ptychography[J]. Ultramicroscopy, 2015, 158: 1-7. doi: 10.1016/j.ultramic.2015.06.010 [244] TIAN L, WALLER L. 3D intensity and phase imaging from light field measurements in an LED array microscope[J]. Optica, 2015, 2(2): 104. doi: 10.1364/OPTICA.2.000104 [245] ZUO C, SUN J, LI J, et al. Wide-field high-resolution 3D microscopy with Fourier ptychographic diffraction tomography[J]. Optics and Lasers in Engineering, 2020, 128: 106003. doi: 10.1016/j.optlaseng.2020.106003 [246] BIAN L, SUO J, SITU G, et al. Content adaptive illumination for Fourier ptychography[J]. Optics Letters, 2014, 39(23): 6648-6651. doi: 10.1364/OL.39.006648 [247] HE X, LIU C, ZHU J. Single-shot Fourier ptychography based on diffractive beam splitting[J]. Optics Letters, 2018, 43(2): 214. doi: 10.1364/OL.43.000214 [248] LEE B, HONG J young, YOO D, et al. Single-shot phase retrieval via Fourier ptychographic microscopy[J]. Optica, 2018, 5(8): 976-983. doi: 10.1364/OPTICA.5.000976 [249] ZHENG G, SHEN C, JIANG S, et al. Concept, implementations and applications of Fourier ptychography[J]. Nature Reviews Physics, 2021, 3(3): 207-223. doi: 10.1038/s42254-021-00280-y [250] PAN A, ZUO C, YAO B. High-resolution and large field-of-view Fourier ptychographic microscopy and its applications in biomedicine[J]. Reports on Progress in Physics, 2020, 83(9): 096101. doi: 10.1088/1361-6633/aba6f0 [251] CUI X, LEE L M, HENG X, et al. Lensless high-resolution on-chip optofluidic microscopes for Caenorhabditis elegans and cell imaging[J]. Proceedings of the National Academy of Sciences, 2008, 105(31): 10670-10675. doi: 10.1073/pnas.0804612105 [252] XU W, JERICHO M H, MEINERTZHAGEN I A, et al. Digital in-line holography for biological applications[J]. Proceedings of the National Academy of Sciences, 2001, 98(20): 11301-11305. doi: 10.1073/pnas.191361398 [253] SU T wei, SEO S, ERLINGER A, et al. Towards Wireless Health: Lensless On-Chip Cytometry[J]. Optics and Photonics News, 2008, 19(12): 24-24. doi: 10.1364/OPN.19.12.000024 [254] ISIKMAN S, SEO S, SENCAN I, et al.. Lensfree cell holography on a chip: From holographic cell signatures to microscopic reconstruction[C/OL]//2009 IEEE LEOS Annual Meeting Conference Proceedings. 2009: 404-405.https://doi.org/10.1109/LEOS.2009.5343233. [255] ZHENG G, LEE S A, ANTEBI Y, et al. The ePetri dish, an on-chip cell imaging platform based on subpixel perspective sweeping microscopy (SPSM)[J]. Proceedings of the National Academy of Sciences, 2011, 108(41): 16889-16894. doi: 10.1073/pnas.1110681108 [256] SEO S, SU T W K, TSENG D, et al. Lensfree holographic imaging for on-chip cytometry and diagnostics[J]. Lab on a Chip, 2009, 9(6): 777-787. doi: 10.1039/B813943A [257] GREENBAUM A, LUO W, SU T W, et al. Imaging without lenses: achievements and remaining challenges of wide-field on-chip microscopy[J]. Nature Methods, 2012, 9(9): 889-895. doi: 10.1038/nmeth.2114 [258] ELSER V. Phase retrieval by iterated projections[J]. JOSA A, 2003, 20(1): 40-55. doi: 10.1364/JOSAA.20.000040 [259] GREENBAUM A, OZCAN A. Maskless imaging of dense samples using pixel super-resolution based multi-height lensfree on-chip microscopy[J]. Optics Express, 2012, 20(3): 3129-3143. doi: 10.1364/OE.20.003129 [260] ZHANG Y, PEDRINI G, OSTEN W, et al. Whole optical wave field reconstruction from double or multi in-line holograms by phase retrieval algorithm[J]. Optics Express, 2003, 11(24): 3234-3241. doi: 10.1364/OE.11.003234 [261] MUDANYALI O, TSENG D, OH C, et al. Compact, light-weight and cost-effective microscope based on lensless incoherent holography for telemedicine applications[J]. Lab on a Chip, 2010, 10(11): 1417-1428. doi: 10.1039/C000453G [262] LUO W, ZHANG Y, GÖRÖCS Z, et al. Propagation phasor approach for holographic image reconstruction[J]. Scientific Reports, 2016, 6(1): 22738. doi: 10.1038/srep22738 [263] ZUO C, SUN J, ZHANG J, et al. Lensless phase microscopy and diffraction tomography with multi-angle and multi-wavelength illuminations using a LED matrix[J]. Optics Express, 2015, 23(11): 14314. doi: 10.1364/OE.23.014314 [264] GREENBAUM A, ZHANG Y, FEIZI A, et al. Wide-field computational imaging of pathology slides using lens-free on-chip microscopy[J]. Science Translational Medicine, 2014, 6(267): 267ra175-267ra175. doi: 10.1126/scitranslmed.3009850 [265] ZHANG J, SUN J, CHEN Q, et al. Adaptive pixel-super-resolved lensfree in-line digital holography for wide-field on-chip microscopy[J]. Scientific Reports, 2017, 7(1): 11777. doi: 10.1038/s41598-017-11715-x [266] LUO W, ZHANG Y, FEIZI A, et al. Pixel super-resolution using wavelength scanning[J]. Light:Science &Applications, 2016, 5(4): e16060-e16060. doi: 10.1038/lsa.2016.60 [267] ZHANG J, CHEN Q, LI J, et al. Lensfree dynamic super-resolved phase imaging based on active micro-scanning[J]. Optics Letters, 2018, 43(15): 3714-3717. doi: 10.1364/OL.43.003714 [268] BISHARA W, SIKORA U, MUDANYALI O, et al. Holographic pixel super-resolution in portable lensless on-chip microscopy using a fiber-optic array[J]. Lab on a Chip, 2011, 11(7): 1276. doi: 10.1039/c0lc00684j [269] WU X, SUN J, ZHANG J, et al. Wavelength-scanning lensfree on-chip microscopy for wide-field pixel-super-resolved quantitative phase imaging[J]. Optics Letters, 2021, 46(9): 2023. doi: 10.1364/OL.421869 [270] LI J, CHEN Q, SUN J, et al. Optimal illumination pattern for transport-of-intensity quantitative phase microscopy[J]. Optics Express, 2018, 26(21): 27599. doi: 10.1364/OE.26.027599 [271] BULBUL A, VIJAYAKUMAR A, ROSEN J. Partial aperture imaging by systems with annular phase coded masks[J]. Optics Express, 2017, 25(26): 33315-33329. doi: 10.1364/OE.25.033315 [272] LI J, CHEN Q, ZHANG J, et al. Efficient quantitative phase microscopy using programmable annular LED illumination[J]. Biomedical Optics Express, 2017, 8(10): 4687-4705. doi: 10.1364/BOE.8.004687 [273] PAPOULIS A. A new algorithm in spectral analysis and band-limited extrapolation[J]. IEEE Transactions on Circuits and Systems, 1975, 22(9): 735-742. doi: 10.1109/TCS.1975.1084118 [274] GERCHBERG R, SAXTON W. A practical algorithm for the determination of the phase from image and diffraction plane pictures[J]. Optik (Jena) , 1972, 35: 237. [275] GERCHBERG R W. Phase determination from image and diffraction plane pictures in the electron microscope[J]. Optik, 1971, 34(3): 275-284. [276] DONOHO D L, JOHNSTONE I M, HOCH J C, et al. Maximum Entropy and the Nearly Black Object[J]. Journal of the Royal Statistical Society. Series B (Methodological) , 1992, 54(1): 41-81. doi: 10.1111/j.2517-6161.1992.tb01864.x [277] SHIEH H M, BYRNE C L. Image reconstruction from limited Fourier data[J]. Journal of the Optical Society of America A, 2006, 23(11): 2732. doi: 10.1364/JOSAA.23.002732 [278] SHIEH H M, BYRNE C L, FIDDY M A. Image reconstruction: a unifying model for resolution enhancement and data extrapolation. Tutorial[J]. JOSA A, 2006, 23(2): 258-266. doi: 10.1364/JOSAA.23.000258 [279] MARCHESINI S, HE H, CHAPMAN H N, et al. X-ray image reconstruction from a diffraction pattern alone[J]. Physical Review B, 2003, 68(14): 140101. doi: 10.1103/PhysRevB.68.140101 [280] SHIEH H M, HSU Y C, BYRNE C L, et al. Resolution enhancement of imaging small-scale portions in a compactly supported function[J]. Journal of the Optical Society of America A, 2010, 27(2): 141. doi: 10.1364/JOSAA.27.000141 [281] LATYCHEVSKAIA T, FINK H W. Resolution enhancement in digital holography by self-extrapolation of holograms[J]. Optics Express, 2013, 21(6): 7726-7733. doi: 10.1364/OE.21.007726 [282] HUANG Z, CAO L. Bicubic interpolation and extrapolation iteration method for high resolution digital holographic reconstruction[J]. Optics and Lasers in Engineering, 2020, 130: 106090. doi: 10.1016/j.optlaseng.2020.106090 [283] KOSAREV E L. Shannon’s superresolution limit for signal recovery[J]. Inverse Problems, 1990, 6(1): 55-76. doi: 10.1088/0266-5611/6/1/007 [284] NARIMANOV E. Resolution limit of label-free far-field microscopy[J]. Advanced Photonics, 2019, 1(05): 1. doi: 10.1117/1.AP.1.5.056003 [285] RADON J. On the determination of functions from their integral values along certain manifolds[J]. IEEE Transactions on Medical Imaging, 1986, 5(4): 170-176. doi: 10.1109/TMI.1986.4307775 [286] WOLF E. Three-dimensional structure determination of semi-transparent objects from holographic data[J]. Optics Communications, 1969, 1(4): 153-156. doi: 10.1016/0030-4018(69)90052-2 [287] CHARRIÈRE F, MARIAN A, MONTFORT F, et al. Cell refractive index tomography by digital holographic microscopy[J]. Optics Letters, 2006, 31(2): 178. doi: 10.1364/OL.31.000178 [288] CHARRIÈRE F, PAVILLON N, COLOMB T, et al. Living specimen tomography by digital holographic microscopy: morphometry of testate amoeba[J]. Optics Express, 2006, 14(16): 7005. doi: 10.1364/OE.14.007005 [289] SUNG Y, CHOI W, FANG-YEN C, et al. Optical diffraction tomography for high resolution live cell imaging[J]. Optics Express, 2009, 17(1): 266-277. doi: 10.1364/OE.17.000266 [290] KIM K, YOON H, DIEZ-SILVA M, et al. High-resolution three-dimensional imaging of red blood cells parasitized by Plasmodium falciparum and in situ hemozoin crystals using optical diffraction tomography[J]. Journal of Biomedical Optics, 2013, 19(1): 011005. doi: 10.1117/1.JBO.19.1.011005 [291] SCHERMELLEH L, CARLTON P M, HAASE S, et al.. Subdiffraction Multicolor Imaging of the Nuclear Periphery with 3D Structured Illumination Microscopy[J]. Science, 2008, 320(5881): 1332-1336. [292] LI J, CHEN Q, SUN J, et al. Three-dimensional tomographic microscopy technique with multi-frequency combination with partially coherent illuminations[J]. Biomedical Optics Express, 2018, 9(6): 2526-2542. doi: 10.1364/BOE.9.002526 [293] ZHOU S, LI J, SUN J, et al. Accelerated Fourier ptychographic diffraction tomography with sparse annular LED illuminations[J]. Journal of Biophotonics, 2022, 15(3): e202100272. doi: 10.1002/jbio.202100272 [294] ZHOU N, ZHOU N, LI J, et al. Single-exposure 3D label-free microscopy based on color-multiplexed intensity diffraction tomography[J]. Optics Letters, 2022, 47(4): 969-972. doi: 10.1364/OL.442171 [295] LIM J, LEE K, JIN K H, et al. Comparative study of iterative reconstruction algorithms for missing cone problems in optical diffraction tomography[J]. Optics Express, 2015, 23(13): 16933. doi: 10.1364/OE.23.016933 [296] HECHT B, SICK B, WILD U P, et al. Scanning near-field optical microscopy with aperture probes: Fundamentals and applications[J]. The Journal of Chemical Physics, 2000, 112(18): 7761-7774. doi: 10.1063/1.481382 [297] HAO XIANG, YANG QING, KUANG CUIFANG, et al. Optical Super-Resolution Imaging Based on Frequency Shift[J]. Acta Optica Sinica, 2021, 41(1): 0111001. doi: 10.3788/AOS202141.0111001 [298] WEI F, LU D, SHEN H, et al. Wide Field Super-Resolution Surface Imaging through Plasmonic Structured Illumination Microscopy[J]. Nano Letters, 2014, 14(8): 4634-4639. doi: 10.1021/nl501695c [299] WEI F, LIU Z. Plasmonic Structured Illumination Microscopy[J]. Nano Letters, 2010, 10(7): 2531-2536. doi: 10.1021/nl1011068 [300] ZHOU Z, LIU W, HE J, et al. Far-field super-resolution imaging by nonlinearly excited evanescent waves[J]. Advanced Photonics, 2021, 3(2): 025001. doi: 10.1117/1.AP.3.2.025001 [301] BARBASTATHIS G, OZCAN A, SITU G. On the use of deep learning for computational imaging[J]. Optica, 2019, 6(8): 921. doi: 10.1364/OPTICA.6.000921 [302] ZUO CHAO, FENG SHIJIE, ZHANG XIANGYU, et al. Deep Learning Based Computational Imaging: Status, Challenges, and Future[J]. Acta Optica Sinica, 2020, 40(1): 0111003. doi: 10.3788/AOS202040.0111003 [303] ZUO C, QIAN J, FENG S, et al. Deep learning in optical metrology: a review[J]. Light:Science &Applications, 2022, 11(1): 39. doi: 10.1038/s41377-022-00714-x [304] WANG H, RIVENSON Y, JIN Y, et al. Deep learning enables cross-modality super-resolution in fluorescence microscopy[J]. Nature Methods, 2019, 16(1): 103-110. doi: 10.1038/s41592-018-0239-0 [305] RIVENSON Y, GÖRÖCS Z, GÜNAYDIN H, et al. Deep learning microscopy[J]. Optica, 2017, 4(11): 1437-1443. doi: 10.1364/OPTICA.4.001437 [306] QIAO C, LI D, GUO Y, et al. Evaluation and development of deep neural networks for image super-resolution in optical microscopy[J]. Nature Methods, 2021, 18(2): 194-202. doi: 10.1038/s41592-020-01048-5 -
下载:
计量
- 文章访问数: 5346
- HTML全文浏览量: 1278
- PDF下载量: 2124
- 被引次数: 0
