Topological circuit: a playground for exotic topological physics
doi: 10.37188/CO.2021-0095
-
摘要: 在过去的十年里,材料的拓扑相变以及它们的奇异物理现象在固态电子学领域掀起了研究热潮。近些年来,人们开始在其他系统中重现和模拟电子体系中的各种拓扑现象,包括冷原子气体、离子阱、光子学、声子学、机械波和电路体系。在这些体系平台中,由电感电容所组成的拓扑电路因具有高度灵活的设计自由度、高性价比、易加工和易集成的独特优势而备受关注。除此之外,在拓扑电路中可以方便地设计具有任意长程耦合、非线性、非互易、增益等效应的拓扑模型,从而实现很多在电子体系和光学体系中难以实现的非线性、非阿贝尔和非厄米的拓扑相变材料。本文作为拓扑电路领域的第一篇综述,系统性地回顾了过去六年拓扑电路领域的主要进展,重点关注其理论建模、电路设计与实验测量,并对拓扑电路与电子和光学体系中的拓扑绝缘体着重进行讨论和区别。本综述涵盖了多种不同类型的拓扑电路,包括含有非平庸边界态、高阶拓扑角模式以及外尔粒子的厄米拓扑电路,拥有拓扑节线和节点态的高维拓扑电路,具有趋肤效应和由增益/衰减导致的非厄米拓扑电路,自感应拓扑边界态的非线性拓扑电路,以及具有非阿贝尔规范场效应的拓扑电路。Abstract: Exploring topological phases of matter and their exotic physics appeared as a rapidly growing field of study in solid-state electron systems in the past decade. In recent years, there has been a trend on the emulation of topological insulators/semimetals in many other systems, including ultracold quantum gases, trapped ions, photonic, acoustic, mechanical, and electrical circuit systems. Among these platforms, topological circuits made of simple capacitive and inductive circuit elements emerged as a very competitive platform because of its highly controllable degrees of freedom, lowercost, easy implementation, and great flexibility for integration. Owing to the unique advantages of electrical circuits such as arbitrary engineering of long-range hopping, convenient realization of nonlinear, nonreciprocal, and gain effects, highly flexible measurement, many of the nonlinear, non-abelian, and non-Hermitian physics can be potentially realized and investigated using the electrical circuit platform. In this review, we provide the first short overview of the main achievements of topological circuits developed in the past six years, primarily focusing on their theoretical modeling, circuit construction, experimental characterization, and their distinction from their counterparts in quantum electronics and photonics. The scope of this review covers a wide variety of topological circuits, including Hermitian topological circuits hosting nontrivial edge state, higher-order corner state, Weyl particles; higher dimensional topological circuits exhibiting nodal link and nodal knot states; non-Hermitian topological circuits showing skin effects, gain and loss induced nontrivial edge state; self-induced topological edge state in nonlinear topological circuit; topological circuit having non-Abelian gauge potential.
-
图 1 (a-b)具有π/2感应强度的Hofstadter电路模型中的自旋依赖的拓扑边界态[9]。(c)三维拓扑电路中受电路参数调控的拓扑节线态和外尔态[16]。(d-f)二维形式的Su–Schrieffer–Heeger拓扑电路[17]。
Figure 1. (a-b) Spin-dependent topological edge state in an electrical circuit mimicking π/2 flux Hofstadter model[9]. (c) 3D topological circuit with topological nodal line state and Weyl state controlled by the circuit parameters[16]. (d-f) Two-dimensional version of the Su–Schrieffer–Heeger model circuit[17].
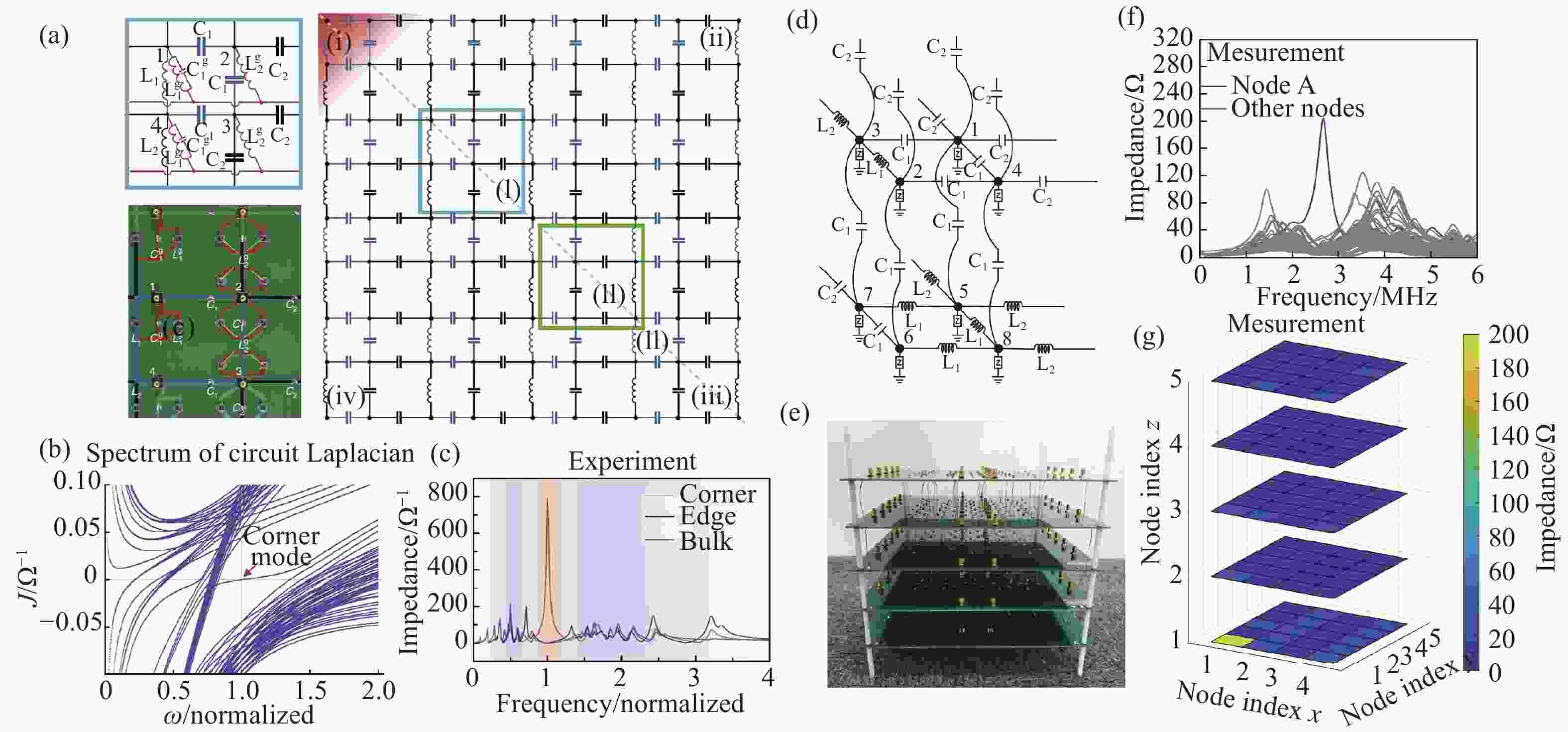
图 3 (a-c)二维高阶拓扑电路中由四极子动量导致的拓扑角模式的实验观测[33]。(d-e)三维高阶拓扑电路中由八极子动量所保护的零维拓扑角模式[34]。
Figure 3. (a-c) Experimental observation of a quadrupole moment induced corner state in a 2D electrical circuit[33]. (d-e) 3D topological circuit exhibiting 0D corner state topologically protected by octupole moment of the bulk circuit[34].
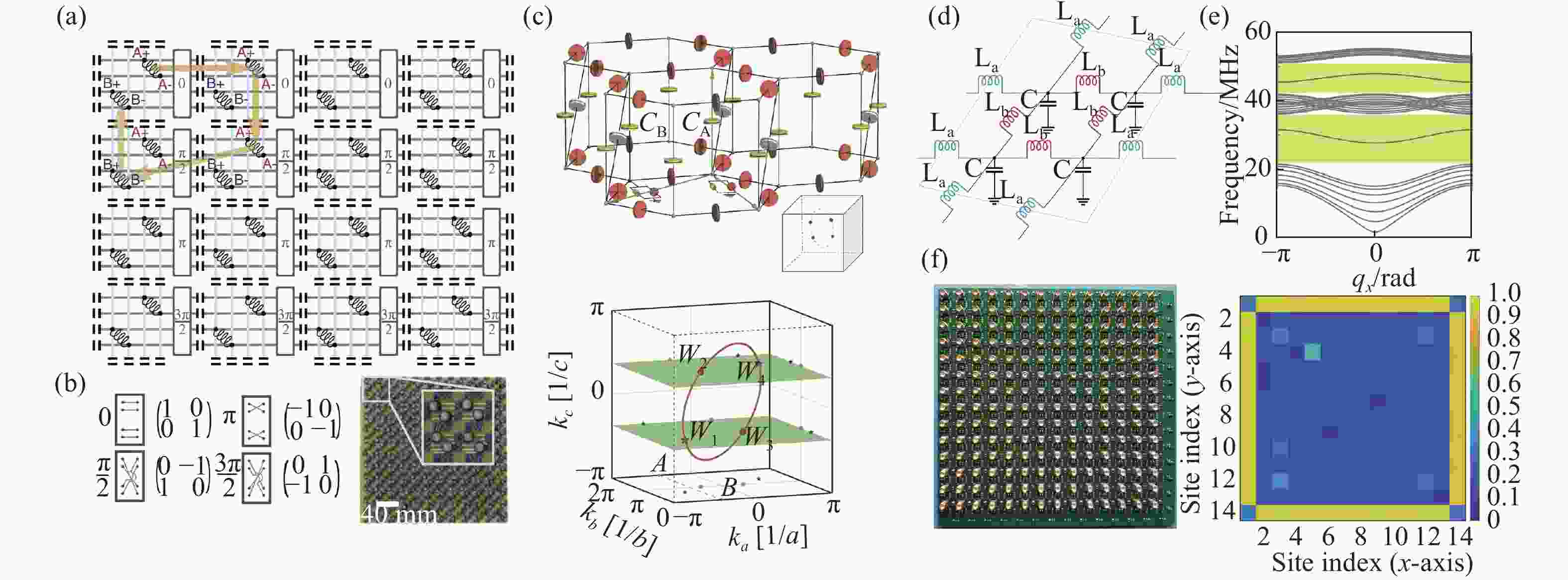
图 4 (a-c)具有二阶陈数的四维拓扑电路中的量子霍尔效应的实验验证[40]。(d,e)四维拓扑电路中的Seifert表面,即三维动量空间中二维表面引出的节线或者节点[41]。
Figure 4. (a-c) Experimental implementation of the first 4D quantum Hall model in electrical circuit characterized by a nonzero second Chern number[40]. (d,e) 4D circuit topological circuit exhibiting a Seifert surface, which is 2D surface with its boundary tracing out a link or knot in 3D momentum space[41].
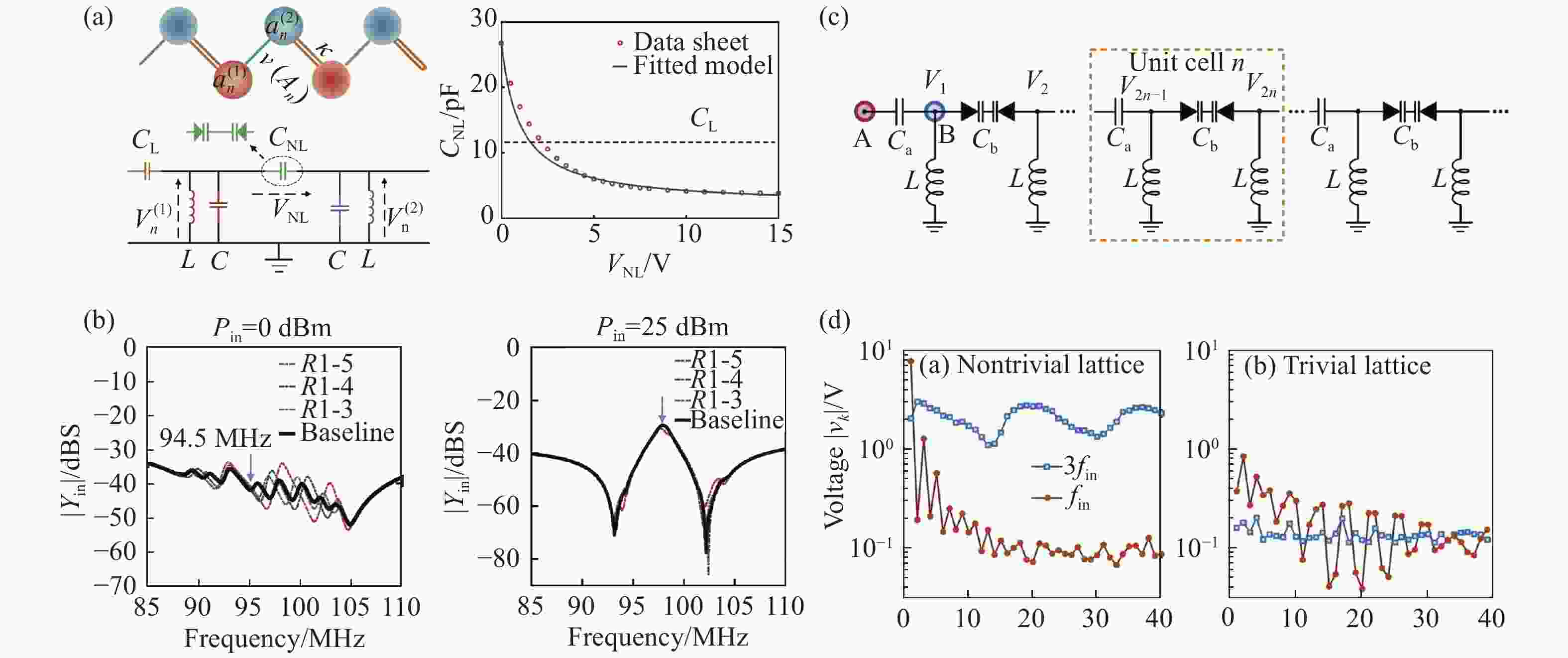
图 5 (a-c)由增益和衰减导致的拓扑相变的实验验证[48]。(d,e)具有非互易效应的非厄米拓扑电路中的趋肤效应的实验验证[13]。
Figure 5. (a-c) Experimental realization of a non-Hermitian electrical circuit whose nontrivial topology is induced by the introduction of gain and loss[48]. (d,e) Experimental observation of non-Hermitian skin effect in a variation of SSH topological circuit with nonreciprocity[13].
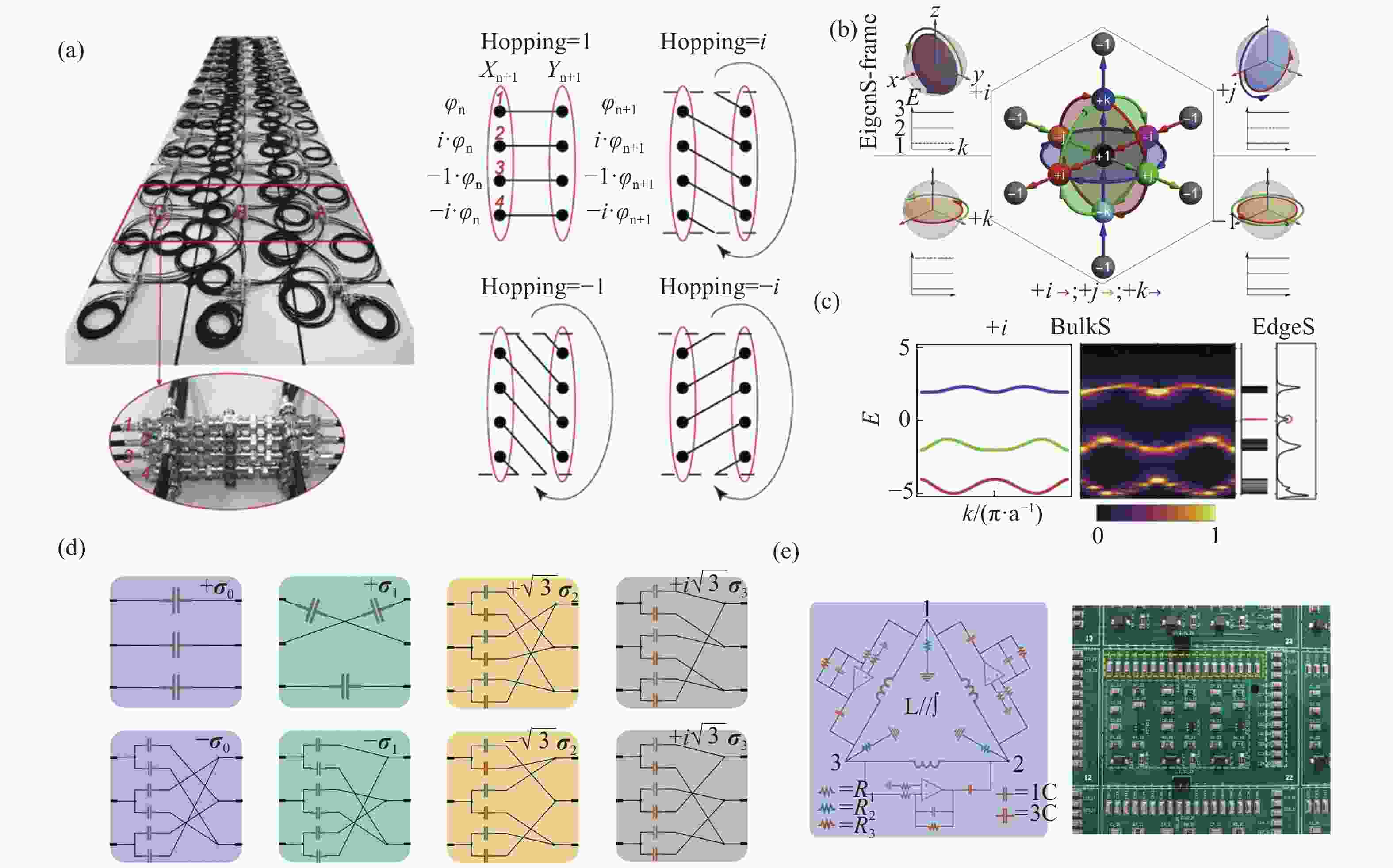
图 6 (a-c)具有编织耦合的传输线网络中的非阿贝尔拓扑电荷的实验观测[63]。(d,e)具有非阿贝尔规范场效应的拓扑电路中的Rashba-Dresselhaus自旋-轨道耦合现象的实验验证[65]。
Figure 6. (a-c) Experimental observation of non-Abelian topological charges in a transmission line network with braiding connectivity[63]. (d,e) Experimental demonstration of the Rashba-Dresselhaus spin-orbit interaction (SOI) in a passive electrical circuit with non-Abelian gauge fields[65].
-
[1] HASAN M Z, KANE C L. Colloquium: topological insulators[J]. Reviews of Modern Physics, 2010, 82(4): 3045-3067. doi: 10.1103/RevModPhys.82.3045 [2] QI X L, ZHANG SH CH. Topological insulators and superconductors[J]. Reviews of Modern Physics, 2011, 83(4): 1057-1110. doi: 10.1103/RevModPhys.83.1057 [3] BERNEVIG B A, HUGHES T L. Topological Insulators and Topological Superconductors[M]. Princeton: Princeton University Press, 2013. [4] HUGHES T L. Photonic topological insulators[B]. Taylor L. Hughes, 2013. [5] LU L, JOANNOPOULOS J D, SOLJAČIĆ M. Topological photonics[J]. Nature Photonics, 2014, 8(11): 821-829. doi: 10.1038/nphoton.2014.248 [6] MA G C, XIAO M, CHAN C T. Topological phases in acoustic and mechanical systems[J]. Nature Reviews Physics, 2019, 1(4): 281-294. doi: 10.1038/s42254-019-0030-x [7] DONG J K, JURICIC V, ROY B. Topolectric circuits: theory and construction[J]. arXiv preprint arXiv: 2008.11202, 2020. [8] LU L. Topology on a breadboard[J]. Nature Physics, 2018, 14(9): 875-877. doi: 10.1038/s41567-018-0235-4 [9] JIA N Y, OWENS C, SOMMER A, et al. Time- and site-resolved dynamics in a topological circuit[J]. Physical Review X, 2015, 5(2): 021031. doi: 10.1103/PhysRevX.5.021031 [10] LEE C H, IMHOF S, BERGER C, et al. Topolectrical circuits[J]. Communications Physics, 2018, 1: 39. doi: 10.1038/s42005-018-0035-2 [11] HOFMANN T, HELBIG T, LEE C H, et al. Chiral voltage propagation and calibration in a topolectrical chern circuit[J]. Physical Review Letters, 2019, 122(24): 247702. doi: 10.1103/PhysRevLett.122.247702 [12] ZHAO E H. Topological circuits of inductors and capacitors[J]. Annals of Physics, 2018, 399: 289-313. doi: 10.1016/j.aop.2018.10.006 [13] LIU SH, SHAO R W, MA SH J, et al. Non-Hermitian skin effect in a non-Hermitian electrical circuit[J]. Research, 2021, 2021: 5608038. [14] PETERSON C W, BENALCAZAR W A, HUGHES T L, et al. A quantized microwave quadrupole insulator with topologically protected corner states[J]. Nature, 2018, 555(7696): 346-350. doi: 10.1038/nature25777 [15] LU Y H, JIA N Y, SU L, et al. Probing the berry curvature and Fermi arcs of a Weyl circuit[J]. Physical Review B, 2019, 99(2): 020302. doi: 10.1103/PhysRevB.99.020302 [16] LUO K F, YU R, WENG H M. Topological nodal states in circuit lattice[J]. Research, 2018, 2018: 6793752. [17] LIU SH, GAO W L, ZHANG Q, et al. Topologically protected edge state in two-dimensional Su–Schrieffer–Heeger Circuit[J]. Research, 2019, 2019: 8609875. [18] XU S Y, BELOPOLSKI I, ALIDOUST N, et al. Discovery of a Weyl fermion semimetal and topological Fermi arcs[J]. Science, 2015, 349(6248): 613-617. doi: 10.1126/science.aaa9297 [19] HUANG L N, MCCORMICK T M, OCHI M, et al. Spectroscopic evidence for a type II Weyl semimetallic state in MoTe2[J]. Nature Materials, 2016, 15(11): 1155-1160. doi: 10.1038/nmat4685 [20] LU L, WANG ZH Y, YE D X, et al. Experimental observation of Weyl points[J]. Science, 2015, 349(6248): 622-624. doi: 10.1126/science.aaa9273 [21] YANG B, GUO Q H, TREMAIN B, et al. Ideal Weyl points and helicoid surface states in artificial photonic crystal structures[J]. Science, 2018, 359(6379): 1013-1016. doi: 10.1126/science.aaq1221 [22] OWENS C, LACHAPELLE A, SAXBERG B, et al. Quarter-flux Hofstadter lattice in a qubit-compatible microwave cavity array[J]. Physical Review A, 2018, 97(1): 013818. doi: 10.1103/PhysRevA.97.013818 [23] LI R J, LV B, TAO H B, et al. Ideal type-II Weyl points in topological circuits[J]. National Science Review, 2020: nwaa192. doi: 10.1093/nsr/nwaa192 [24] SOLUYANOV A A, GRESCH D, WANG ZH J, et al. Type-II Weyl semimetals[J]. Nature, 2015, 527(7579): 495-498. doi: 10.1038/nature15768 [25] JIANG J, LIU Z K, SUN Y, et al. Signature of type-II Weyl semimetal phase in MoTe2[J]. Nature Communications, 2017, 8: 13973. doi: 10.1038/ncomms13973 [26] NOH J, HUANG SH, LEYKAM D, et al. Experimental observation of optical Weyl points and Fermi arc-like surface states[J]. Nature Physics, 2017, 13(6): 611-617. doi: 10.1038/nphys4072 [27] YANG B, GUO Q H, TREMAIN B. Direct observation of topological surface-state arcs in photonic metamaterials[J]. Nature Communications, 2017, 8: 97. doi: 10.1038/s41467-017-00134-1 [28] RAFI-UL-ISLAM SM, SIU BZ, SUN CH, et al. Realization of Weyl semimetal phases in topoelectrical circuits[J]. New Journal of Physics, 2020, 22: 023025. doi: 10.1088/1367-2630/ab6eaf [29] RAFI-UI-ISLAM SM, SIU Z B, JALI L M. Topoelectrical circuit realization of a Weyl semimetal heterojunction[J]. Communication Physics, 2020, 3: 72. [30] BENALCAZAR W A, BERNEVIG B A, HUGHES T L. Quantized electric multipole insulators[J]. Science, 2017, 357(6346): 61-66. doi: 10.1126/science.aah6442 [31] LANGBEHN J, PENG Y, TRIFUNOVIC L, et al. Reflection-symmetric second-order topological insulators and superconductors[J]. Physical Review Letters, 2017, 119(24): 246401. doi: 10.1103/PhysRevLett.119.246401 [32] KHALAF E. Higher-order topological insulators and superconductors protected by inversion symmetry[J]. Physical Review B, 2018, 97(20): 205136. doi: 10.1103/PhysRevB.97.205136 [33] IMHOF S, BERGER C, BAYER F, et al. Topoelectrical-circuit realization of topological corner modes[J]. Nature Physics, 2018, 14(9): 925-929. doi: 10.1038/s41567-018-0246-1 [34] LIU SH, MA SH J, ZHANG L, et al. Octupole corner state in a three-dimensional topological circuit[J]. Light:Science &Applications, 2020, 9: 145. [35] BAO J CH, ZOU D Y, ZHANG W X, et al. Topoelectrical circuit octupole insulator with topologically protected corner states[J]. Physical Review B, 2019, 100(200): 201406. [36] EZAWA M. Braiding of Majorana-like corner states in electric circuits and its non-Hermitian generalization[J]. Physical Review B, 2019, 100(4): 045407. doi: 10.1103/PhysRevB.100.045407 [37] CHIU C K, TEO J C Y, SCHNYDER A P, et al. Classification of topological quantum matter with symmetries[J]. Review Modern Physics, 2016, 88(3): 035005. doi: 10.1103/RevModPhys.88.035005 [38] PRICE H M, ZILBERBERG O, OZAWA T, et al. Four-dimensional quantum hall effect with ultracold atoms[J]. Physical Review Letters, 2015, 115(19): 195303. doi: 10.1103/PhysRevLett.115.195303 [39] OZAWA T, PRICE H M, GOLDMAN N, et al. Synthetic dimensions in integrated photonics: from optical isolation to four-dimensional quantum Hall physics[J]. Physical Review A, 2016, 93(4): 043827. doi: 10.1103/PhysRevA.93.043827 [40] WANG Y, PRICE H M, ZHANG B L, et al. Circuit implementation of a four-dimensional topological insulator[J]. Nature Communications, 2020, 11: 2356. doi: 10.1038/s41467-020-15940-3 [41] LI L H, LEE C H, GONG J B. Boundary states of 4D topological matter: emergence and full 3D-imaging of nodal Seifert surfaces[J]. Communications Physics, 2019, 2: 135. doi: 10.1038/s42005-019-0235-4 [42] TAKATA K, NOTOMI M. Photonic topological insulating phase induced solely by gain and loss[J]. Physical Review Letters, 2018, 121(21): 213902. doi: 10.1103/PhysRevLett.121.213902 [43] YAO SH Y, WANG ZH. Edge states and topological invariants of non-hermitian systems[J]. Physical Review Letters, 2018, 121(8): 086803. doi: 10.1103/PhysRevLett.121.086803 [44] SHEN H T, ZHEN B, FU L. Topological band theory for non-Hermitian Hamiltonians[J]. Physical Review Letters, 2018, 120(14): 146402. doi: 10.1103/PhysRevLett.120.146402 [45] KAWABATA K, HIGASHIKAWA S, GONG Z P, et al. Topological unification of time-reversal and particle-hole symmetries in non-Hermitian physics[J]. Nature Communications, 2019, 10: 297. doi: 10.1038/s41467-018-08254-y [46] YAO SH Y, SONG F, WANG ZH. Non-hermitian chern bands[J]. Physical Review Letters, 2018, 121(12): 136802. [47] YOKOMIZO K, MURAKAMI S. Non-bloch band theory of non-Hermitian systems[J]. Physical Review Letters, 2019, 123(6): 066404. doi: 10.1103/PhysRevLett.123.066404 [48] LIU SH, MA SH J, YANG CH, et al. Gain-and loss-induced topological insulating phase in a non-Hermitian electrical circuit[J]. Physical Review Applied, 2020, 13(1): 014047. doi: 10.1103/PhysRevApplied.13.014047 [49] HELBIG T, HOFMANN T, IMHOF M, et al. Generalized bulk–boundary correspondence in non-Hermitian topolectrical circuits[J]. Nature Physics, 2020, 16(7): 747-750. doi: 10.1038/s41567-020-0922-9 [50] EZAWA M. Non-Hermitian boundary and interface states in nonreciprocal higher-order topological metals and electrical circuits[J]. Physical Review B, 2019, 99(12): 121411. doi: 10.1103/PhysRevB.99.121411 [51] EZAWA M. Electric circuits for non-Hermitian Chern insulators[J]. Physical Review B, 2019, 100(8): 081401. doi: 10.1103/PhysRevB.100.081401 [52] LUO K F, FENG J J, ZHAO Y X, et al.. Nodal manifolds bounded by exceptional points on non-hermitian honeycomb lattices and electrical-circuit realizations[J]. arXiv preprint arXiv: 1810.09231, 2018. [53] WU Q SH, SOLUYANOV A A, BZDUŠEK T. Non-Abelian band topology in noninteracting metals[J]. Science, 2019, 365(6459): 1273-1277. doi: 10.1126/science.aau8740 [54] TIWARI A, BZDUŠEK T. Non-Abelian topology of nodal-line rings in PT-symmetric systems[J]. Physical Review B, 2020, 101(19): 195130. doi: 10.1103/PhysRevB.101.195130 [55] YANG E CH, YANG B, YOU O B, et al. Observation of non-Abelian nodal links in photonics[J]. Physical Review Letters, 2020, 125(3): 033901. doi: 10.1103/PhysRevLett.125.033901 [56] JACOB A, ÖHBERG P, JUZELIŪNAS G, et al. Cold atom dynamics in non-Abelian gauge fields[J]. Applied Physics B, 2007, 89(4): 439-445. doi: 10.1007/s00340-007-2865-6 [57] STERN A, LINDNER N H. Topological quantum computation—from basic concepts to first experiments[J]. Science, 2013, 339(6124): 1179-1184. doi: 10.1126/science.1231473 [58] WU ZH, ZHANG L, SUN W, et al. Realization of two-dimensional spin-orbit coupling for Bose-Einstein condensates[J]. Science, 2016, 354(6308): 83-88. doi: 10.1126/science.aaf6689 [59] CHEN Y T, ZHANG R Y, XIONG ZH F, et al. Non-Abelian gauge field optics[J]. Nature Communications, 2019, 10: 3125. doi: 10.1038/s41467-019-10974-8 [60] YANG Y, PENG CH, ZHU D, et al. Synthesis and observation of non-Abelian gauge fields in real space[J]. Science, 2019, 365(6457): 1021-1025. doi: 10.1126/science.aay3183 [61] RECHCIŃSKA K, KRÓL M, MAZUR R, et al. Engineering spin-orbit synthetic Hamiltonians in liquid-crystal optical cavities[J]. Science, 2019, 366(6466): 727-730. doi: 10.1126/science.aay4182 [62] GIANFRATE A, BLEU O, DOMINICI L, et al. Measurement of the quantum geometric tensor and of the anomalous Hall drift[J]. Nature, 2020, 578(7795): 381-385. doi: 10.1038/s41586-020-1989-2 [63] GUO Q H, JIANG T SH, ZHANG R Y, et al.. Experimental observation of non-Abelian topological charges and bulk-edge correspondence[J]. arXiv preprint arXiv: 2008.06100, 2020. [64] SONG Z Y, WU T Y, WU W Q, et al.. Experimental realization of non-Abelian gauge potentials and topological Chern state in circuit system[J]. arXiv preprint arXiv: 2009.04870, 2020. [65] DRESSELHAUS G. Spin-orbit coupling effects in zinc blende structures[J]. Physical Review, 1955, 100(2): 580-586. doi: 10.1103/PhysRev.100.580 [66] EZAWA M. Non-Abelian braiding of Majorana-like edge states and topological quantum computations in electric circuits[J]. Physical Review B, 2020, 102(7): 075424. doi: 10.1103/PhysRevB.102.075424 [67] NAYAK C, SIMON S H, STERN A, et al. Non-Abelian anyons and topological quantum computation[J]. Reviews of Modern Physics, 2008, 80(3): 1083-1159. doi: 10.1103/RevModPhys.80.1083 [68] HADAD Y, SORIC J C, KHANIKAEV A B, et al. Self-induced topological protection in nonlinear circuit arrays[J]. Nature Electronics, 2018, 1(3): 178-182. doi: 10.1038/s41928-018-0042-z [69] HADAD Y, VITELLI V, ALU A, et al. Solitons and propagating domain walls in topological resonator arrays[J]. ACS Photonics, 2017, 4(8): 1974-1979. doi: 10.1021/acsphotonics.7b00303 [70] WANG Y, LANG L J, LEE C H, et al. Topologically enhanced harmonic generation in a nonlinear transmission line metamaterial[J]. Nature Communications, 2019, 10: 1102. doi: 10.1038/s41467-019-08966-9 [71] HIROTA R, SUZUKI K. Theoretical and experimental studies of lattice solitons in nonlinear lumped networks[J]. Proceedings of the IEEE, 1973, 61(10): 1483-1491. doi: 10.1109/PROC.1973.9297 [72] NAGASHIMA H, AMAGISHI Y. Experiment on solitons in the dissipative Toda lattice using nonlinear transmission line[J]. Journal of the Physical Society of Japan, 1979, 47(6): 2021-2027. doi: 10.1143/JPSJ.47.2021 [73] MUROYA K, WATANABE S. Experiment on soliton in inhomogeneous electric circuit. I. Dissipative case[J]. Journal of the Physical Society of Japan, 1981, 50(9): 3159-3165. doi: 10.1143/JPSJ.50.3159 [74] MUROYA K, SAITOH N, WATANABE S. Experiment on lattice soliton by nonlinear LC Circuit—observation of a dark soliton[J]. Journal of the Physical Society of Japan, 1982, 51(3): 1024-1029. doi: 10.1143/JPSJ.51.1024 [75] KUUSELA T, HIETARINTA J, KOKKO K, et al. Soliton experiments in a nonlinear electrical transmission line[J]. European Journal of Physics, 1987, 8(1): 27-33. doi: 10.1088/0143-0807/8/1/007 [76] LEE C H, SUTRISNO A, HOFMANN T, et al. Imaging nodal knots in momentum space through topolectrical circuits[J]. Nature Communications, 2020, 11: 4385. doi: 10.1038/s41467-020-17716-1 [77] ZHANG W X, ZOU D Y, BAO J CH, et al. Topolectrical-circuit realization of a four-dimensional hexadecapole insulator[J]. Physical Review B, 2020, 102(10): 100102. doi: 10.1103/PhysRevB.102.100102 [78] HOFMANN T, HELBIG T, SCHINDLER F, et al. Reciprocal skin effect and its realization in a topolectrical circuit[J]. Physical Review Research, 2020, 2(2): 023265. doi: 10.1103/PhysRevResearch.2.023265 -





 下载:
下载: