-
摘要:
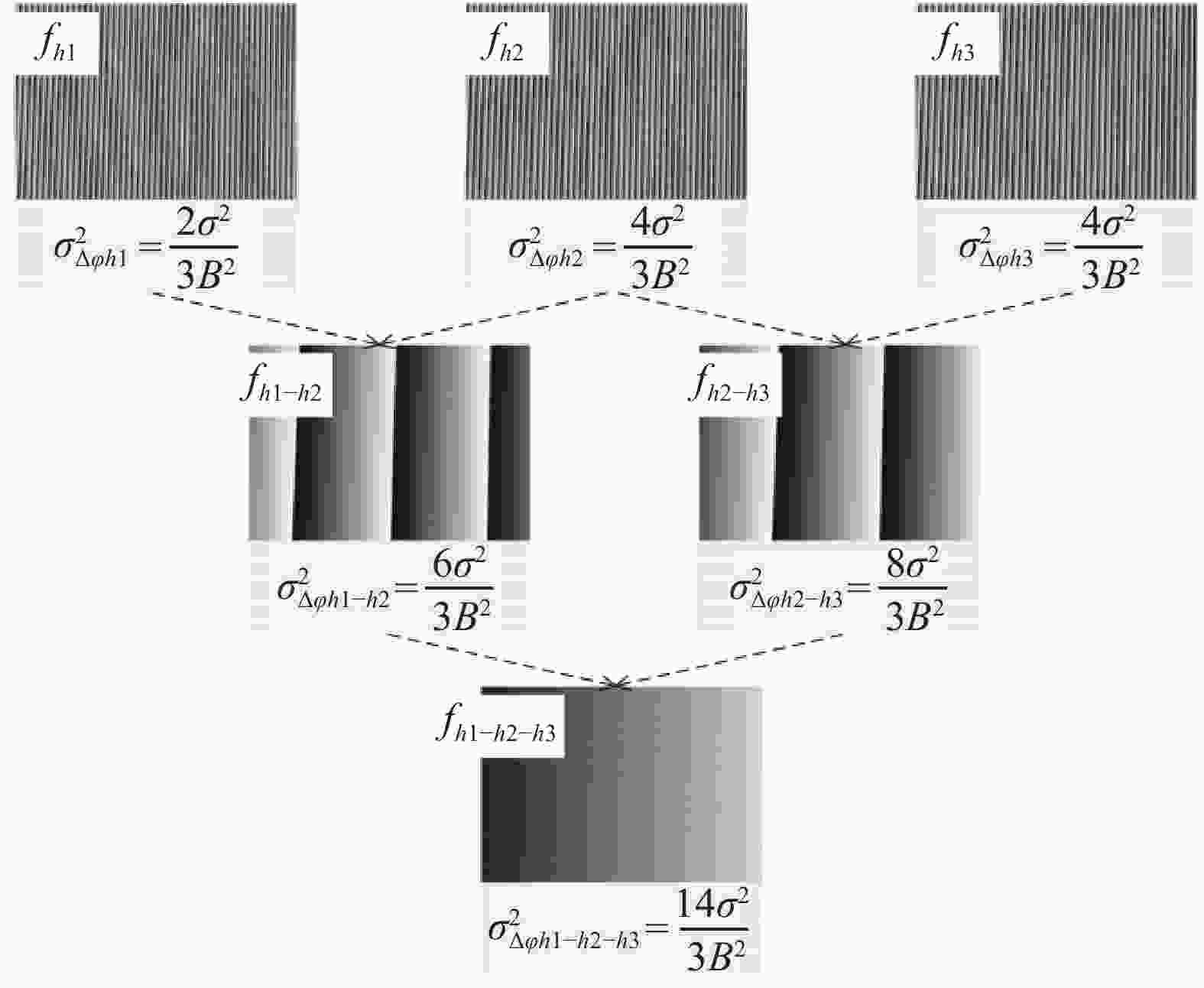
在相移轮廓术中,非标准相移轮廓术结合时域相位展开算法仅需较少的条纹图案,因而具备较高的测量效率。鉴于条纹频率对测量精度有显著影响,本文分析了非标准相移轮廓术的时域相位展开中的相位误差,并进一步评估其可靠性。研究发现,相位展开的可靠性与条纹频率分配密切相关。据此,本文引入了一种最优条纹频率分配策略。基于该策略,本文对非标准相移轮廓术的不同频率组合进行了对比实验,实验结果显示,相比于3
f h 1+2f h 2+2f h 3外差法的非最优频率组合,本文提出的频率组合的平均错误率降低了62.96%;相比于2f h +2f m +3f l 分层法的非最优频率组合,本文提出的频率组合的平均错误率降低了49.23%。Abstract:In phase-shifting profilometry, the non-standard phase-shifting profilometry combined with the temporal phase unwrapping algorithm requires fewer fringe patterns, thereby achieving higher measurement efficiency. Given that fringe frequency has a significant effect on measurement accuracy, this paper analyzes phase errors in the temporal phase unwrapping of the non-standard phase-shifting profilometry and further evaluates its reliability. It is found that the reliability of phase unwrapping is closely related to the allocation of fringe frequencies. Consequently, an optimal fringe frequency allocation strategy is proposed. Based on this strategy, this paper conducts comparative experiments on different frequency combinations of non-standard phase-shifting profilometry, and the experimental results show that compared with the non-optimal frequency combinations of the 3
f h 1+2f h 2+2f h 3 heterodyne algorithm, the average error rate of the frequency combination proposed in this paper is reduced by 62.96%; compared with the non-optimal frequency combinations of the 2f h +2f m +3f l hierarchical algorithm, the average error rate of the frequency combination proposed in this paper is reduced by 49.23%. -
图 1 双频与三频TPU算法的重建结果对比。 (a) 3-step和{fh=64, fm=8, fl=1};(b) 3-step和{fh=64, fl=1};(c) 3fh+2fm+2fl和{fh=64, fm=8, fl=1};(d) 3fh+2fl和{fh=64, fl=1};
Figure 1. Comparison of reconstruction results between dual-frequency and triple-frequency TPU algorithms. (a) 3-step and {fh=64, fm=8, fl=1};(b) 3-step and {fh=64, fl=1};(c) 3fh+2fm+2fl and {fh=64, fm=8, fl=1};(d) 3fh+2fl and {fh=64, fl=1};
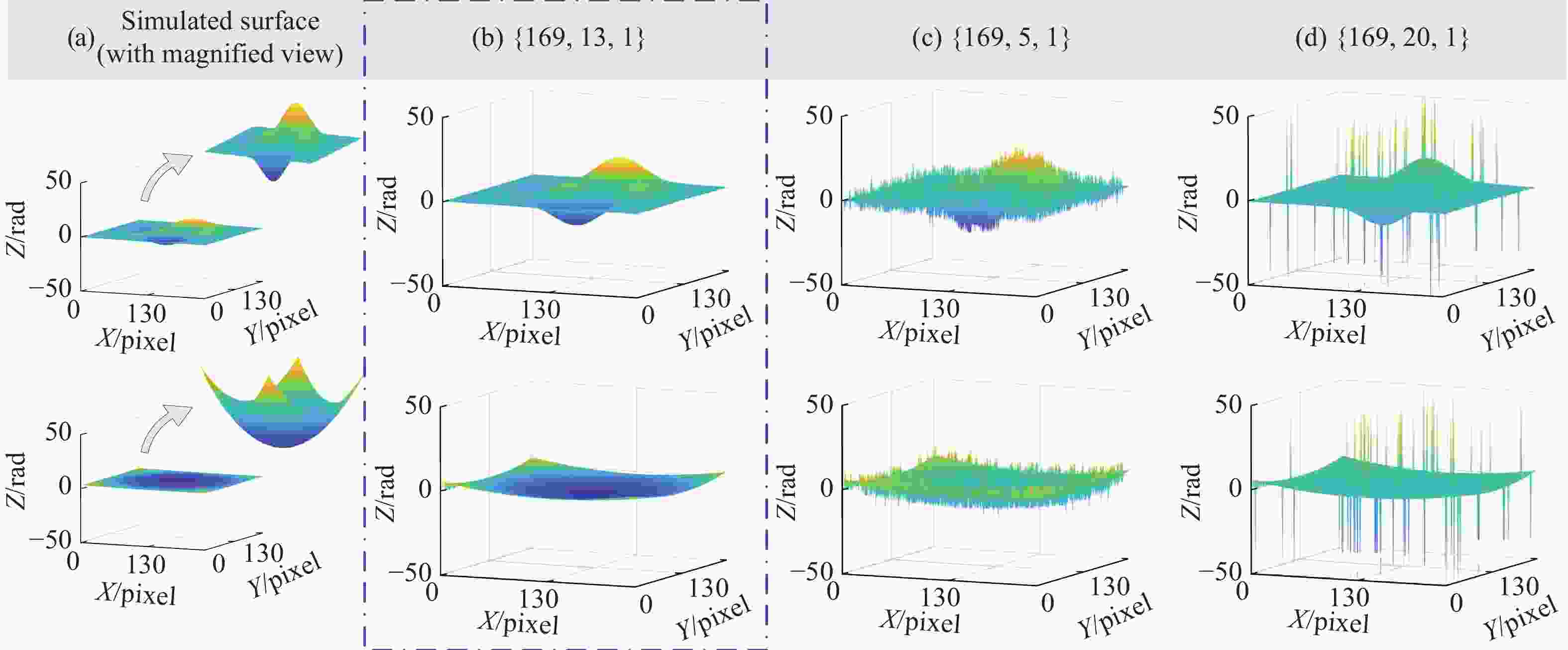
图 3 仿真曲面的三维重建结果。(a) 仿真曲面(含放大图);(b) { fh=169, fm=13, fl=1};(c) { fh=169, fm=5, fl=1};(d) { fh=169, fm=20, fl=1}
Figure 3. The 3D reconstruction results of the simulated surface. (a) simulated surface (with magnified view); (b) { fh=169, fm=13, fl=1}; (c) { fh=169, fm=5, fl=1}; (d) { fh=169, fm=20, fl=1}
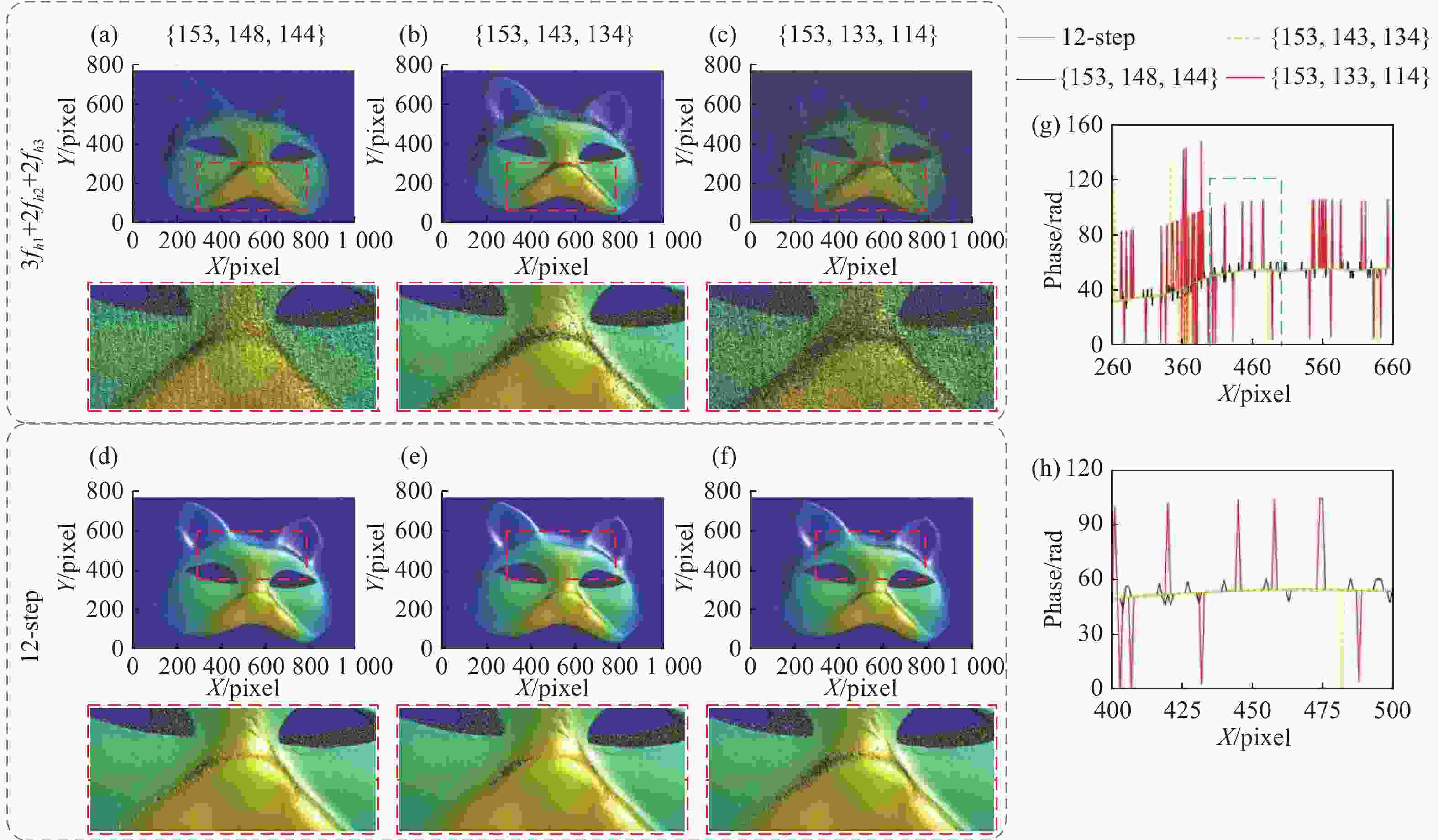
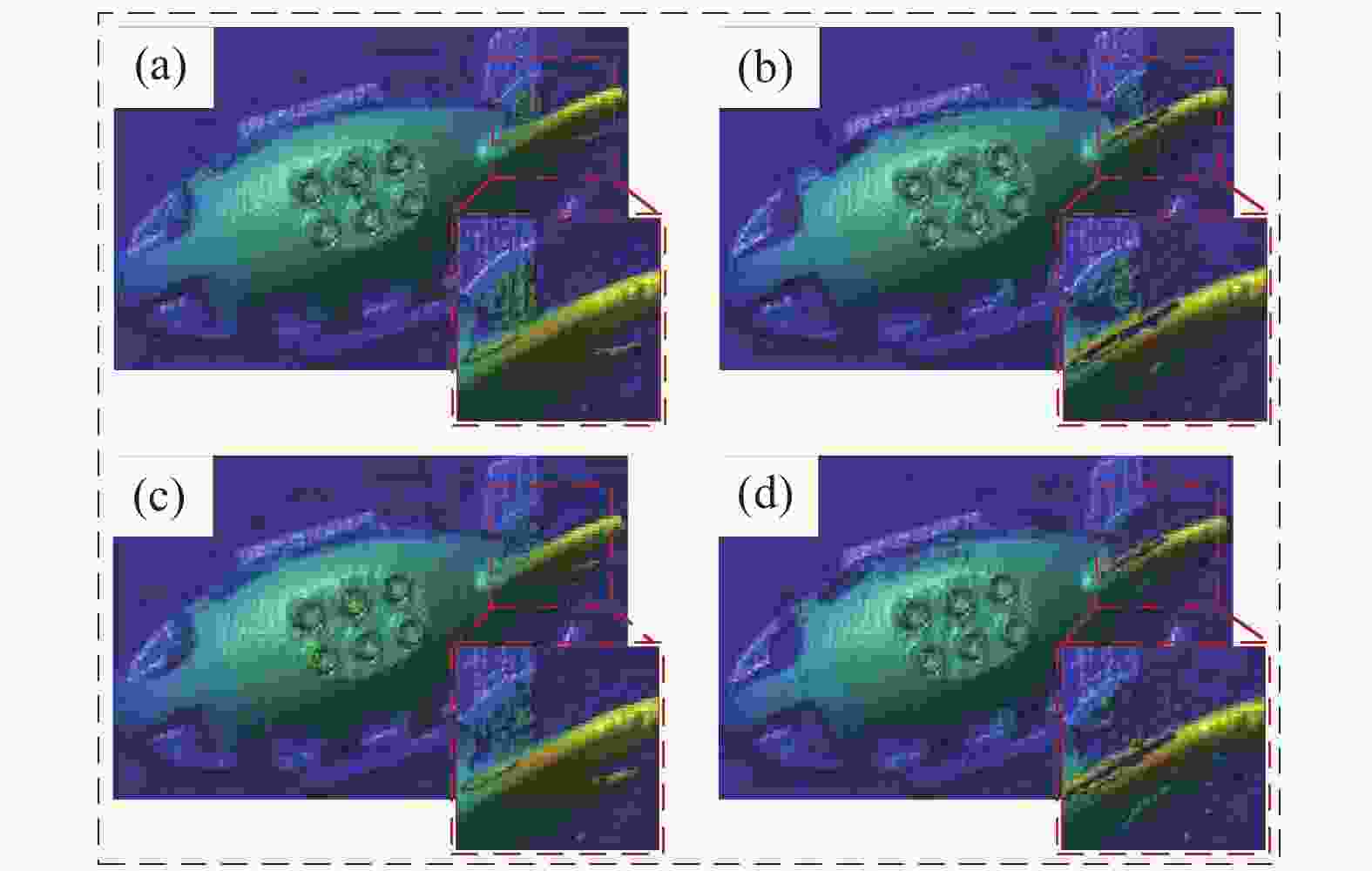
图 4 3fh1+2fh2+2fh3外差TPU算法的重建结果比较。 (a) 3fh1+2fh2+2fh3和{fh1=153, fh2=148, fh3=144};(b) 3fh1+2fh2+2fh3和{fh1=153, fh2=143, fh3=134};(c) 3fh1+2fh2+2fh3和{fh1=153, fh2=133, fh3=114};(d) 12-step和{fh1=153, fh2=148, fh3=144};(e) 12-step和{fh1=fh1=153, fh2=143, fh3=134};(f) 12-step和{fh1=153, fh2=133, fh3=114};(g) 横截面对比(第600行);(h)为(g)的放大视图
Figure 4. Comparison of reconstruction results based on 3fh1+2fh2+2fh3 heterodyne TPU. (a) 3fh1+2fh2+2fh3 and {fh1=153, fh2=148, fh3=144}; (b) 3fh1+2fh2+2fh3 and {fh1=153, fh2=143, fh3=134}; (c) 3fh1+2fh2+2fh3 and {fh1=153, fh2=133, fh3=114}; (d) 12-step and {fh1=153, fh2=148, fh3=144}; (e) 12-step and {fh1=153, fh2=143, fh3=134}; (f) 12-step and {fh1=153, fh2=133, fh3=114}; (g) cross-sectional comparison (line 600); (h) larger view of (g)
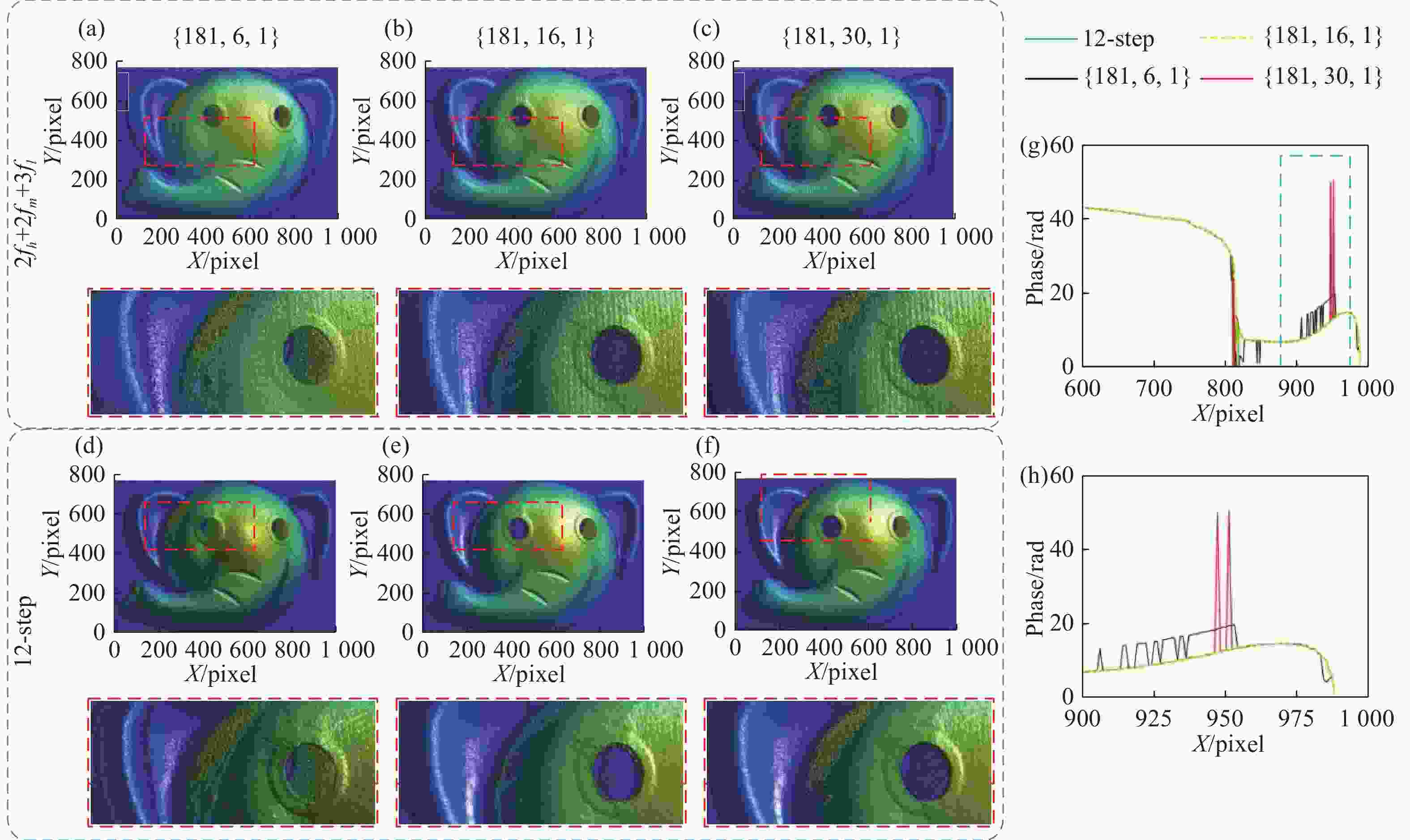
图 5 基于2fh+2fm+3fl分层TPU算法的重建结果比较。(a) 2fh+2fm+3fl和{fh=181, fm=6, fl=1};(b) 2fh+2fm+3fl和{ fh=181, fm=16, fl=1};(c) 2fh+2fm+3fl和{fh=181, fm=30, fl=1};(d) 12-step和{fh=181, fm=6, fl=1};(e) 12-step和{ fh=181, fm=16, fl=1}(f) 12-step和{fh=181, fm=30, fl=1};(g) 横截面对比(第170行);(h)为(g)的放大视图
Figure 5. Comparison of reconstruction results based on 2fh+2fm+3fl hierarchical TPU. (a) 2fh+2fm+3fl and {fh=181, fm=6, fl=1}; (b) 2fh+2fm+3fl and {fh=181, fm=16, fl=1}; (c) 2fh+2fm+3fl and {fh=181, fm=30, fl=1}; (d) 12-step and {fh=181, fm=6, fl=1}; (e)12-step and {fh=181, fm=16, fl=1}; (f) 12-step and {fh=181, fm=30, fl=1}; (g) cross-sectional comparison (line 170); (h) larger view of (g)
表 1 非标准三频外差TPU的最优频率组合
Table 1. Optimal frequency combinations for non-standard triple-frequency heterodyne TPU
fh1 fh2 fh3 3fh1+2fh2+2fh3 153 143 134 2fh1+2fh2+3fh3 160 149 139 表 2 非标准三频分层TPU的最优频率组合
Table 2. Optimal frequency combinations for non-standard triple-frequency hierarchical TPU
fh fm fl 3fh+2fm+2fl 169 13 1 2fh+2fm+3fl 181 16 1 表 3 基于3fh1+2fh2+2fh3外差TPU算法的定量比较
Table 3. Quantitative comparison based on 3fh1+2fh2+2fh3 heterodyne TPU
{fh1, fh2, fh3} Error rate/% RMSE/rad {153, 143, 134} 11.76 10.4789 {153, 148, 144} 30.41 11.3039 {153, 133, 114} 33.18 24.6249 表 4 基于2fh+2fm+3fl分层TPU的定量比较
Table 4. Quantitative comparison based on 2fh+2fm+3fl hierarchical TPU
{fh, fm, fl} Error rate/% RMSE/rad {181, 16, 1} 4.56 1.5398 {181, 6, 1} 15.41 3.9469 {181, 30, 1} 6.33 4.5043 -
[1] 吴荣, 赵世丽, 赵洋, 等. 条纹投影用于不同景深物体的三维测量[J]. 红外与金宝搏188软件怎么用 工程,2022,51(11):20220088. doi: 10.3788/IRLA20220088WU R, ZHAO SH L, ZHAO Y, et al. Fringe projection profilometry for 3D measurement of objects with different depth of fields[J]. Infrared and Laser Engineering, 2022, 51(11): 20220088. (in Chinese). doi: 10.3788/IRLA20220088 [2] ZUO CH, FENG SH J, HUANG L, et al. Phase shifting algorithms for fringe projection profilometry: a review[J]. Optics and Lasers in Engineering, 2018, 109: 23-59. doi: 10.1016/j.optlaseng.2018.04.019 [3] FENG SH J, ZUO CH, ZHANG L, et al. Calibration of fringe projection profilometry: a comparative review[J]. Optics and Lasers in Engineering, 2021, 143: 106622. doi: 10.1016/j.optlaseng.2021.106622 [4] 王永红, 张倩, 胡寅, 等. 显微条纹投影小视场三维表面成像技术综述[J]. 中国光学,2021,14(3):447-457. doi: 10.37188/CO.2020-0199WANG Y H, ZHANG Q, HU Y, et al. 3D small-field surface imaging based on microscopic fringe projection profilometry: a review[J]. Chinese Optics, 2021, 14(3): 447-457. (in Chinese). doi: 10.37188/CO.2020-0199 [5] GU Z M, FU J ZH, LIN H, et al. Development of 3D bioprinting: from printing methods to biomedical applications[J]. Asian Journal of Pharmaceutical Sciences, 2020, 15(5): 529-557. doi: 10.1016/j.ajps.2019.11.003 [6] HU Y, CHEN Q, FENG SH J, et al. Microscopic fringe projection profilometry: a review[J]. Optics and Lasers in Engineering, 2020, 135: 106192. doi: 10.1016/j.optlaseng.2020.106192 [7] GENG J. Structured-light 3D surface imaging: a tutorial[J]. Advances in Optics and Photonics, 2011, 3(2): 128-160. doi: 10.1364/AOP.3.000128 [8] VAN DER JEUGHT S, DIRCKX J J J. Real-time structured light profilometry: a review[J]. Optics and Lasers in Engineering, 2016, 87: 18-31. doi: 10.1016/j.optlaseng.2016.01.011 [9] 王霖, 韩旭, 伏燕军, 等. 用于三维测量的快速相位解包裹算法[J]. 应用光学,2019,40(2):271-277.WANG L, HAN X, FU Y J, et al. Fast phase unwrapping algorithm for 3D measurement[J]. Journal of Applied Optics, 2019, 40(2): 271-277. (in Chinese). [10] WANG L, YI L N, ZHANG Y T, et al. 3D reconstruction method based on N-step phase unwrapping[J]. The Visual Computer, 2024, 40(5): 3601-3613. doi: 10.1007/s00371-023-03054-y [11] SU X Y, CHEN W J. Reliability-guided phase unwrapping algorithm: a review[J]. Optics and Lasers in Engineering, 2004, 42(3): 245-261. doi: 10.1016/j.optlaseng.2003.11.002 [12] 杨泽霖, 张启灿, 衷涵. 利用双频外差和时空相位展开实现三维测量[J]. 光学与光电技术,2023,21(1):46-56.YANG Z L, ZHANG Q C, ZHONG H. Three dimensional shape measurement using dual-frequency heterodyne and spatial-temporal Phase Unwrapping[J]. Optics & Optoelectronic Technology, 2023, 21(1): 46-56. (in Chinese). [13] ZUO CH, HUANG L, ZHANG M L, et al. Temporal phase unwrapping algorithms for fringe projection profilometry: a comparative review[J]. Optics and Lasers in Engineering, 2016, 85: 84-103. doi: 10.1016/j.optlaseng.2016.04.022 [14] ZHANG S. Absolute phase retrieval methods for digital fringe projection profilometry: a review[J]. Optics and Lasers in Engineering, 2018, 107: 28-37. doi: 10.1016/j.optlaseng.2018.03.003 [15] WU H T, CAO Y P, AN H H, et al. A novel phase-shifting profilometry to realize temporal phase unwrapping simultaneously with the least fringe patterns[J]. Optics and Lasers in Engineering, 2022, 153: 107004. doi: 10.1016/j.optlaseng.2022.107004 [16] 张昂, 孙亚琴, 高楠, 等. 三灰度编码相位展开方法条纹投影轮廓术[J]. 光学 精密工程,2022,30(5):518-526. doi: 10.37188/OPE.20223005.0518ZHANG A, SUN Y Q, GAO N, et al. Fringe projection profilometry by ternary-gray encoded phase unwrapping method[J]. Optics and Precision Engineering, 2022, 30(5): 518-526. (in Chinese). doi: 10.37188/OPE.20223005.0518 [17] OCHOA N A. Fringe analysis with a two-step phase shifting method based on local Lissajous ellipse fitting[J]. Optics Communications, 2021, 483: 126647. doi: 10.1016/j.optcom.2020.126647 [18] 李乐阳, 吴周杰, 张启灿. 基于相移条纹分析的相位误差补偿技术发展综述(特邀)[J]. 金宝搏188软件怎么用 与光电子学进展,2024,61(2):0211008.LI Y Y, WU ZH J, ZHANG Q C. Phase error compensation technique based on phase-shifting fringe analysis: a review (Invited)[J]. Laser & Optoelectronics Progress, 2024, 61(2): 0211008. (in Chinese). [19] 王一, 王祎泽, 邾继贵. 光学相控器件调制误差对干涉投影条纹的影响[J]. 金宝搏188软件怎么用 与红外,2016,46(7):852-856.WANG Y, WANG Y Z, ZHU J G. Influence of modulation error of optical phase control device on interference projection fringe[J]. Laser & Infrared, 2016, 46(7): 852-856. (in Chinese). [20] LI J L, HASSEBROOK L G, GUAN CH. Optimized two-frequency phase-measuring-profilometry light-sensor temporal-noise sensitivity[J]. Journal of the Optical Society of America A, 2003, 20(1): 106-115. doi: 10.1364/JOSAA.20.000106 [21] 刘路, 潘艳娟, 奚冬冬, 等. 相位编码条纹投影轮廓术的相位展开误差校正方法[J]. 应用光学,2020,41(5):978-983. doi: 10.5768/JAO202041.0502007LIU L, PAN Y J, XI D D, et al. Phase unwrapping errors correction for phase-encoding based on fringe projection profilometry[J]. Journal of Applied Optics, 2020, 41(5): 978-983. (in Chinese). doi: 10.5768/JAO202041.0502007 [22] 侯艳丽, 梁瀚钢, 李付谦, 等. 相位测量轮廓术中时空结合的三频相位展开[J]. 光学学报,2022,42(1):0112006. doi: 10.3788/AOS202242.0112006HOU Y L, LIANG H G, LI F Q, et al. Spatial-temporal combined phase unwrapping in phase measurement profilometry[J]. Acta Optica Sinica, 2022, 42(1): 0112006. (in Chinese). doi: 10.3788/AOS202242.0112006 [23] CAI Z W, LIU X L, JIANG H, et al. Flexible phase error compensation based on Hilbert transform in phase shifting profilometry[J]. Optics Express, 2015, 23(19): 25171-25181. doi: 10.1364/OE.23.025171 [24] WANG J, WU ZH X, HUANG Y Y, et al. A rapid and accurate gamma compensation method based on double response curve fitting for high-quality fringe pattern generation[J]. Optics & Laser Technology, 2023, 160: 109084. [25] BING P, QIAN K M, LEI H, et al. Phase error analysis and compensation for nonsinusoidal waveforms in phase-shifting digital fringe projection profilometry[J]. Optics Letters, 2009, 34(4): 416-418. doi: 10.1364/OL.34.000416 [26] LIU K, WANG Y CH, LAU D L, et al. Gamma model and its analysis for phase measuring profilometry[J]. Journal of the Optical Society of America A, 2010, 27(3): 553-562. doi: 10.1364/JOSAA.27.000553 [27] ZHANG X, ZHU L M, LI Y F, et al. Generic nonsinusoidal fringe model and gamma calibration in phase measuring profilometry[J]. Journal of the Optical Society of America A, 2012, 29(6): 1047-1058. doi: 10.1364/JOSAA.29.001047 [28] ZUO CH, CHEN Q, GU G H, et al. Optimized three-step phase-shifting profilometry using the third harmonic injection[J]. Optica Applicata, 2013, 43(2): 393-408. [29] PETKOVIĆ T, PRIBANIĆ T, ZORAJA D. Selection of optimal frequencies in multiple-frequency fringe projection profilometry[J]. Optics and Lasers in Engineering, 2023, 163: 107455. doi: 10.1016/j.optlaseng.2022.107455 [30] ZHU J P, FENG X Y, ZHU CH H, et al. Optimal frequency selection for accuracy improvement in binary defocusing fringe projection profilometry[J]. Applied Optics, 2022, 61(23): 6897-6904. doi: 10.1364/AO.464506 [31] ZHANG M L, CHEN Q, TAO T Y, et al. Robust and efficient multi-frequency temporal phase unwrapping: optimal fringe frequency and pattern sequence selection[J]. Optics Express, 2017, 25(17): 20381-20400. doi: 10.1364/OE.25.020381 -




 下载:
下载: